Three vertices of a parallelogram ABCD taken in order are A(3, 6), B(5, 10) and C(3, 2) find :

(i) the coordinates of the fourth vertex D.

(ii) length of diagonal BD.

(iii) equation of side AB of the parallelogram ABCD.

The parallelogram ABCD is shown in the figure below:

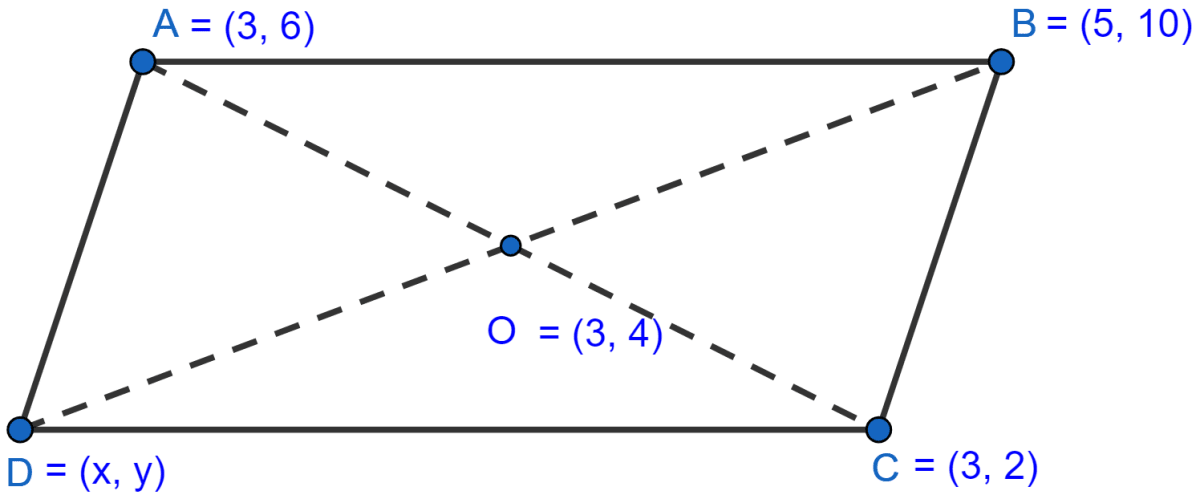
(i) We know that the diagonals of a parallelogram bisect each other. Let (x, y) be the coordinates of D.

Mid-point of diagonal AC = $\Big(\dfrac{3 + 3}{2}, \dfrac{6 + 2}{2}\Big)$ = (3, 4)

Mid-point of diagonal BD = $\Big(\dfrac{5 + x}{2}, \dfrac{10 + y}{2}\Big)$

These two should be same. On equating we get,

$\Rightarrow \dfrac{5 + x}{2} = 3 \text{ and } \dfrac{10 + y}{2} = 4 \\[1em] \Rightarrow 5 + x = 6 \text{ and } 10 + y = 8 \\[1em] \Rightarrow x = 6 - 5 \text{ and } y = 8 - 10 \\[1em] \Rightarrow x = 1 \text{ and } y = -2.$

Hence, coordinates of D are (1, -2).

(ii) By distance formula the distance between B(5, 10) and D(1, -2) is given by

$\sqrt{(x$2 - x1)^2 + (y2 - y1)^2}

Putting values we get BD,

$= \sqrt{(1 - 5)^2 + (-2 - 10)^2} \\[1em] = \sqrt{(-4)^2 + (-12)^2} \\[1em] = \sqrt{16 + 144} \\[1em] = \sqrt{160} = 4\sqrt{10}$

Hence, the length of diagonal BD is $4\sqrt{10}$ units.

(iii) Equation of side AB can be given by two point formula i.e.,

$y - y$1 = \dfrac{y2 - y1}{x2 - x1}(x - x1)

Putting values we get,

$\Rightarrow y - 6 = \dfrac{10 - 6}{5 - 3}(x - 3) \\[1em] \Rightarrow y - 6 = \dfrac{4}{2}(x - 3) \\[1em] \Rightarrow y - 6 = 2(x - 3) \\[1em] \Rightarrow y - 6 = 2x - 6 \\[1em] \Rightarrow 2x - y - 6 + 6 = 0 \\[1em] \Rightarrow 2x - y = 0.$