The line segment joining the points (3, -4) and (1, 2) is trisected at the points P and Q. If the coordinates of P and Q are (p, -2) and $\Big(\dfrac{5}{3}, q\Big)$ respectively, find the values of p and q.

Given P and Q trisect the points (3, -4) and (1, 2).

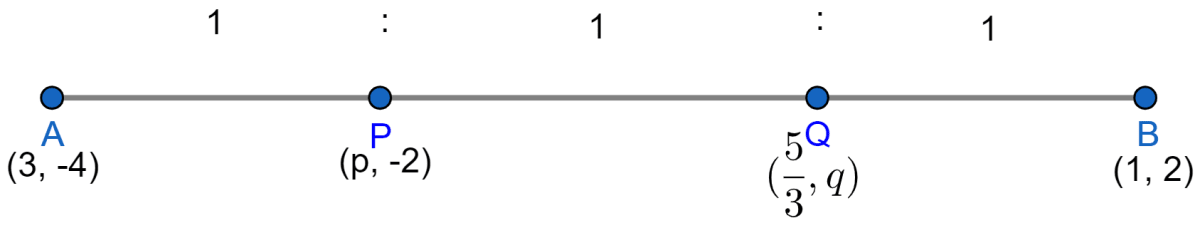
AP = PQ = QB ⇒ 2AP = PB

⇒ $\dfrac{AP}{PB} = \dfrac{1}{2}$ ⇒ P divides AB in the ratio 1 : 2, so coordinates of P are,

$\Rightarrow \Big(\dfrac{m$1x2 + m2x1}{m1 + m2}, \dfrac{m1y2 + m2y1}{m1 + m2}\Big) \\[1em] = \Big(\dfrac{1 \times 1 + 2 \times 3}{1 + 2}, \dfrac{1 \times 2 + 2 \times (-4)}{1 + 2}\Big) \\[1em] = \Big(\dfrac{1 + 6}{3}, \dfrac{2 - 8}{3}\Big) \\[1em] = \Big(\dfrac{7}{3}, -\dfrac{6}{3}\Big) \\[1em] = \Big(\dfrac{7}{3}, -2\Big).

Q divides AB in the ratio 2 : 1, so coordinates of Q are

$\Rightarrow \Big(\dfrac{m$1x2 + m2x1}{m1 + m2}, \dfrac{m1y2 + m2y1}{m1 + m2}\Big) \\[1em] = \Big(\dfrac{2 \times 1 + 1 \times 3}{2 + 1}, \dfrac{2 \times 2 + 1 \times (-4)}{2 + 1}\Big) \\[1em] = \Big(\dfrac{2 + 3}{3}, \dfrac{4 - 4}{3}\Big) \\[1em] = \Big(\dfrac{5}{3}, 0\Big) \\[1em] = \Big(\dfrac{5}{3}, 0\Big).

According to question,

Coordinates of P = (p, -2). Comparing it with $\Big(\dfrac{7}{3}, -2\Big)$ we get, p = $\dfrac{7}{3}.$

Coordinates of Q = $\Big(\dfrac{5}{3}, q\Big)$. Comparing it with $\Big(\dfrac{5}{3}, 0\Big)$ we get, q = 0.

Hence, the value of p = $\dfrac{7}{3}$ and q = 0.