Mathematics
The length of AC is :
m
m
m
m
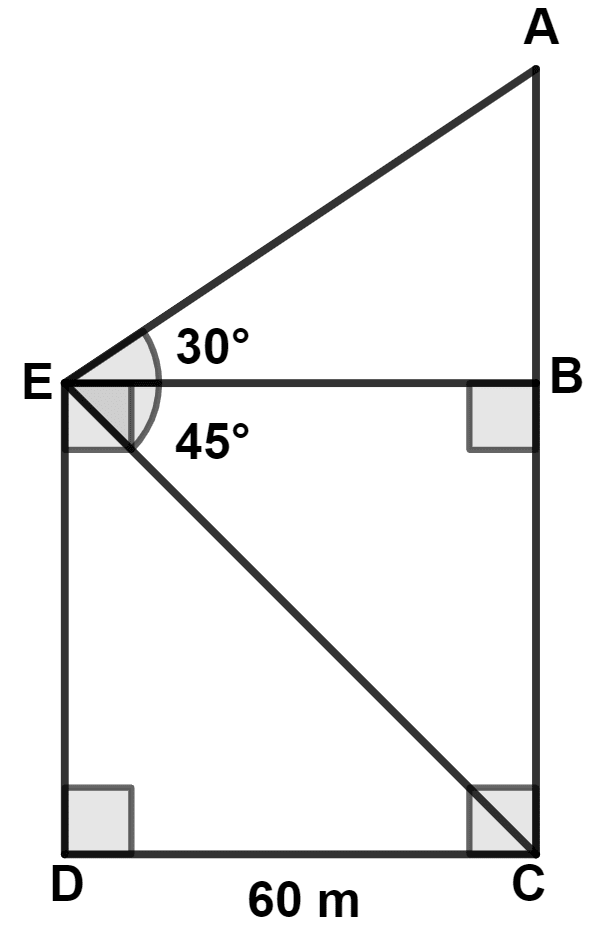
Heights & Distances
1 Like
Answer
In rectangle BCDE,
Opposite sides of rectangle are equal.
∴ BE = DC = 60 m
We know that,
tan θ =
From figure,
In △ ABE,
⇒ tan 30° =
⇒
⇒ AB = m.
In △ EBC,
⇒ tan 45° =
⇒
⇒ BC = 60 m.
From figure,
AC = AB + BC = m.
Hence, Option 3 is the correct option.
Answered By
2 Likes



