Mathematics
The diagonals of a quadrilateral ABCD intersect each other at the point O such that Show that ABCD is a trapezium.
Triangles
1 Like
Answer
Let ABCD be the quadrilateral where AC and BD intersect each other at O such that .
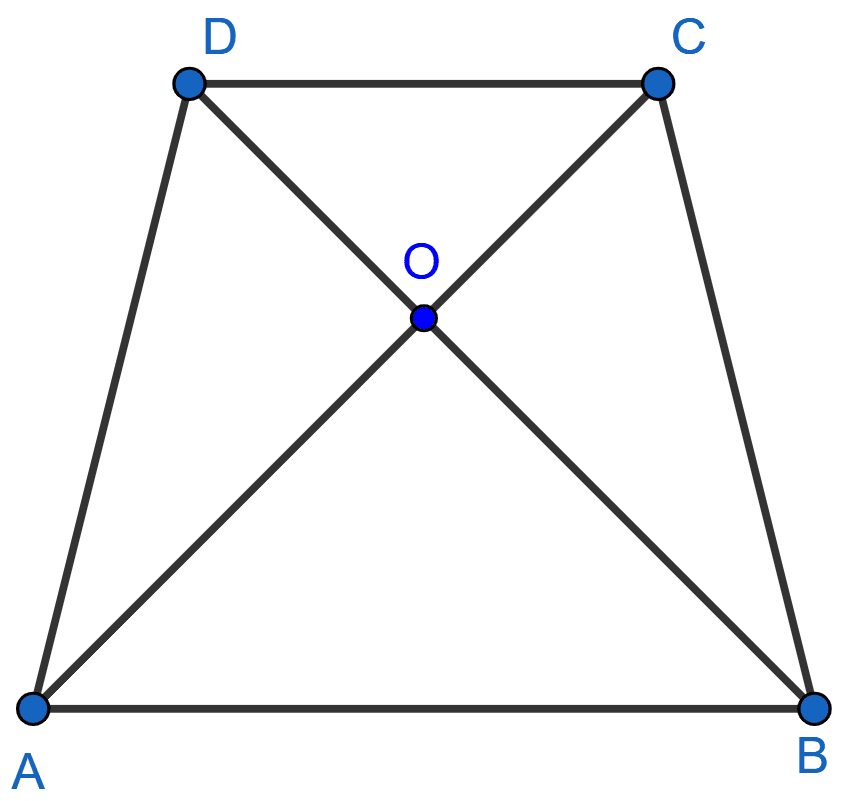
From point O,
Draw a line EO touching AD at E in such a way that, EO || AB.
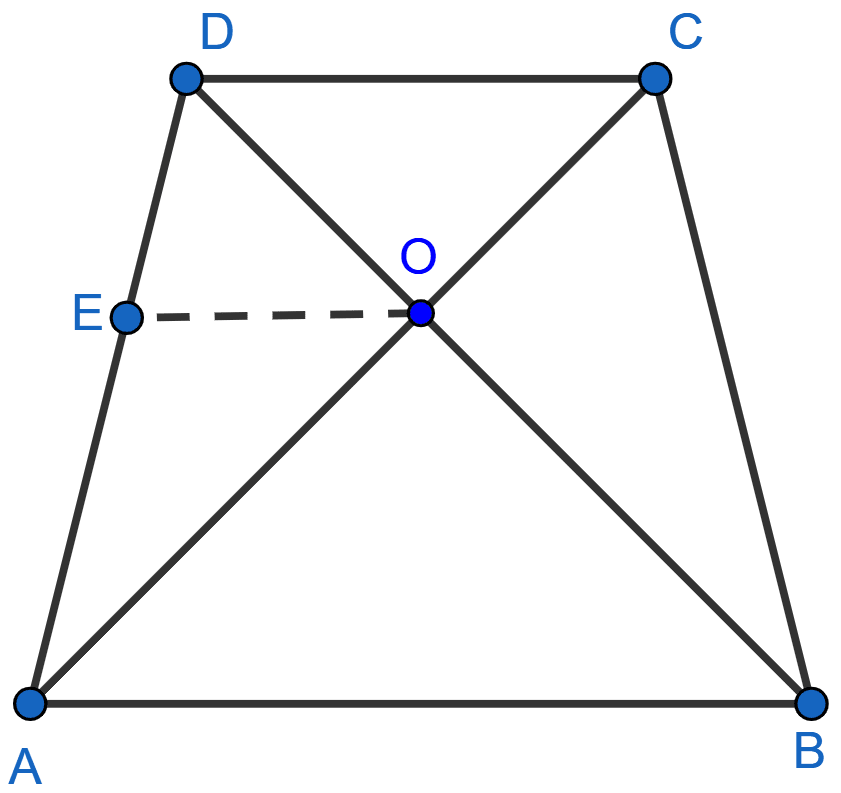
We know that,
If a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio.
In △ DAB,
EO || AB
……….(1)
Given,
From (1) and (2), we get :
We know that,
If a line divides any two sides of a triangle in the same ratio, then the line is parallel to the third side.
∴ EO || DC.
Since, EO || AB and EO || DC.
∴ AB || DC.
Hence, proved that ABCD is a trapezium.
Answered By
2 Likes
Related Questions
ABCD is a trapezium in which AB || DC and its diagonals intersect each other at the point O. Show that .
Using Theorem 6.2, prove that the line joining the mid-points of any two sides of a triangle is parallel to the third side.
Using Theorem 6.1, prove that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side.
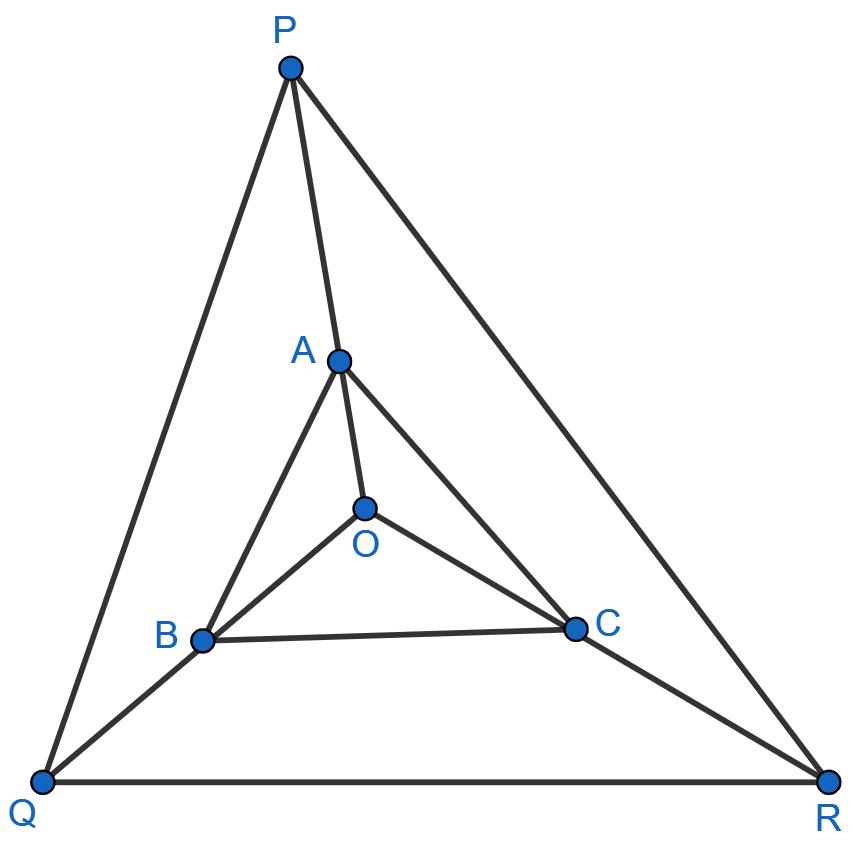
In figure, A, B and C are points on OP, OQ and OR respectively such that AB || PQ and AC || PR. Show that BC || QR.