Mathematics
The base of a right angled triangle is 12 cm and its hypotenuse is 13 cm long. Find its area and the perimeter.
Mensuration
36 Likes
Answer
It is given that,
ABC is a right angled triangle.
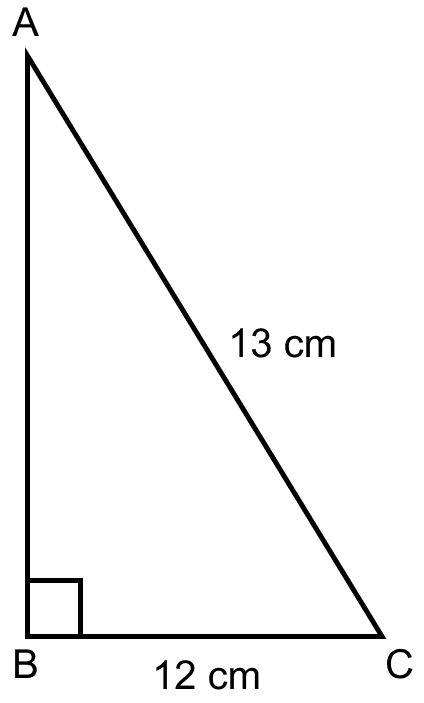
From figure,
BC = 12 cm and AC = 13 cm
Using the Pythagoras theorem,
AC2 = AB2 + BC2
Substituting the values we get,
⇒ 132 = AB2 + 122
⇒ AB2 = 132 – 122
⇒ AB2 = 169 – 144 = 25
⇒ AB = = 5 cm.
We know that,
Area of triangle ABC = × base × height.
Substituting the values we get,
A = = 30 cm2.
Perimeter of triangle ABC (P) = AB + BC + CA
Substituting the values we get,
= 5 + 12 + 13
= 30 cm.
Hence, area of triangle = 30 cm2 and perimeter = 30 cm.
Answered By
20 Likes
Related Questions
The sides of a triangular field are 975 m, 1050 m and 1125 m. If this field is sold at the rate of ₹ 1000 per hectare, find its selling price.
Find the area of a triangle whose sides are 34 cm, 20 cm and 42 cm. Hence, find the length of the altitude corresponding to the shortest side.
Find the area of an equilateral triangle whose side is 8 m. Give your answer correct to two decimal places.
If the area of an equilateral triangle is cm2, find its perimeter.