The angle of elevation of the top of a tower, from a point on the ground and at a distance of 150 m from its foot, is 30°. Find the height of the tower correct to one place of decimal.

Let MP be the tower of height h metres and O be the point on the ground 150 m away from the foot of the tower.

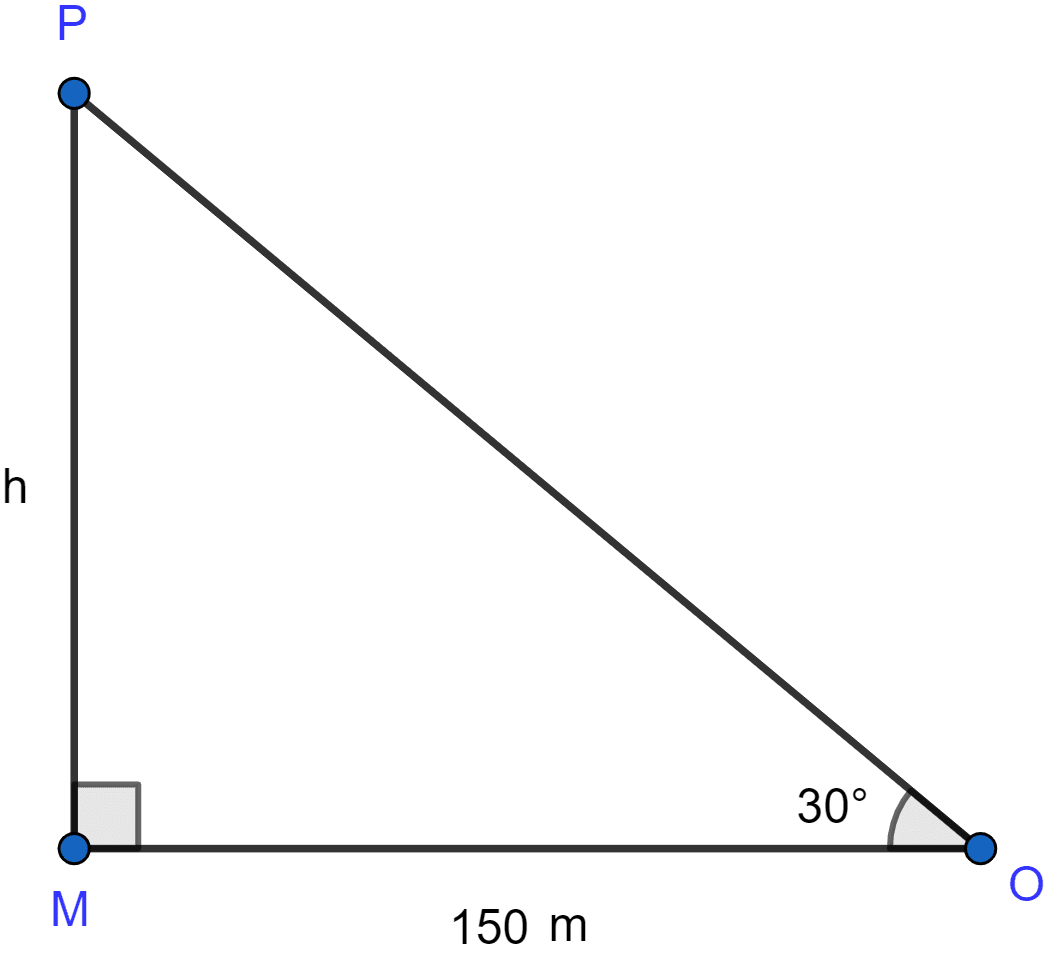
Then, the angle of elevation = ∠MOP = ∠30° (given).

In △OMP, ∠OMP = 90°.

From △OMP, we get

$\Rightarrow \text{tan 30°} = \dfrac{MP}{OM} \\[1em] \Rightarrow \dfrac{1}{\sqrt{3}} = \dfrac{h}{150} \\[1em] \Rightarrow h = \dfrac{150}{\sqrt{3}} \\[1em] \Rightarrow h = 86.6$

Hence, the height of the tower = 86.6 m.