The angle of elevation of the top of a tower as observed from a point on the ground is 'α' and on moving a metre towards the tower, the angle of elevation is 'β'.

Prove that the height of the tower is : $\dfrac{\text{a tan α tan β}}{\text{tan β - tan α}}$

Let CO be the tower of height h meters.

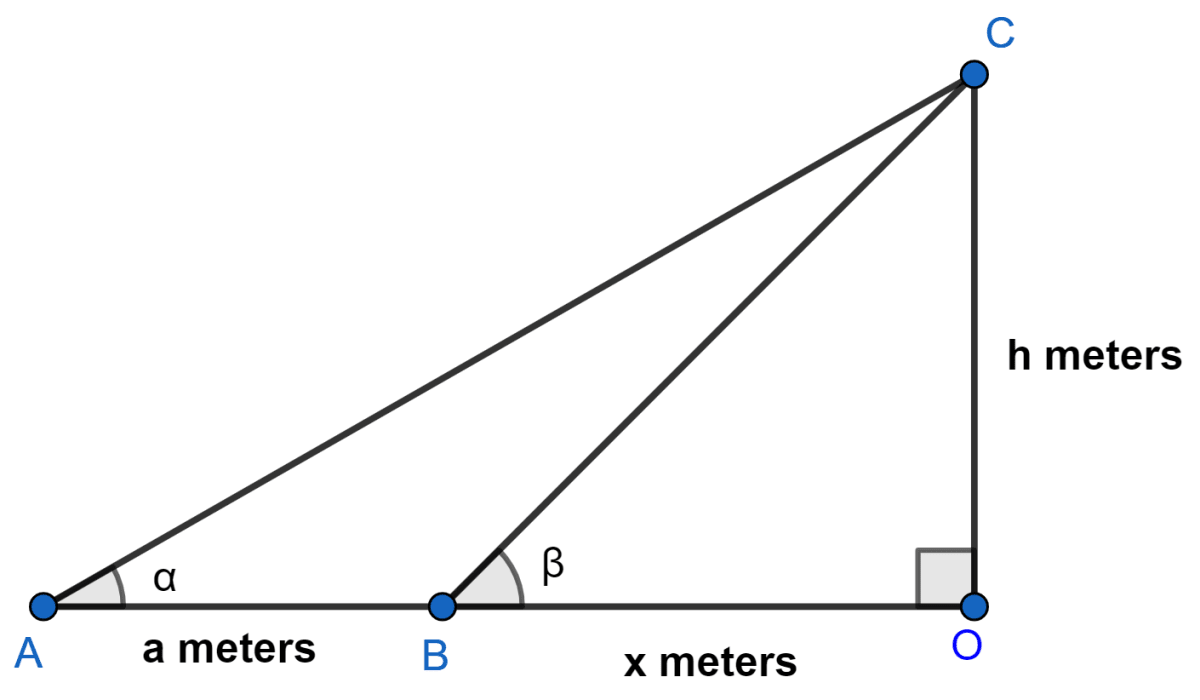
In △AOC,

⇒ tan α = $\dfrac{\text{Perpendicular}}{\text{Base}}$

⇒ tan α = $\dfrac{OC}{AO}$

⇒ tan α = $\dfrac{h}{a + x}$

⇒ (a + x)tan α = h

⇒ a tan α + x tan α = h

⇒ x tan α = h - a tan α

⇒ x = $\dfrac{\text{h - a tan α}}{\text{tan α}}$ ……..(1)

In △BOC,

⇒ tan β = $\dfrac{\text{Perpendicular}}{\text{Base}}$

⇒ tan β = $\dfrac{OC}{BO}$

⇒ tan β = $\dfrac{h}{x}$

⇒ x = $\dfrac{h}{\text{tan β}}$ …………(2)

From (1) and (2)

$\Rightarrow \dfrac{h}{\text{tan β}} = \dfrac{\text{h - a tan α}}{\text{tan α}} \\[1em] \Rightarrow \dfrac{h}{\text{tan β}} =\dfrac{h}{\text{tan α}} - \dfrac{\text{a tan α}}{\text{tan α}} \\[1em] \Rightarrow \dfrac{h}{\text{tan β}} - \dfrac{h}{\text{tan α}} = -a \\[1em] \Rightarrow \dfrac{\text{h tan α - h tan β}}{\text{tan α tan β}} = -a \\[1em] \Rightarrow \dfrac{\text{h (tan α - tan β)}}{\text{tan α tan β}} = -a \\[1em] \Rightarrow h = \dfrac{-\text{a tan α tan β}}{\text{(tan α - tan β)}} \\[1em] \Rightarrow h = \dfrac{\text{a tan α tan β}}{\text{(tan β - tan α)}}$

Hence, proved that $h = \dfrac{\text{a tan α tan β}}{\text{(tan β - tan α)}}$.