Solve the following pair of equations graphically. Plot atleast 3 points for each straight line.

2x - 7y = 6, 5x - 8y = -4.

Given,

Equation :

⇒ 2x - 7y = 6

⇒ 2x = 6 + 7y

⇒ x = $\dfrac{6 + 7y}{2}$ ……….(1)

When y = 0, x = $\dfrac{6 + 7 \times 0}{2} = \dfrac{6}{2}$ = 3,

y = -1, x = $\dfrac{6 + 7 \times -1}{2} = \dfrac{6 - 7}{2}$ = -0.5,

y = -2, x = $\dfrac{6 + 7 \times -2}{2} = \dfrac{6 - 14}{2} = -\dfrac{8}{2}$ = -4.

Table of values for equation (1)

Steps of construction :

1. Plot the points (3, 0), $(-\dfrac{1}{2}, -1)$ and (-4, -2) on graph paper.

2. Connect points by straight line.

Given,

Equation :

⇒ 5x - 8y = -4

⇒ 5x = 8y - 4

⇒ x = $\dfrac{8}{5}$y - $\dfrac{4}{5}$ …………..(2)

When, y = 0, x = $\dfrac{8}{5} \times 0 - \dfrac{4}{5} = 0 - \dfrac{4}{5} =$ -0.8,

y = 3, x = $\dfrac{8}{5} \times 3 - \dfrac{4}{5} = \dfrac{24}{5} - \dfrac{4}{5} = \dfrac{20}{5}$ = 4,

y = -2, x = $\dfrac{8}{5} \times -2 - \dfrac{4}{5} = -\dfrac{16}{5} - \dfrac{4}{5} = -\dfrac{20}{5}$ = -4.

Table of values for equation (2)

Steps of construction :

1. Plot the points (-0.8, 0), (4, 3) and (-4, -2) on graph paper.

2. Connect points by straight line.

The graphs of both the straight lines are shown in the figure.

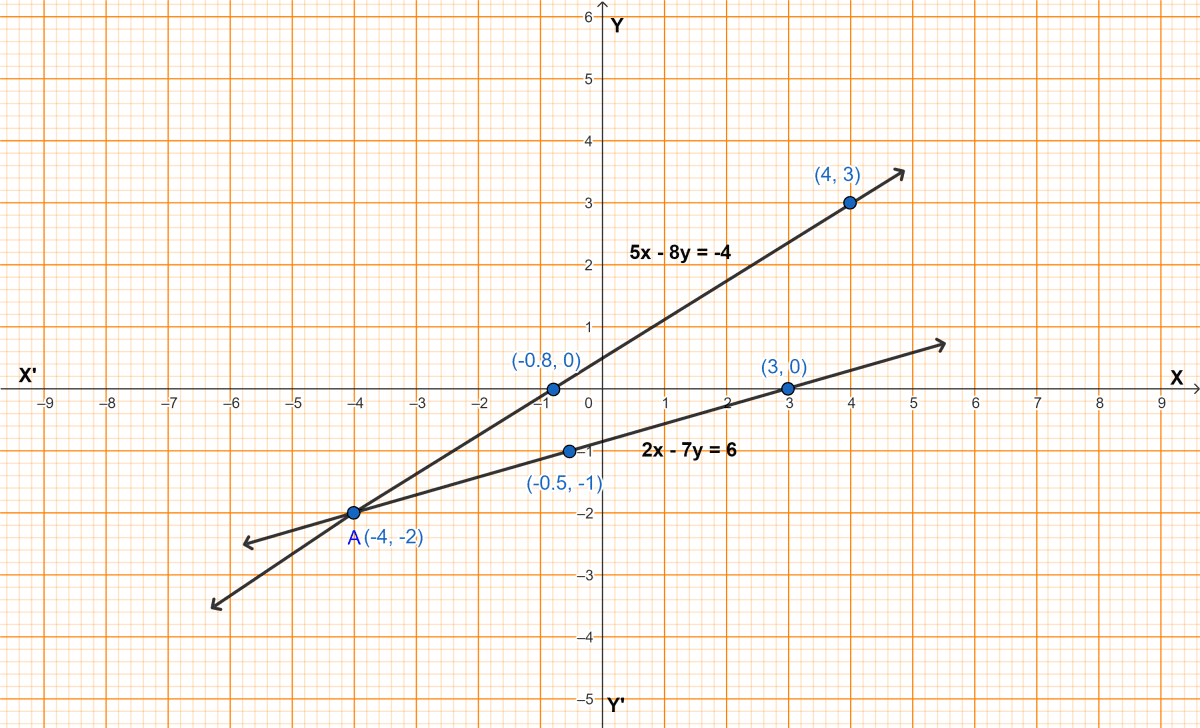
The lines intersect at point A(-4, -2).

Hence, the solution of the given equations is x = -4, y = -2.