Mathematics
Solve the following inequation and represent the solution set on a number line.
, x ∈ I
Linear Inequations
14 Likes
Answer
Given,
Solving L.H.S. of the inequation,
Solving R.H.S. of the inequation,
From (i) and (ii) we get,
-2 ≤ x < 2
Since, x ∈ I
∴ Solution set = {-2, -1, 0, 1}.
Solution on the number line is :
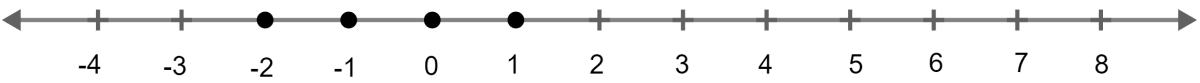
Answered By
9 Likes
Related Questions
Solve the inequation :
, x ∈ W.
Graph the solution set on the number line.
Find three consecutive largest positive integers such that the sum of one-third of first, one-fourth of second and one-fifth of third is at most 20.
Solve the following inequation and represent the solution set on the number line :
4x - 19 < , x ∈ R
Solve the following inequation and write the solution set :
13x - 5 < 15x + 4 < 7x + 12, x ∈ R
Represent the solution on a real number line.