KnowledgeBoat Logo

Mathematics

Solve and graph the solution set of :

(i) 2x - 9 < 7 and 3x + 9 ≤ 25; x ∈ R

(ii) 3x - 2 > 19 or 3 - 2x ≥ 7; x ∈ R

Linear Inequations

4 Likes

Answer

(i) Given,

⇒ 2x - 9 < 7

⇒ 2x < 7 + 9

⇒ 2x < 16

⇒ x < 162\dfrac{16}{2}

⇒ x < 8 ………..(1)

Also,

⇒ 3x + 9 ≤ 25

⇒ 3x ≤ 25 - 9

⇒ 3x ≤ 16

⇒ x ≤ 163\dfrac{16}{3}

⇒ x ≤ 5135\dfrac{1}{3}……….(2)

From (1) and (2), we get :

⇒ x ≤ 5135\dfrac{1}{3}

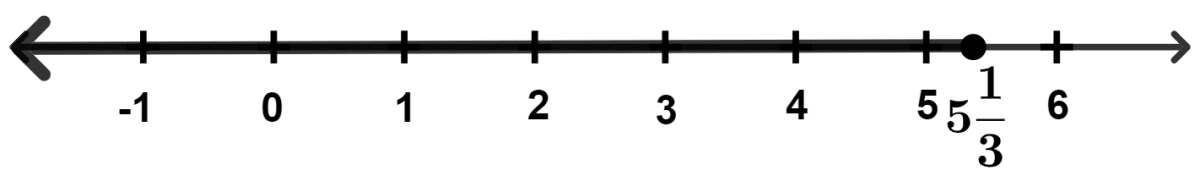
Solve and graph the solution set of : (i) 2x - 9 < 7 and 3x + 9 ≤ 25; x ∈ R (ii) 3x - 2 > 19 or 3 - 2x ≥ 7; x ∈ R. Mixed Practice, Concise Mathematics Solutions ICSE Class 10.

Hence, solution = {x : x ≤ 5135\dfrac{1}{3}, x ∈ R}.

(ii) Given,

⇒ 3x - 2 > 19

⇒ 3x > 19 + 2

⇒ 3x > 21

⇒ x > 213\dfrac{21}{3}

⇒ x > 7 ……..(1)

Also,

⇒ 3 - 2x ≥ 7

⇒ 2x ≤ 3 - 7

⇒ 2x ≤ -4

⇒ x ≤ −42-\dfrac{4}{2}

⇒ x ≤ -2 ……..(2)

From (1) and (2), we get :

x > 7 or x ≤ -2

Solve and graph the solution set of : (i) 2x - 9 < 7 and 3x + 9 ≤ 25; x ∈ R (ii) 3x - 2 > 19 or 3 - 2x ≥ 7; x ∈ R. Mixed Practice, Concise Mathematics Solutions ICSE Class 10.

Hence, solution = {x : x > 7 or x ≤ -2, x ∈ R}.

Answered By

1 Like

Related Questions