Mathematics
Prove the following:
(sin A + cos A)2 + (sin A - cos A)2 = 2
Trigonometrical Ratios
12 Likes
Answer
Solving L.H.S. of equation : (sin A + cos A)2 + (sin A - cos A)2 = 2, we get,
⇒ (sin A + cos A)2 + (sin A - cos A)2
⇒ sin2 A + cos2 A + 2 sin A cos A + sin2 A + cos2 A - 2 sin A cos A
Since, sin2 A + cos2 A = 1.
⇒ 1 + 2 sin A cos A + 1 - 2 sin A cos A
⇒ 2.
Since, L.H.S. = R.H.S.
Hence, proved that (sin A + cos A)2 + (sin A - cos A)2 = 2.
Answered By
9 Likes
Related Questions
Prove the following:
cot2 A - + 1 = 0
Prove the following:
= 1
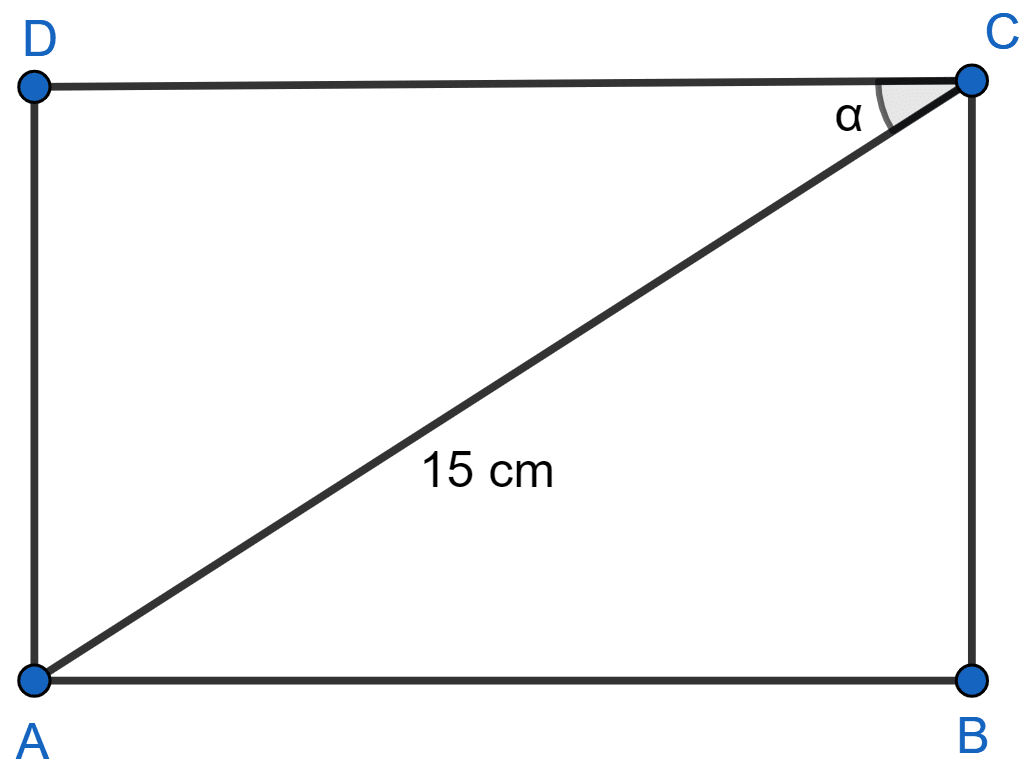
In the adjoining figure, ABCD is a rectangle. Its diagonal AC = 15 cm and ∠ACD = α. If cot α = , find the perimeter and the area of the rectangle.
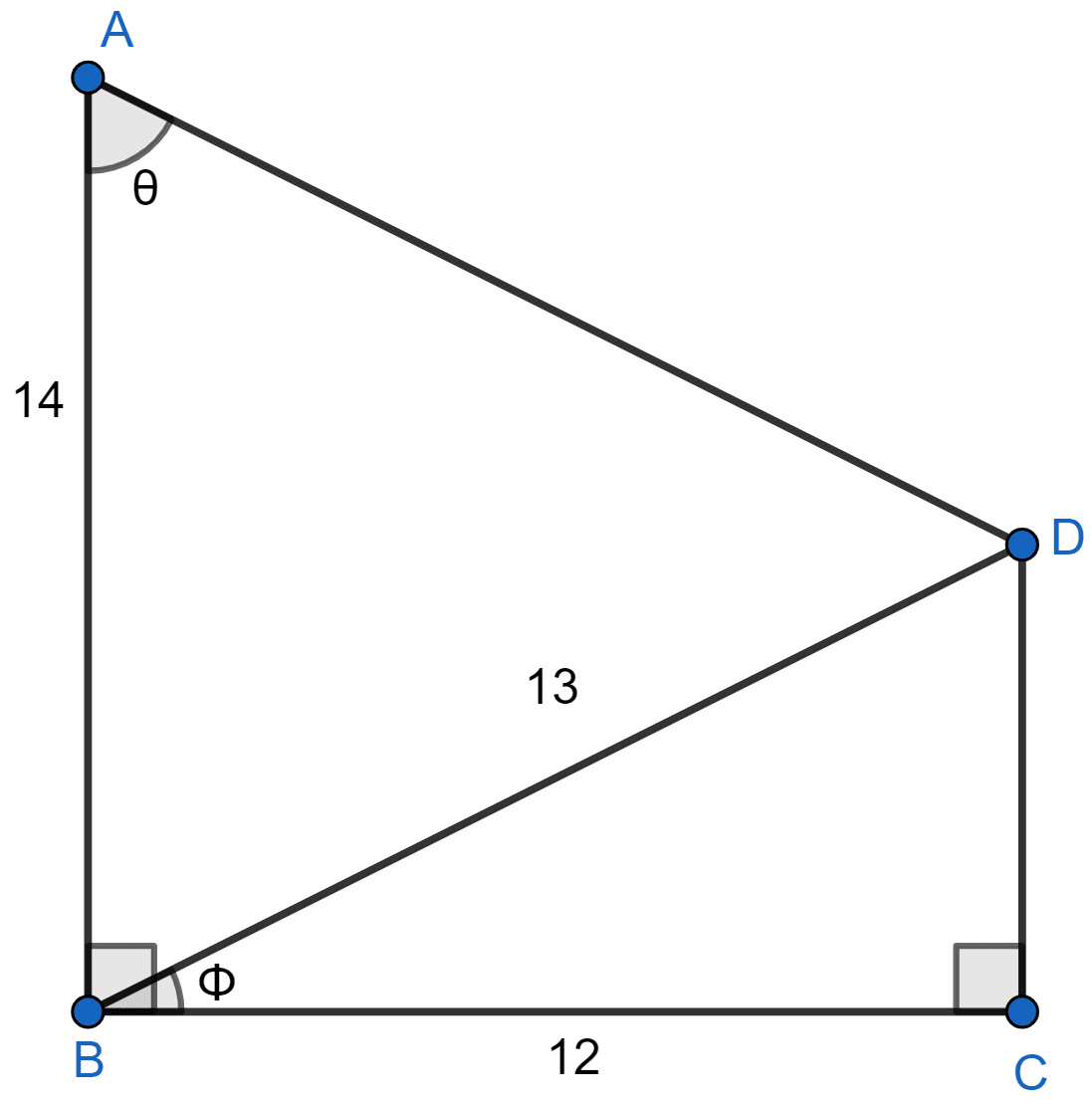
Using the measurements given in the figure alongside,
(a) Find the values of:
(i) sin Φ
(ii) tan θ.
(b) Write an expression for AD in terms of θ.