Mathematics
Prove that the points (3, 0), (6, 4) and (-1, 3) are the vertices of a right angled isosceles triangle.
Coordinate Geometry
1 Like
Answer
Let the points be A(3, 0), B(6, 4) and C(-1, 3).
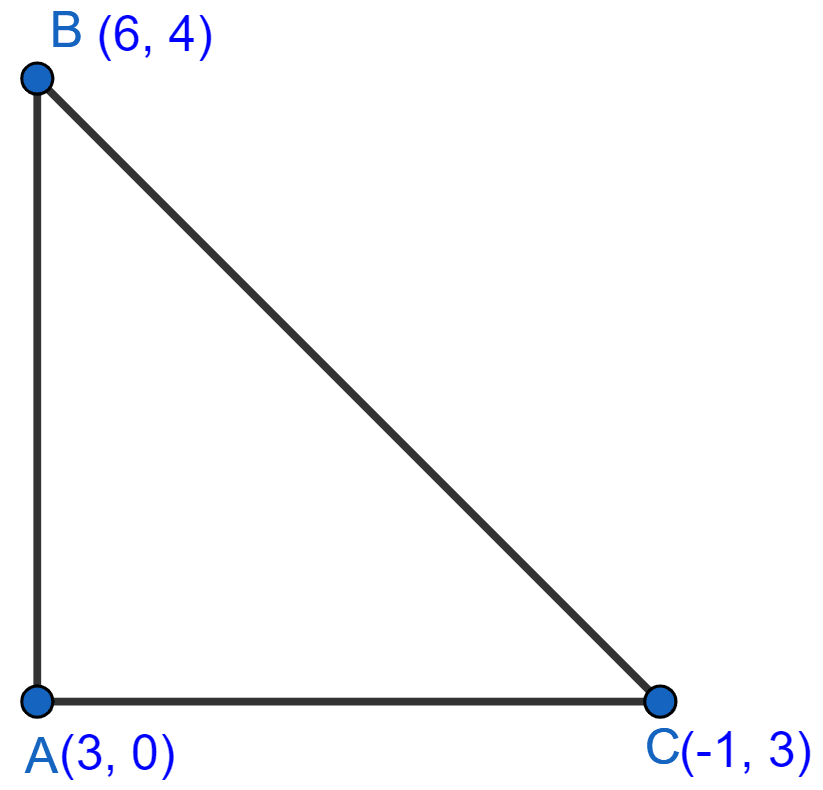
By distance formula,
∴ AB = AC = 5
∴ ΔABC is an isosceles triangle
AB2 + AC2 = 52 + 52
= 25 + 25
= 50.
BC2 = = 50.
Since, AB2 + AC2 = BC2.
Hence, proved that (3, 0), (6, 4) and (-1, 3) are the vertices of a right angled isosceles triangle.
Answered By
2 Likes
Related Questions
Show that the points (-3, 2), (-5, -5), (2, -3) and (4, 4), taken in order, are the vertices of rhombus. Also, find its area. Do the given points form a square?
A(-4, -1), B(-1, 2) and C(α, 5) are the vertices of an isosceles triangle. Find the value of α given that AB is the unequal side.
If A(-3, 2), B(α, β) and C(-1, 4) are the vertices of an isosceles triangle, prove that α + β = 1, given AB = BC.
Show that the points (2, 1), (0, 3), (-2, 1) and (0, -1), taken in order, are the vertices of a square. Also find the area of the square.