Prove that bisectors of any two opposite angles of a parallelogram are parallel.

Let the parallelogram be ABCD as shown in the figure below:

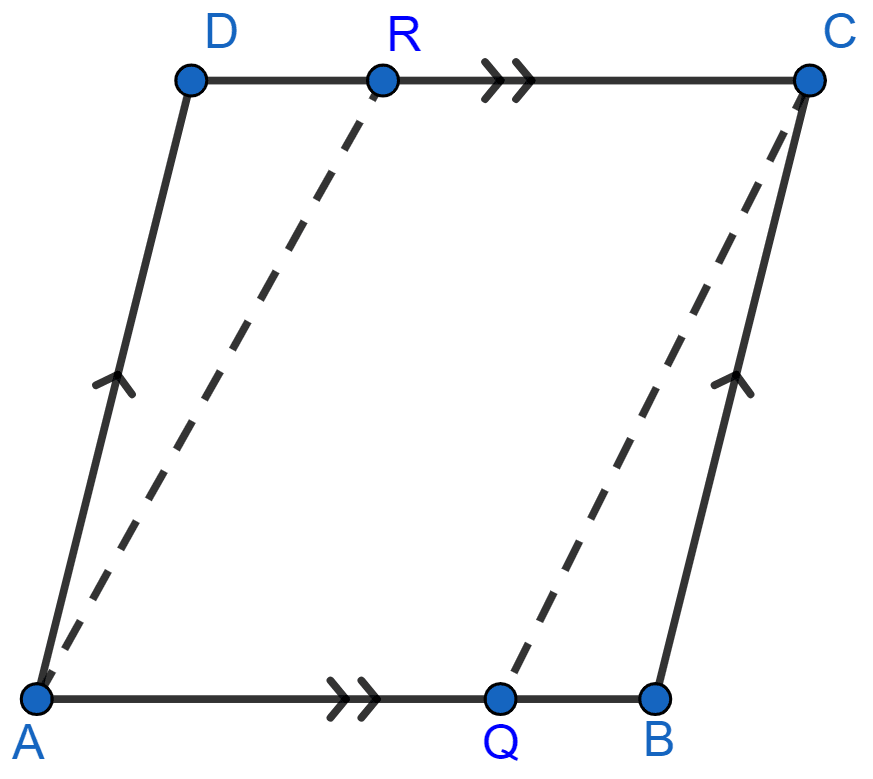
In parallelogram ABCD we have,

∠A = ∠C (Opposite angles are equal)

so,

$\dfrac{∠A}{2} = \dfrac{∠C}{2}$

∠DAR = ∠QCB (As AR bisects ∠A and QC bisects ∠C and ∠A = ∠C)

In △ADR and △CBQ,

⇒ ∠DAR = ∠QCB (Proved above)

⇒ AD = BC (Opposite sides of a || gm)

⇒ ∠D = ∠B (Opposite angles of a || gm)

Hence, △ADR ≅ △CBQ by ASA axiom.

∴ ∠DRA = ∠BQC (By C.P.C.T.) …….(i)

Also,

∠RAQ = ∠DRA (Alternate angles are equal) ………(ii)

From (i) and (ii) we get,

∠RAQ = ∠BQC (These are also corresponding angles)

Since, corresponding angles are equal, we can say that

AR || QC.

Hence, proved that bisectors of any two opposite angles of a parallelogram are parallel.