Make a diagram to show how hypermetropia is corrected. The near point of a hypermetropic eye is 1 m. What is the power of the lens required to correct this defect? Assume that the near point of the normal eye is 25 cm.

Hypermetropia can be corrected by using a convex lens as shown in the diagram below:

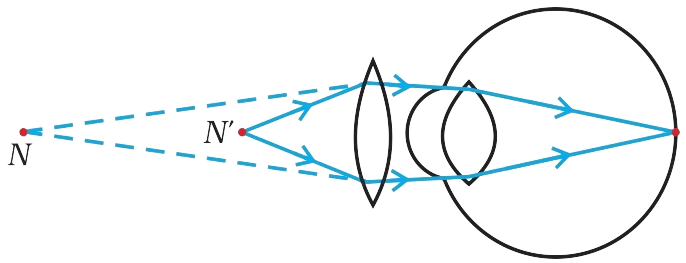
An object at 25 cm forms an image at the near point of hypermetropic eye.

Given,

Near point of hypermetropic eye = 1 m =100 cm

Object distance, u = -25 cm

Image distance, v = -100 cm

According to the formula,

$\phantom{\Rightarrow} \dfrac{1}{v} - \dfrac{1}{u} = \dfrac{1}{f} \\[1 em] \Rightarrow \dfrac{1}{-100} - \dfrac{1}{-25} = \dfrac{1}{f} \\[1 em] \Rightarrow \dfrac{-1 + 4}{100} = \dfrac{1}{f} \\[1 em] \Rightarrow \dfrac{3}{100} = \dfrac{1}{f} \\[1 em] \Rightarrow f = \dfrac{100}{3} \text{ cm} \\[1 em] \Rightarrow f = \dfrac{1}{3} \text{ m} \\[1 em] \text{Power} = \dfrac{1}{f} = \dfrac{1}{\dfrac{1}{3}} \\[1 em] \therefore \text{Power} = 3 \text{ D}$

Hence, the power of the lens required to correct this defect is 3 dioptre.