Mathematics
In triangle ABC, AD is perpendicular to side BC and AD2 = BD × DC.
Show that angle BAC = 90°.
Similarity
Answer
Triangle ABC is shown in the figure below:
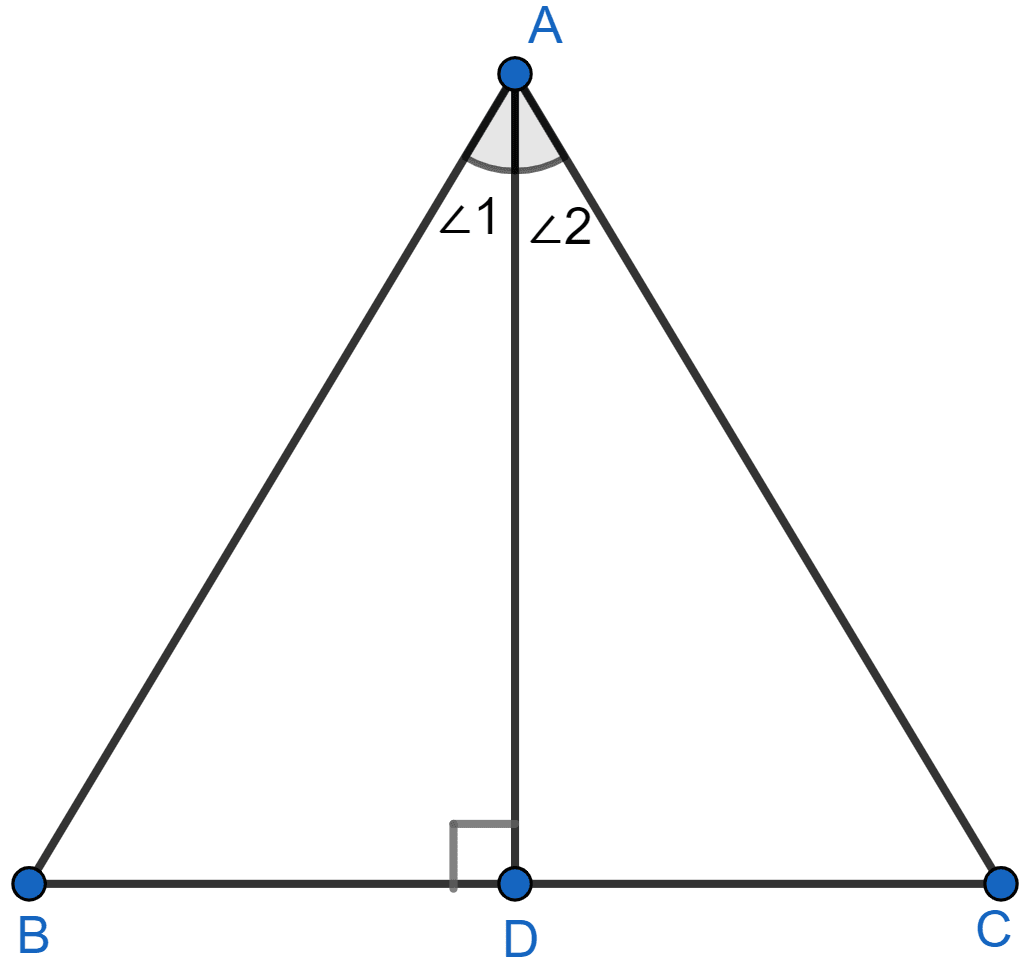
Given :
AD2 = BD × DC
⇒
∠ADB = ∠ADC [Both = 90°]
∴ △DBA ~ △DAC (By SAS).
Since, triangles are similar they will be equiangular.
∴ ∠1 = ∠C and ∠2 = ∠B
⇒ ∠1 + ∠2 = ∠B + ∠C
⇒ ∠A = ∠B + ∠C
By angle sum property :
⇒ ∠A + ∠B + ∠C = 180°
⇒ ∠A + ∠A = 180°
⇒ 2∠A = 180°
⇒ ∠A = 90°.
From figure,
⇒ ∠BAC = ∠A = 90°.
Hence, proved that ∠BAC = 90°.
Answered By
Related Questions
In △ABC, ∠B = 90° and BD ⊥ AC.
(i) If CD = 10 cm and BD = 8 cm; find AD.
(ii) If AC = 18 cm and AD = 6 cm; find BD.
(iii) If AC = 9 cm and AB = 7 cm; find AD.
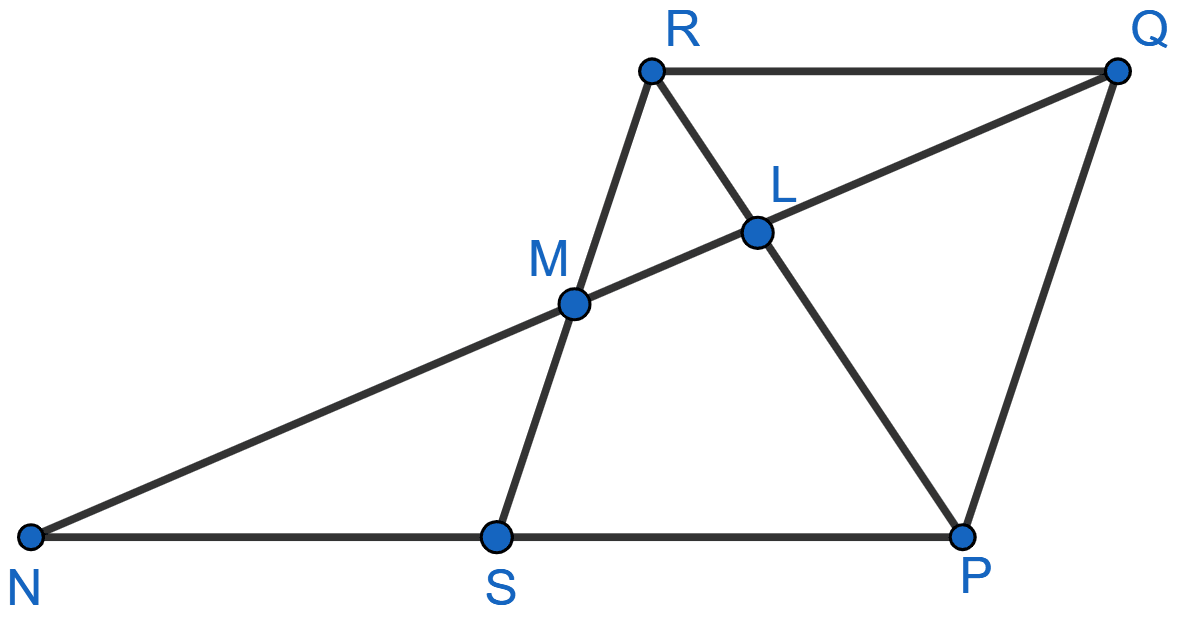
In the figure, PQRS is a parallelogram with PQ = 16 cm and QR = 10 cm, L is a point on PR such that RL : LP = 2 : 3. QL produced meets RS at M and PS produced at N. Find the lengths of PN and RM.
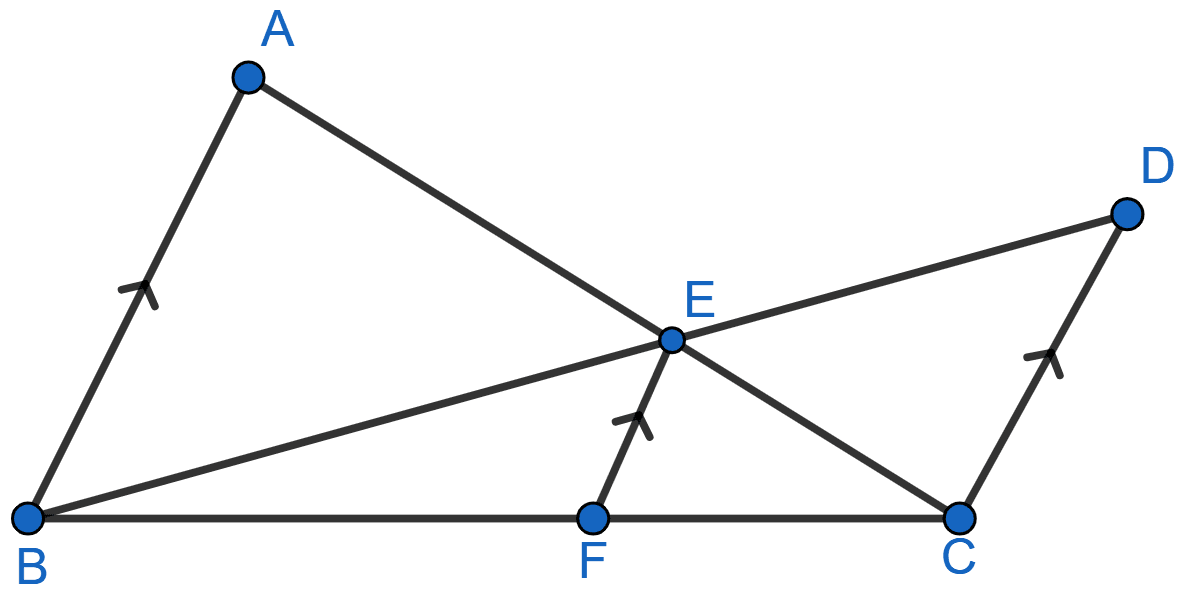
In the given figure, AB || EF || DC; AB = 67.5 cm, DC = 40.5 cm and AE = 52.5 cm.
(i) Name the three pairs of similar triangles.
(ii) Find the length of EC and EF.
In the given figure, P is a point on AB such that AP : PB = 4 : 3. PQ is parallel to AC.
(i) Calculate the ratio PQ : AC, giving reason for your answer.
(ii) In triangle ARC, ∠ARC = 90° and in triangle PQS, ∠PSQ = 90°. Given QS = 6 cm, calculate the length of AR.
