Physics
In the network shown in figure, calculate the equivalent resistance between the points (a) A and B (b) A and C
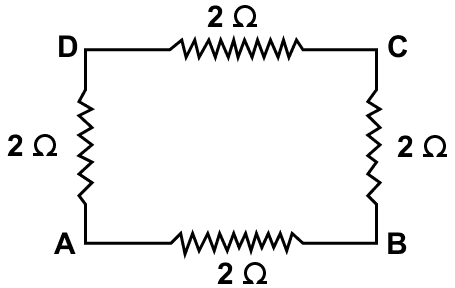
Current Electricity
51 Likes
Answer
In the circuit, there are two parts. In the first part, three resistors of 2 Ω each are connected in series. If the equivalent resistance of this part is R's then
R's = (2 + 2 + 2) Ω = 6 Ω
In the second part, the resistance of first part (R's = 6 Ω ) and 2 Ω are connected in parallel. If the equivalent resistance of this part is Rp then
p} = \dfrac{1}{6} + \dfrac{1}{2} \\[0.5em] \dfrac{1}{Rp} = \dfrac{1 + 3}{6} \\[0.5em] \dfrac{1}{Rp} = \dfrac{4}{6} \\[0.5em] \Rightarrow Rp = 1.5 Ω \\[0.5em]
∴ Rp = 1.5 Ω
(b) In the circuit, there are three parts. In the first part, two resistors of 2 Ω each are connected in series. If the equivalent resistance of this part is R's then
R's = (2 + 2) Ω = 4 Ω
In the second part, two resistors of 2 Ω each are connected in series. If the equivalent resistance of this part is R''s then
R''s = (2 + 2) Ω = 4 Ω
In the third part, the two parts of resistance R's = 4 Ω and R''s = 4 Ω are connected in parallel. If the equivalent resistance between points A and C is Rp then
p} = \dfrac{1}{4} + \dfrac{1}{4} \\[0.5em] \dfrac{1}{Rp} = \dfrac{2}{4} \\[0.5em] \dfrac{1}{Rp} = \dfrac{1}{2} \\[0.5em] \Rightarrow Rp = 2 Ω \\[0.5em]
∴ Equivalent Resistance between A and C = 2 Ω
Answered By
28 Likes
Related Questions
Calculate the effective resistance between the points A and B in the network shown below in figure.

Calculate the equivalent resistance between the points A and B in figure.

Five resistors, each of 3 ohm, are connected as shown in figure. Calculate the resistance (a) between the points P and Q, and (b) between the points X and Y.

Two resistors of 4.0 Ω and 6.0 Ω are connected (a) in series, (b) in parallel, with a battery of 6.0 V and negligible internal resistance. For each case draw a circuit diagram and calculate the current through the battery.