Mathematics
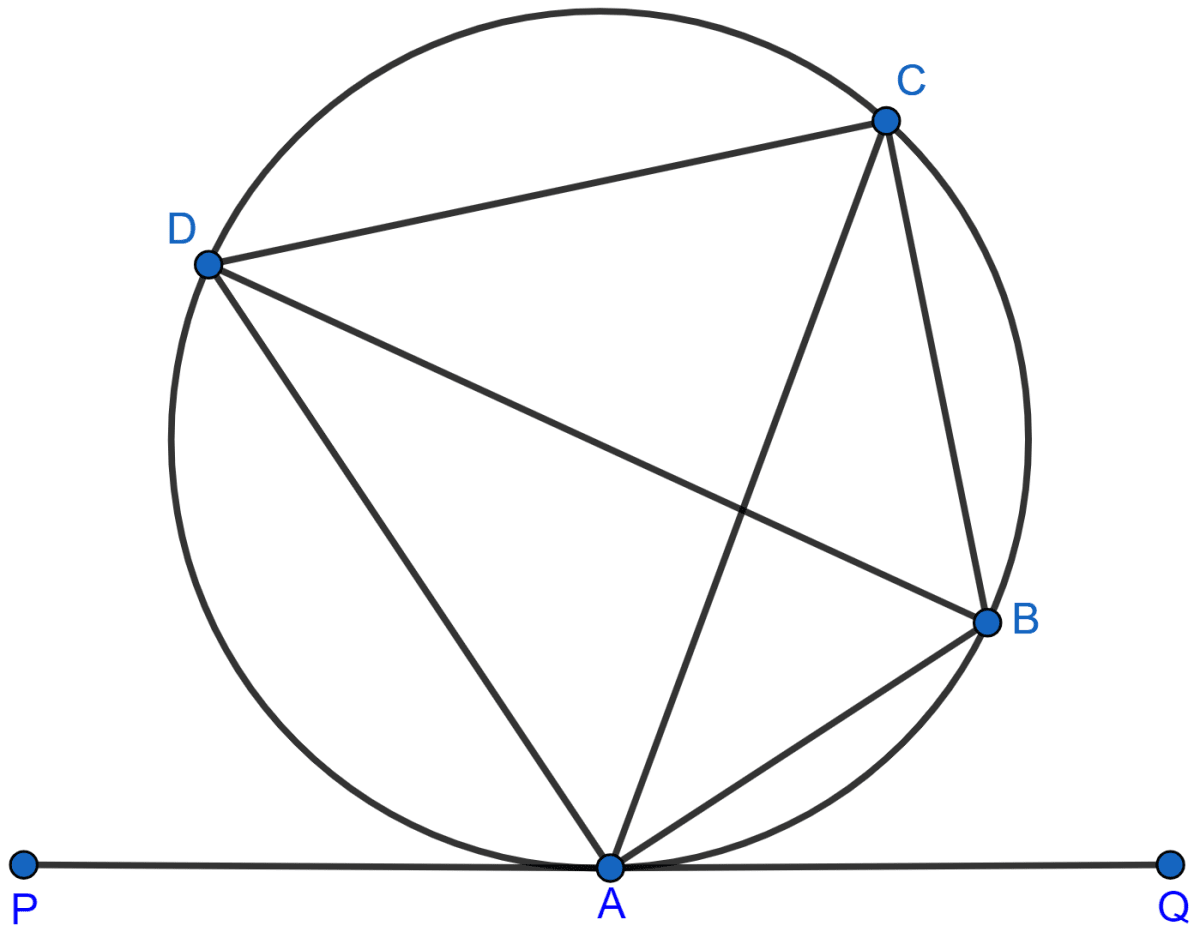
In the given figure, PQ is a tangent to the circle at A. AB and AD are bisectors of ∠CAQ and ∠PAC. If ∠BAQ = 30°, prove that : BD is diameter of the circle.
Circles
13 Likes
Answer
From figure,
∠CAB = ∠BAQ = 30° (AB is angle bisector of ∠CAQ)
⇒ ∠CAQ = 2∠BAQ = 60°.
From figure,
⇒ ∠CAQ + ∠PAC = 180° [Linear pair]
⇒ 60° + ∠PAC = 180°
⇒ ∠PAC = 180° - 60°
⇒ ∠PAC = 120°.
⇒ ∠PAC = 2∠CAD (AD is angle bisector of ∠PAC)
⇒ 120° = 2∠CAD
⇒ ∠CAD =
⇒ ∠CAD = 60°.
From figure,
∠DAB = ∠CAD + ∠CAB = 60° + 30° = 90°.
Thus BD, subtends 90° on the circle. Since, angle in semi-circle is a right angle.
Hence, BD is the diameter of the circle.
Answered By
8 Likes
Related Questions
In the given figure, AB is the diameter of the circle, with center O, and AT is the tangent. Calculate the numerical value of x.
In the given figure, O is the center of the circumcircle ABC. Tangents A and C intersect at P. Given angle AOB = 140° and angle APC = 80°; find the angle BAC.
In the given figure, PT touches the circle with center O at point R. Diameter SQ is produced to meet the tangent TR at P.
Given ∠SPR = x° and ∠QRP = y°;
prove that :
(i) ∠ORS = y°
(ii) write an expression connecting x and y.
PT is a tangent to the circle at T. If ∠ABC = 70° and ∠ACB = 50°; calculate :
(i) ∠CBT
(ii) ∠BAT
(iii) ∠APT
