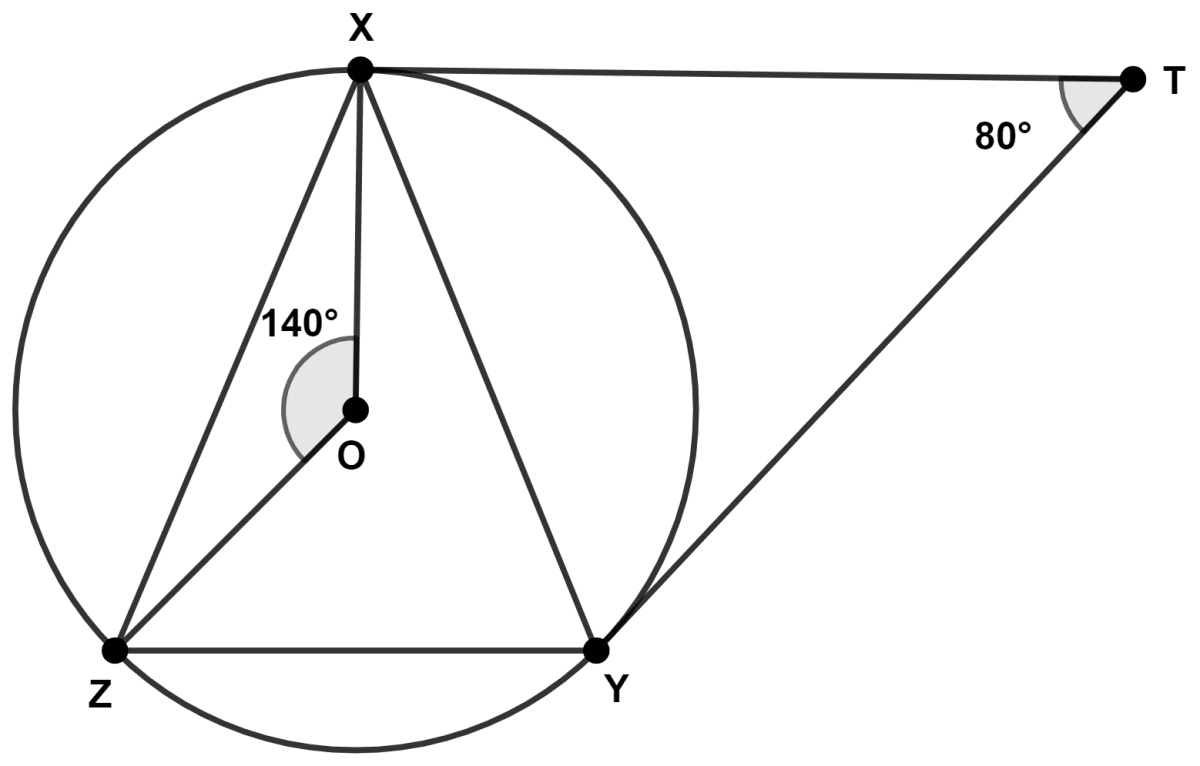
In the given figure, O is the center of the circumcircle of triangle XYZ. Tangents at points X and Y intersect at point T. If angle XTY = 80° and angle XOZ = 140°, find the angle ZXY.

Join OY.

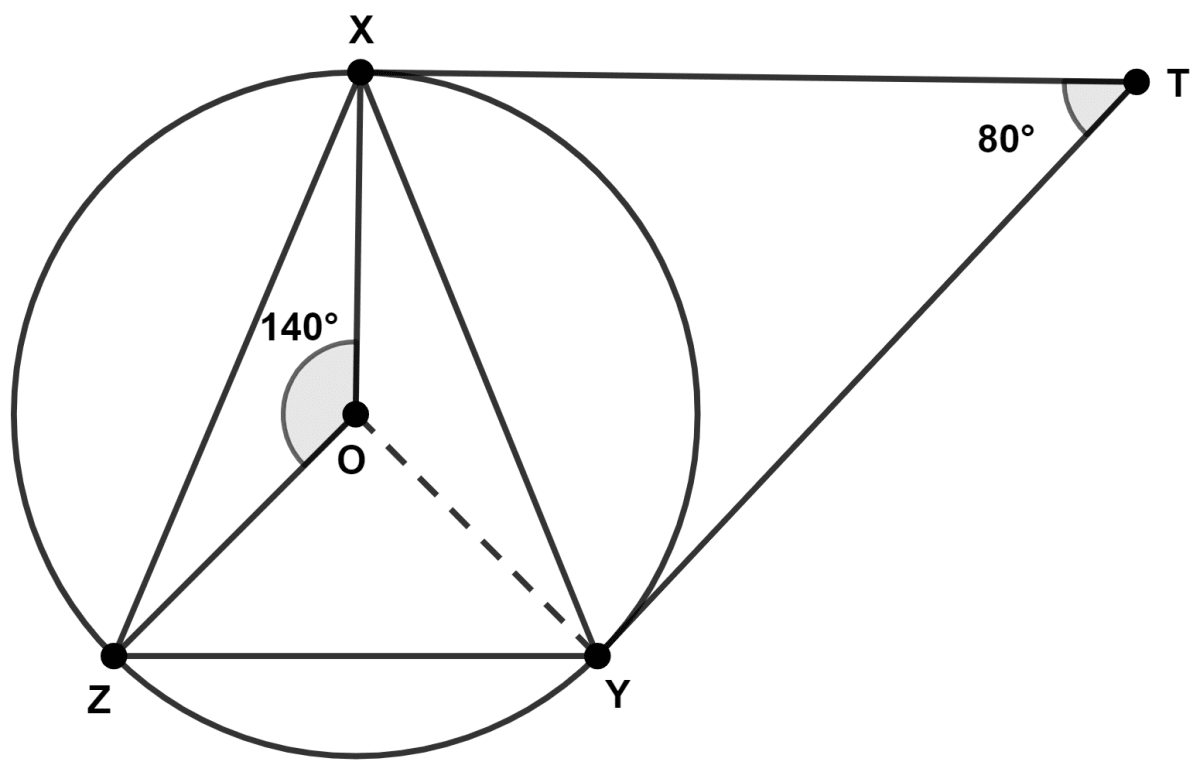
Since a tangent at any point of a circle is perpendicular to the radius at the point of contact, we have

∠OXT = ∠OYT = 90°.

Since sum of angles of a quadrilateral is 360°.

In XOYT,

⇒ ∠OXT + ∠OYT + ∠XOY + ∠XTY = 360°

⇒ 90° + 90° + ∠XOY + 80° = 360°

⇒ ∠XOY = 360° - 90° - 90° - 80° = 100°.

From figure,

⇒ ∠XOY + ∠XOZ + ∠ZOY = 360°

⇒ 100° + 140° + ∠ZOY = 360°

⇒ ∠ZOY = 360° - 100° - 140° = 120°.

We know that,

Angle subtended by an arc on the center of circle is twice the angle subtended by it on any part of the circumference.

∴ 2∠ZXY = ∠ZOY

⇒ ∠ZXY = $\dfrac{∠ZOY}{2} = \dfrac{120}{2}$ = 60°.

Hence, ∠ZXY = 60°.