KnowledgeBoat Logo

Mathematics

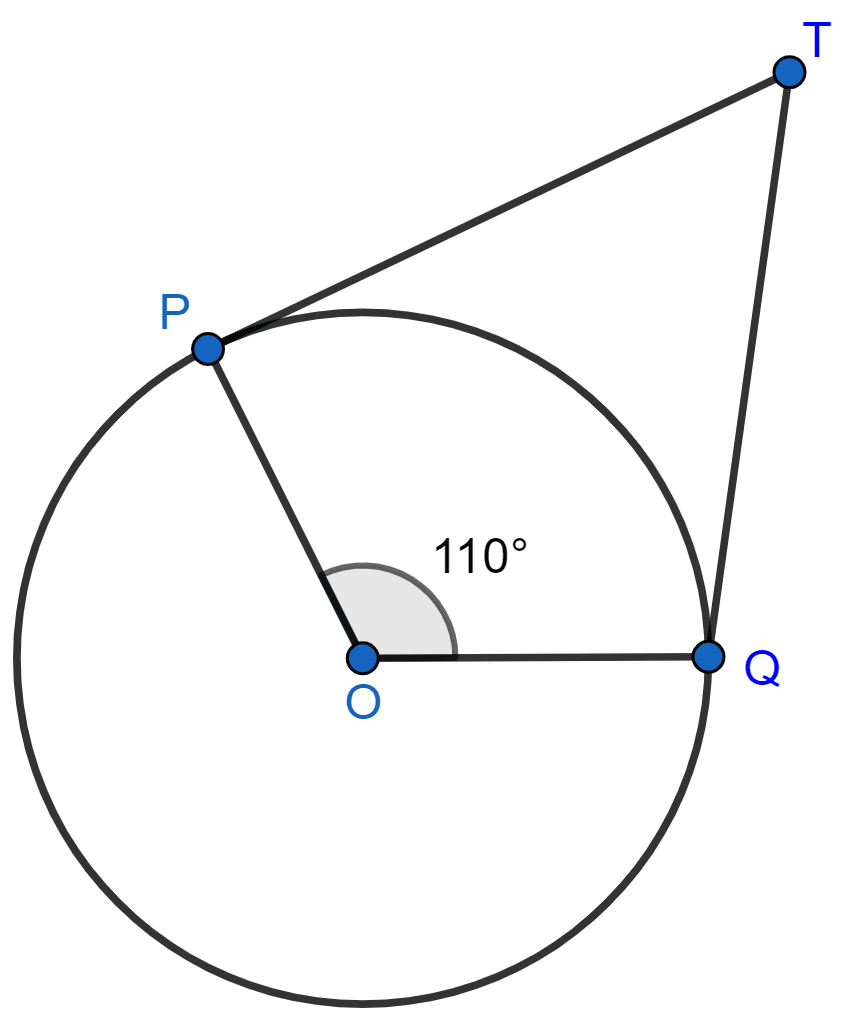
In the given figure, if TP and TQ are the two tangents to a circle with centre O so that ∠POQ = 110°, then ∠PTQ is equal to

  1. 60°

  2. 70°

  3. 80°

  4. 90°

In the given figure, if TP and TQ are the two tangents to a circle with centre O so that ∠POQ = 110°, then ∠PTQ is equal to 1. 60° 2. 70° 3. 80° 4. 90°. NCERT Class 10 Mathematics CBSE Solutions.

Circles

1 Like

Answer

We know that,

The tangent at any point of a circle is perpendicular to the radius through the point of contact.

So,

OP ⊥ PT and OQ ⊥ QT

∠OPT = 90° and ∠OQT = 90°

In quadrilateral OPTQ,

⇒ ∠OPT + ∠PTQ + ∠OQT + ∠POQ = 360°

⇒ 90° + ∠PTQ + 90° + 110° = 360°

⇒ ∠PTQ + 290° = 360°

⇒ ∠PTQ = 360° - 290° = 70°.

Hence, Option 2 is the correct option.

Answered By

1 Like

Related Questions