Mathematics
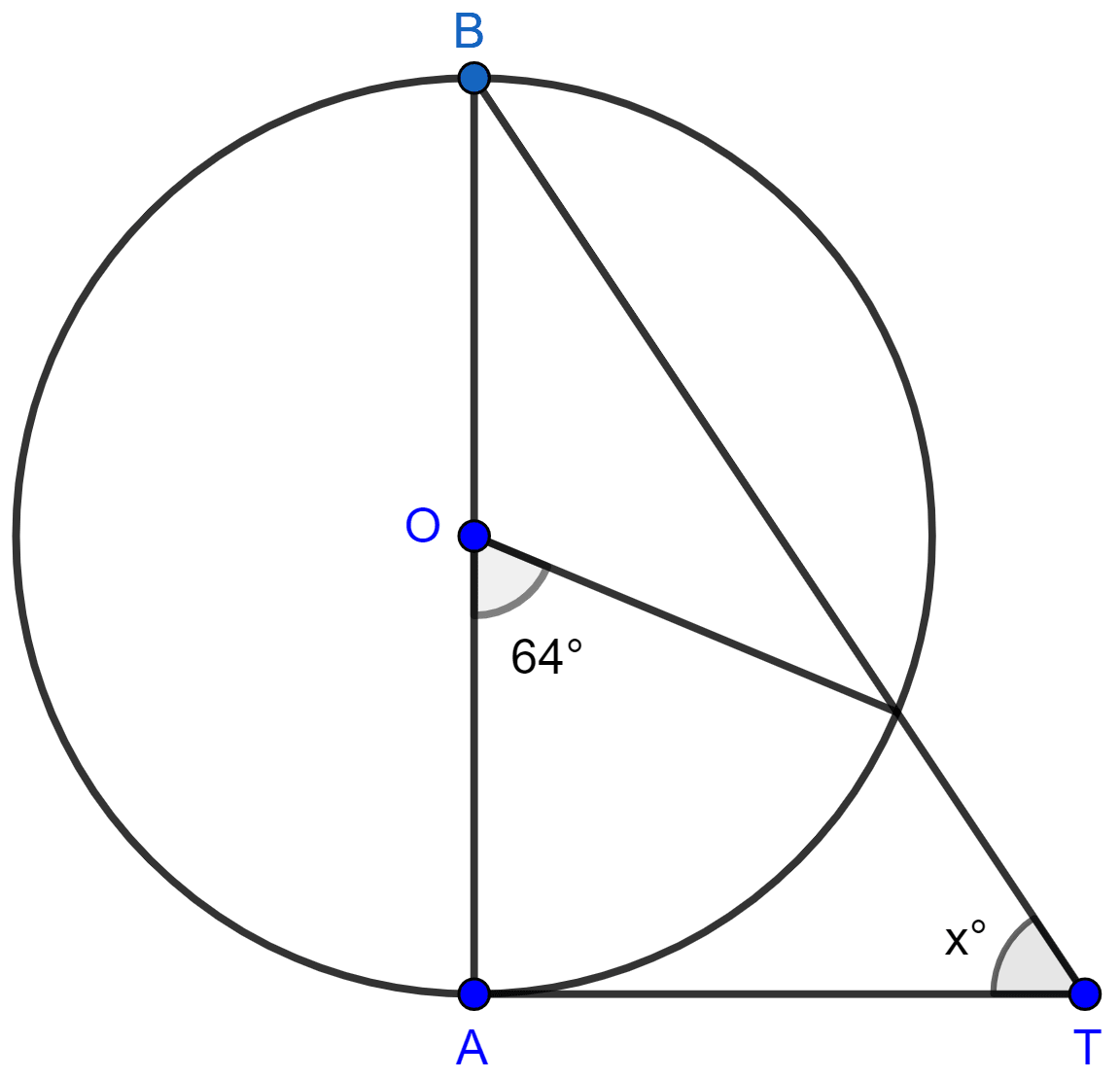
In the given figure, AB is the diameter of the circle, with center O, and AT is the tangent. Calculate the numerical value of x.
Answer
In △OBC,
OB = OC (Radius of same circle)
As, angles opposite to equal sides are equal.
∴ ∠OBC = ∠OCB
As, exterior angle is equal to the sum of two opposite interior angles.
∴ ∠COA = ∠OBC + ∠OCB
⇒ ∠COA = 2∠OBC
⇒ 2∠OBC = 64°
⇒ ∠OBC = 32°.
In △ABT,
∠BAT = 90° (∵ Tangent at any point of a circle and the radius through this point are perpendicular to each other.)
⇒ ∠BAT + ∠ABT + ∠ATB = 180° (By angle sum property of triangle)
⇒ 90° + 32° + x° = 180° [∵ ∠ABT and ∠OBC is the same angle]
⇒ x° + 122° = 180°
⇒ x° = 180° - 122°
⇒ x° = 58°.
Hence, x = 58°.
Related Questions
In a triangle ABC, the incircle (center O) touches BC, CA and AB at points P, Q and R respectively. Calculate :
(i) ∠QOR
(ii) ∠QPR;
given that ∠A = 60°.
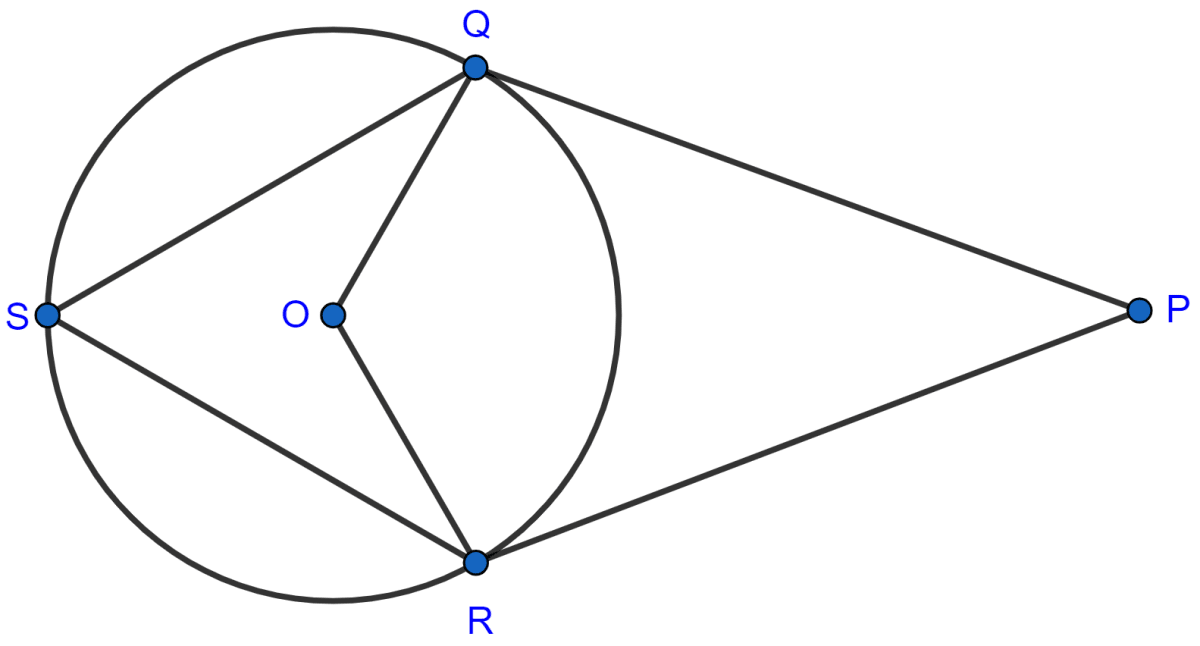
In the following figure, PQ and PR are tangents to the circle, with center O. If ∠QPR = 60°, calculate :
(i) ∠QOR,
(ii) ∠OQR,
(iii) ∠QSR.
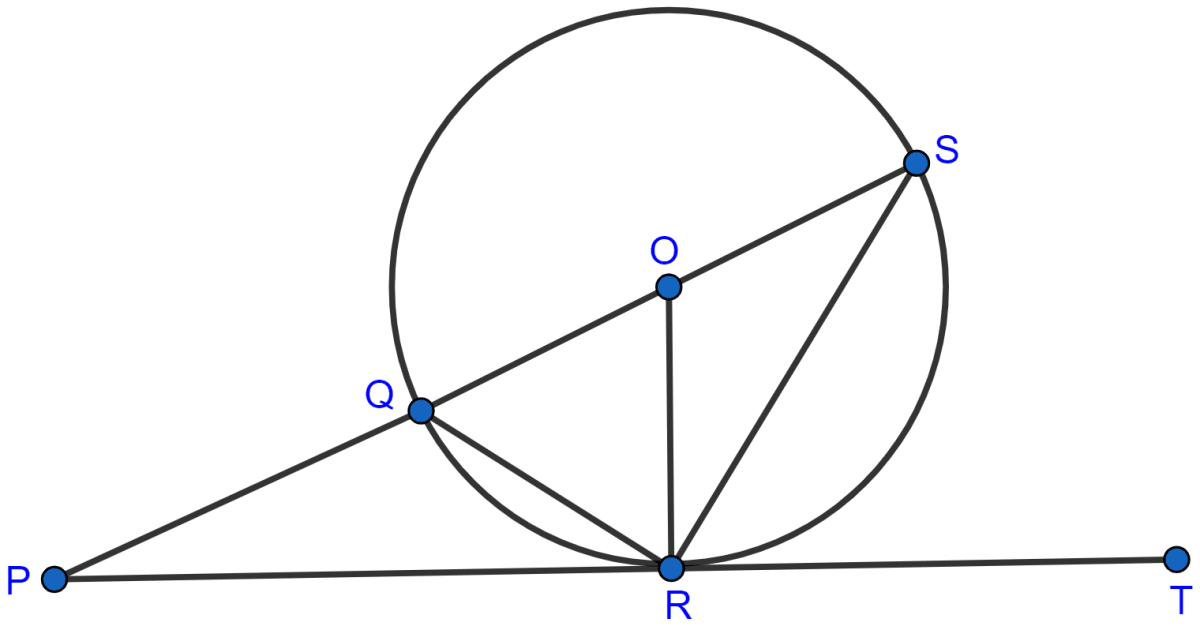
In the given figure, PT touches the circle with center O at point R. Diameter SQ is produced to meet the tangent TR at P.
Given ∠SPR = x° and ∠QRP = y°;
prove that :
(i) ∠ORS = y°
(ii) write an expression connecting x and y.
PT is a tangent to the circle at T. If ∠ABC = 70° and ∠ACB = 50°; calculate :
(i) ∠CBT
(ii) ∠BAT
(iii) ∠APT