Mathematics
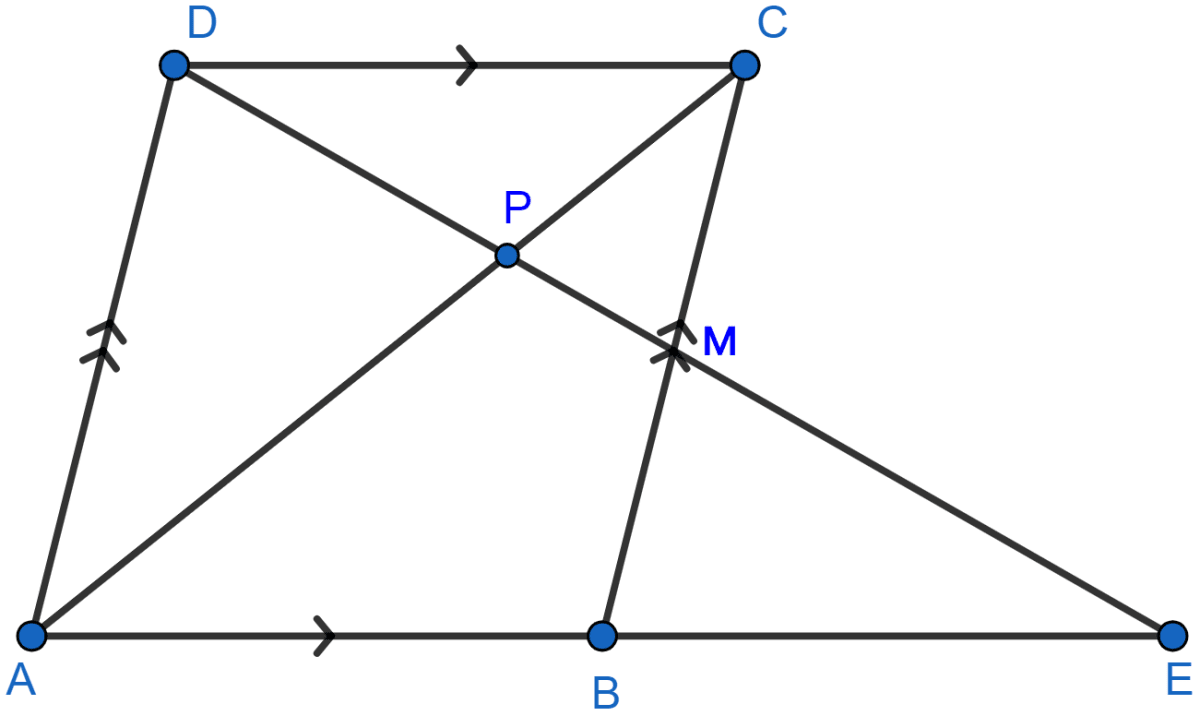
In the following figure, M is the mid-point of BC of a parallelogram ABCD. DM intersects the diagonal AC at P and AB produced at E. Prove that : PE = 2PD.
Similarity
18 Likes
Answer
In ∆BME and ∆DMC,
∠BME = ∠CMD [Vertically opposite angles are equal.]
∠MCD = ∠MBE [Alternate angles are equal]
BM = MC [M is mid-point of BC]
∴ ∆BME ≅ ∆DMC [By AAS congruence rule]
∴ BE = DC [By C.P.C.T]
Since, opposite sides of parallelogram are equal.
∴ AB = DC
or, AB = DC = BE. ………..(1)
In ∆DCP and ∆EPA,
∠DPC = ∠EPA [Vertically opposite angles are equal.]
∠CDP = ∠AEP [Alternate angles are equal]
∴ ∆DCP ~ ∆EAP [By AA]
Since, corresponding sides of similar triangles are proportional we have :
Hence proved that PE = 2PD.
Answered By
9 Likes
Related Questions
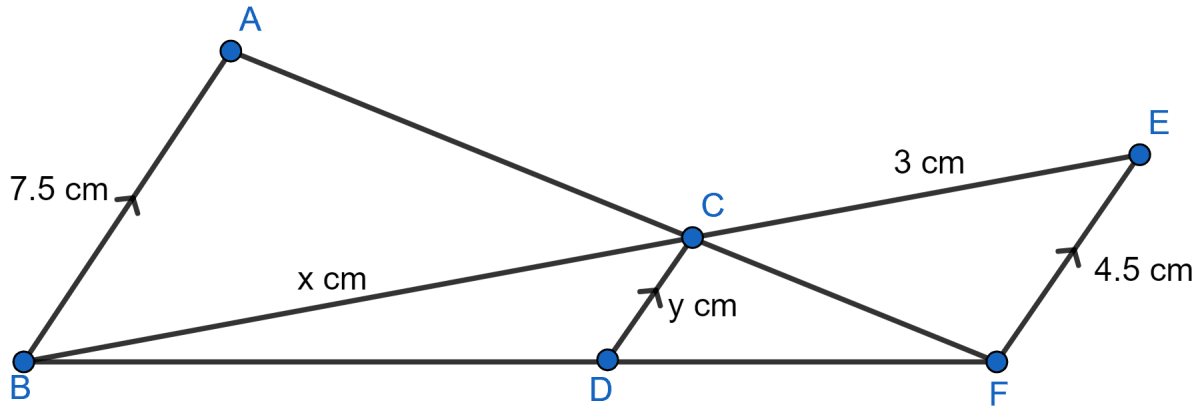
In the figure, given below, AB, CD and EF are parallel lines. Given AB = 7.5 cm, DC = y cm, EF = 4.5 cm, BC = x cm and CE = 3 cm, calculate the values of x and y.
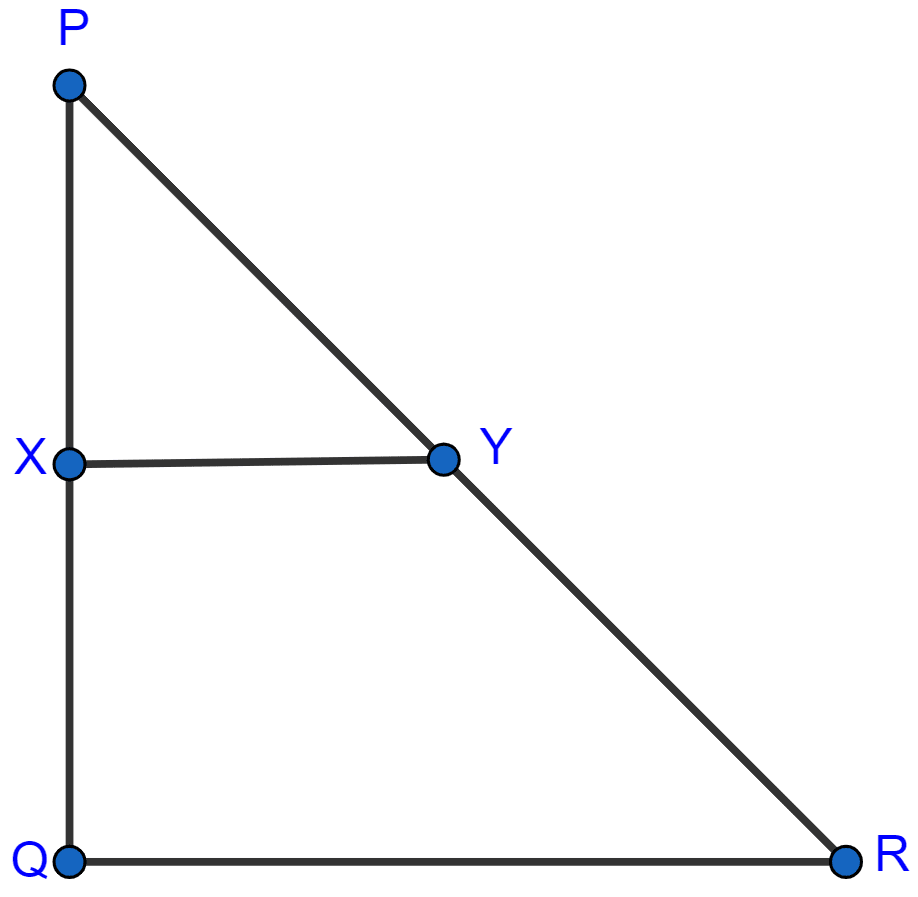
In the figure, given below, PQR is a right-angled triangle at Q. XY is parallel to QR, PQ = 6 cm, PY = 4 cm and PX : XQ = 1 : 2. Calculate the lengths of PR and QR.
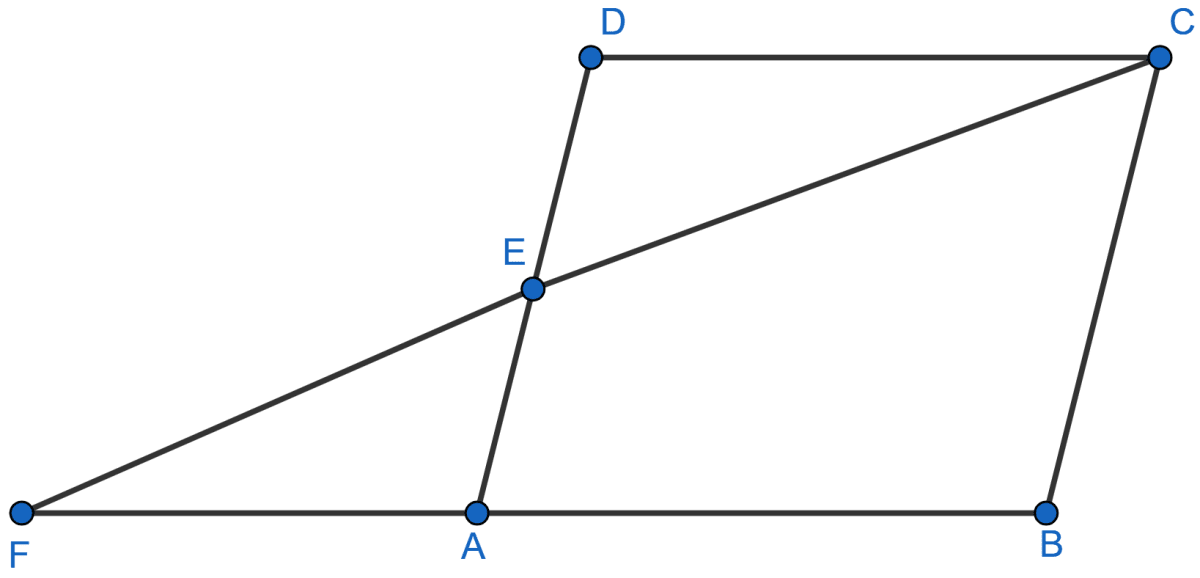
The given figure shows a parallelogram ABCD. E is a point in AD and CE produced meets BA produced at point F. If AE = 4 cm, AF = 8 cm and AB = 12 cm, find the perimeter of the parallelogram ABCD.
In the given figure, AE = 5 cm and EC = 7 cm, then area of △ ADE : area of △ ABC is :
5 : 7
7 : 5
25 : 144
144 : 25
