Mathematics

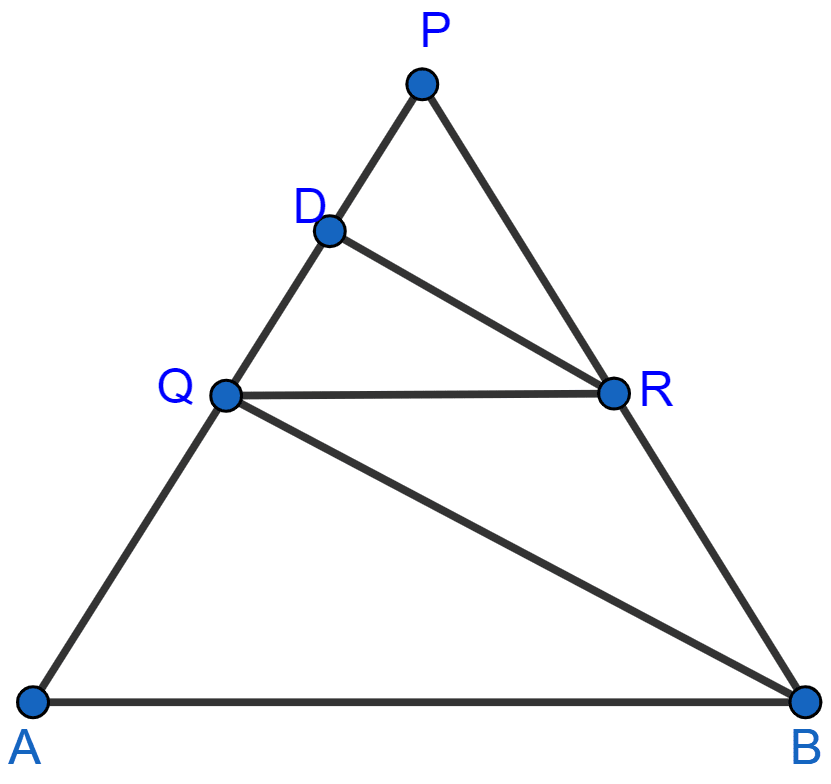
In the figure, QR is parallel to AB and DR is parallel to QB.
Prove that : PQ² = PD × PA.

10 Likes

In △PQR and △PAB,

∠PQR = ∠PAB [Corresponding angles are equal]

∠PRQ = ∠PBA [Corresponding angles are equal]

Hence, △PQR ~ △PAB [By AA]

Since, corresponding sides of similar triangles are proportional to each other.

$\therefore \dfrac{PQ}{PA} = \dfrac{PR}{PB}$ ……..(1)

In △PDR and △PQB,

∠PDR = ∠PQB [Corresponding angles are equal]

∠PRD = ∠PBQ [Corresponding angles are equal]

Hence, △PDR ~ △PQB [By AA]

Since, corresponding sides of similar triangles are proportional to each other.

$\therefore \dfrac{PD}{PQ} = \dfrac{PR}{PB}$ ……..(2)

From (1) and (2) we get :

$\Rightarrow \dfrac{PQ}{PA} =\dfrac{PD}{PQ} \\[1em] \Rightarrow PQ^2 = PD \times PA.$

Hence, proved that PQ² = PD × PA.

Answered By

7 Likes