Mathematics
In the figure (ii) given below, D is any point on the side BC of △ABC. If AB > AC, show that AB > AD.
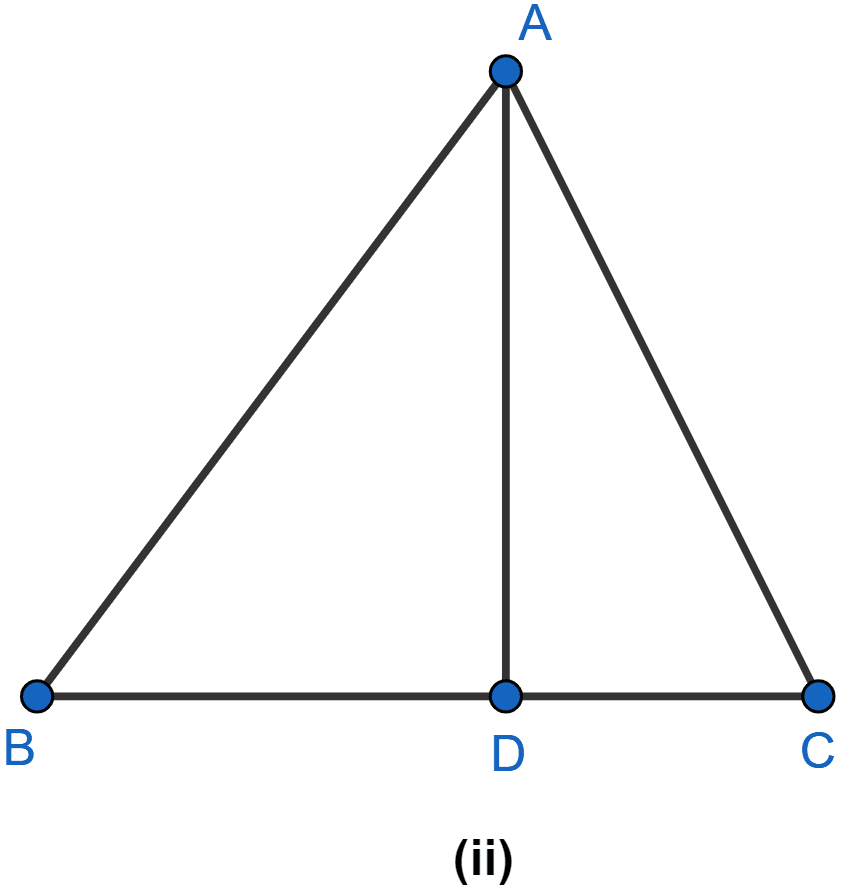
Answer
Given,
AB > AC
∴ ∠ACB > ∠ABC (As angle opposite to greater side is greater.)
From figure,
∠ADB = ∠ACB + ∠DAC (As exterior angle is equal to sum of two opposite interior angles.)
⇒ ∠ADB > ∠ACB
⇒ ∠ADB > ∠ABC [∵ ∠ACB > ∠ABC]
∴ AB > AD (As side opposite to greater angle is greater.)
Hence, proved that AB > AD.
Related Questions
Is it possible to construct a triangle with lengths of its sides as 9 cm, 7 cm and 17 cm? Give reason for your answer.
Is it possible to construct a triangle with lengths of its sides as 4 cm, 3 cm and 7 cm? Give reason for your answer.
In the figure (3) given below, AC = CD. Prove that BC < CD.

In the figure (i) given below, ∠B < ∠A and ∠C < ∠D. Show that AD < BC.
