Mathematics
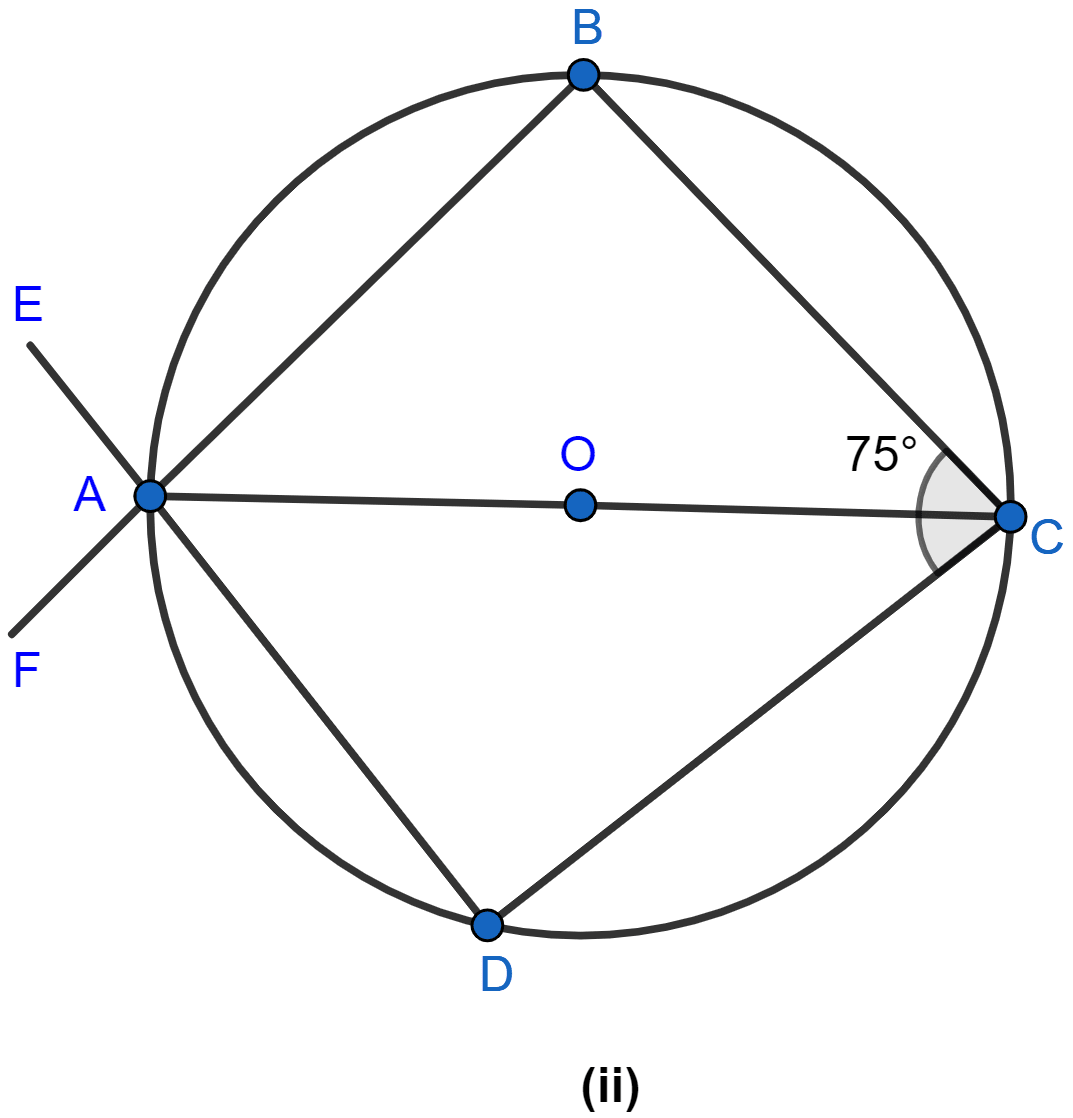
In the figure (ii) given below, AC is a diameter of the given circle and ∠BCD = 75°. Calculate the size of
(i) ∠ABC
(ii) ∠EAF
Answer
(i) From figure,
∠ABC = 90° (∵ angle in semicircle is equal to 90°)
Hence, the value of ∠ABC = 90°.
(ii) Since opposite angles sum is 180° in cyclic quadrilateral
⇒ ∠BAD + ∠BCD = 180°
⇒ ∠BAD + 75° = 180°
⇒ ∠BAD = 180° - 75°
⇒ ∠BAD = 105°.
From figure,
∠EAF = ∠BAD = 105°.
Hence, the value of ∠BAD = 105°.
Related Questions
In the figure (ii) given below, AB is parallel to DC, ∠BCE = 80° and ∠BAC = 25°. Find :
(i) ∠CAD
(ii) ∠CBD
(iii) ∠ADC

If O is the center of the circle find the value of x in the following figure (using the given information) :

In the figure (i) given below, if ∠DBC = 58° and BD is a diameter of the circle, calculate
(i) ∠BDC
(ii) ∠BEC
(iii) ∠BAC

In the figure (i) given below, O is the center of the circle. If ∠AOC = 150°, find
(i) ∠ABC
(ii) ∠ADC.
