Mathematics
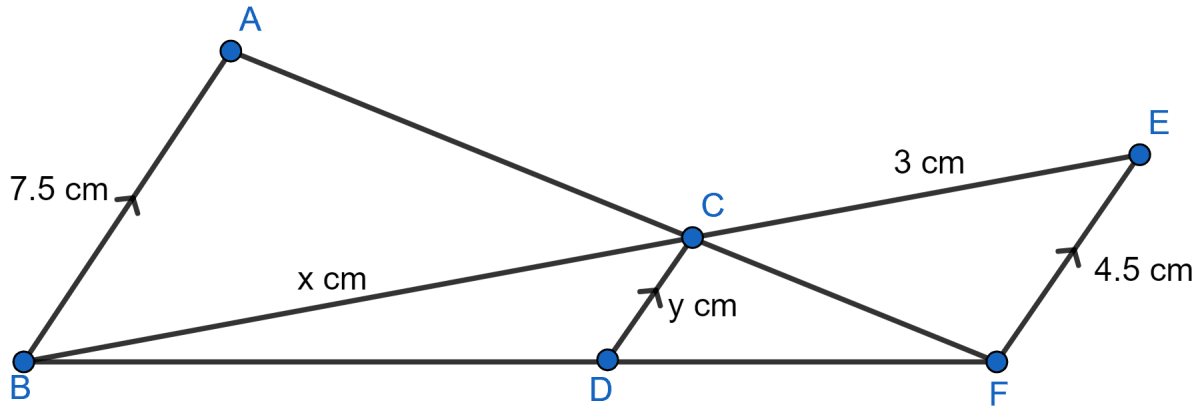
In the figure, given below, AB, CD and EF are parallel lines. Given AB = 7.5 cm, DC = y cm, EF = 4.5 cm, BC = x cm and CE = 3 cm, calculate the values of x and y.
Similarity
10 Likes
Answer
In ∆BEF,
DC || FE
So, by basic proportionality theorem,
…..(1)
Let BD = ax and DF = 3a.
From figure,
BF = BD + DF = ax + 3a = a(x + 3).
…..(2)
In ∆AFB and CDF,
∠AFB = ∠CFD [Common angles]
∠ABF = ∠CDF [Corresponding angles are equal]
∴ ∆AFB ~ ∆CDF [By AA]
Since, corresponding sides of similar triangles are proportional.
In ∆BCD and ∆BEF ,
∠FBE = ∠DBC [Common angles]
∠CDB = ∠EFB [Corresponding angles are equal]
∴ ∆BEF ~ ∆BCD [By AA]
Since, corresponding sides of similar triangles are proportional.
Let BD = ay and BF = 4.5a
From figure,
DF = BF - BD = 4.5a - ay = a(4.5 - y).
…..(5)
From (1) and (5) we get,
Substituting value of y from (4) in above equation we get,
Since, side of triangle cannot be negative. So, x = 5 cm.
Substituting value of x in (4) we get,
Hence, x = 5 cm and y = 2.8125 cm
Answered By
5 Likes
Related Questions
In the given figure, ΔABC ~ ΔADE. If AE : EC = 4 : 7 and DE = 6.6 cm, find BC. If 'x' be the length of the perpendicular from A to DE, find the length of perpendicular from A to BC in terms of 'x'.
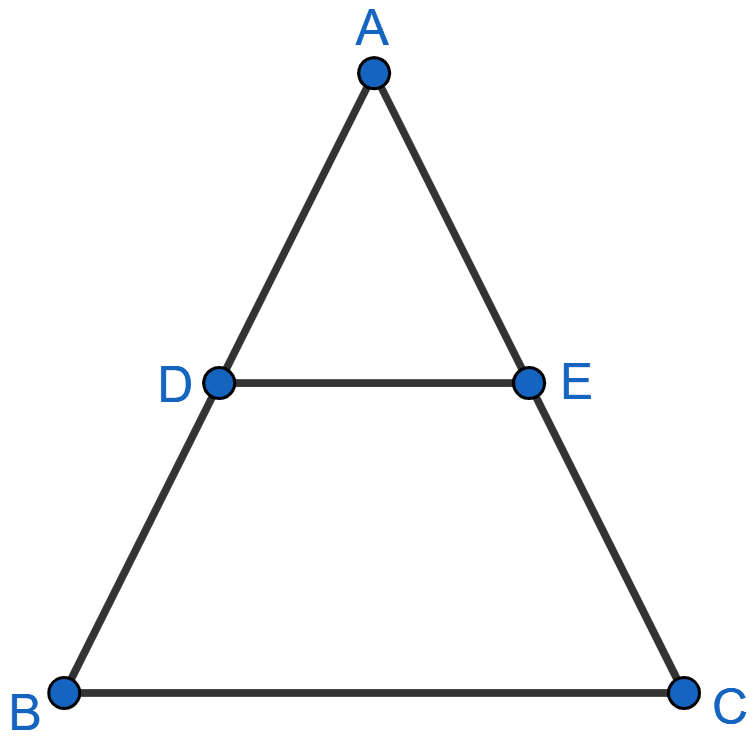
A line segment DE is drawn parallel to base BC of ∆ABC which cuts AB at point D and AC at point E. If AB = 5BD and EC = 3.2 cm, find the length of AE.
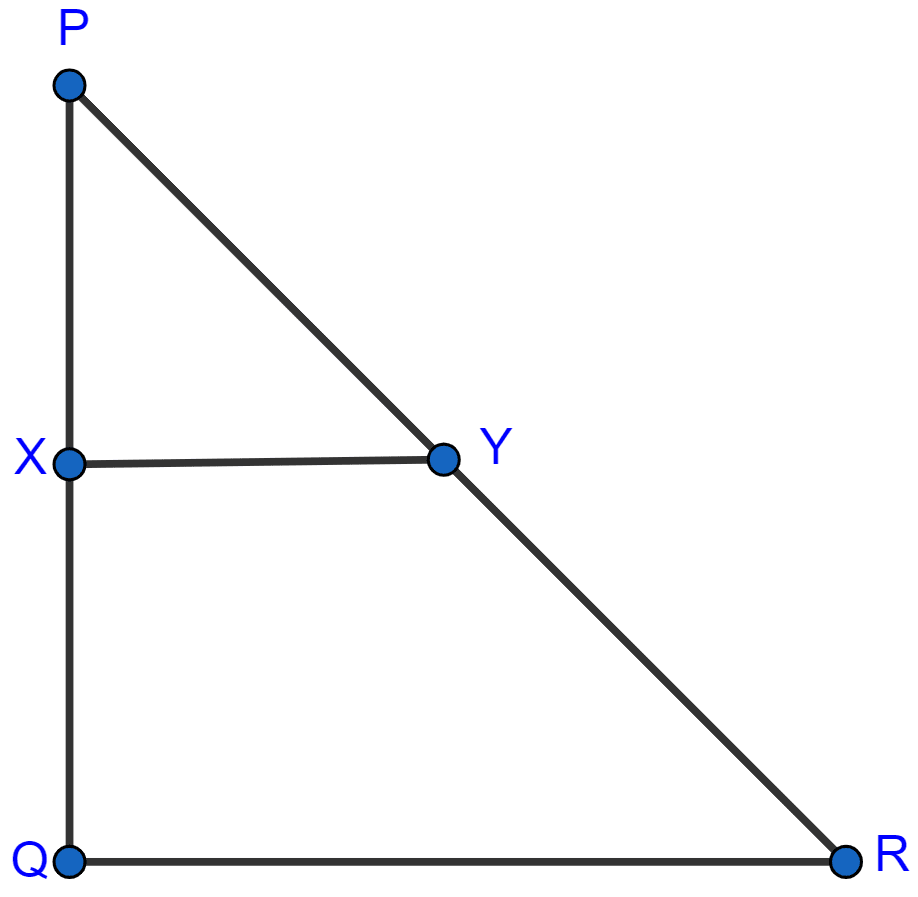
In the figure, given below, PQR is a right-angled triangle at Q. XY is parallel to QR, PQ = 6 cm, PY = 4 cm and PX : XQ = 1 : 2. Calculate the lengths of PR and QR.
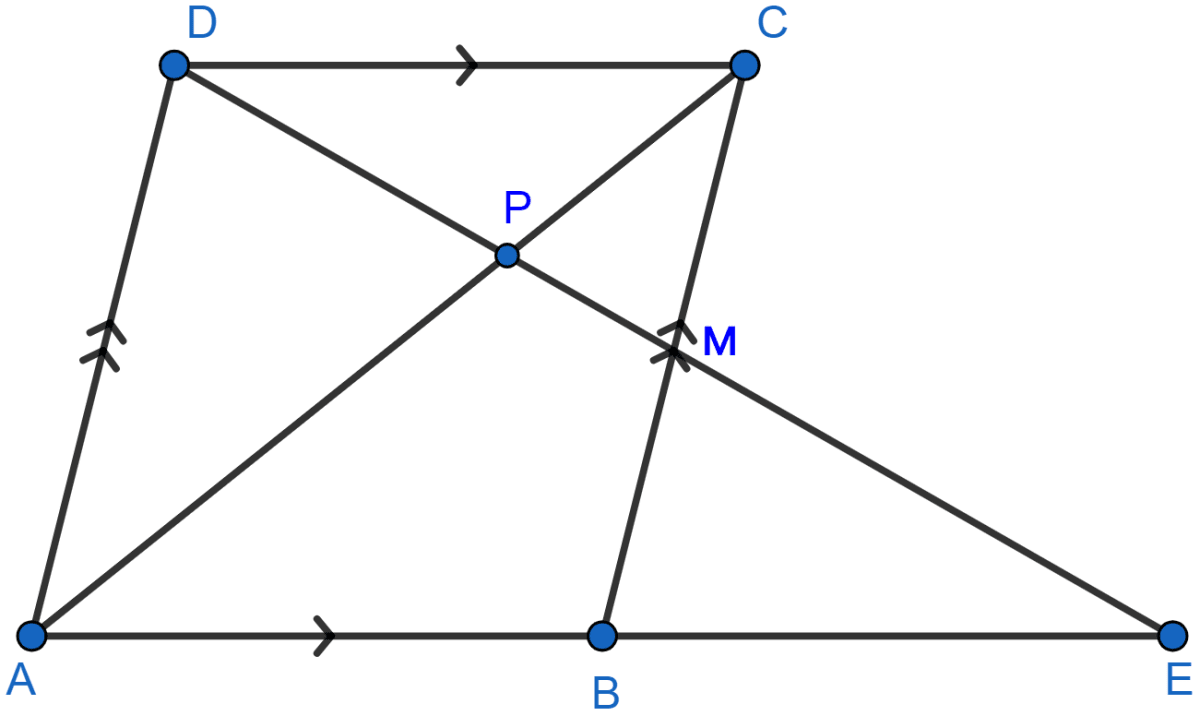
In the following figure, M is the mid-point of BC of a parallelogram ABCD. DM intersects the diagonal AC at P and AB produced at E. Prove that : PE = 2PD.