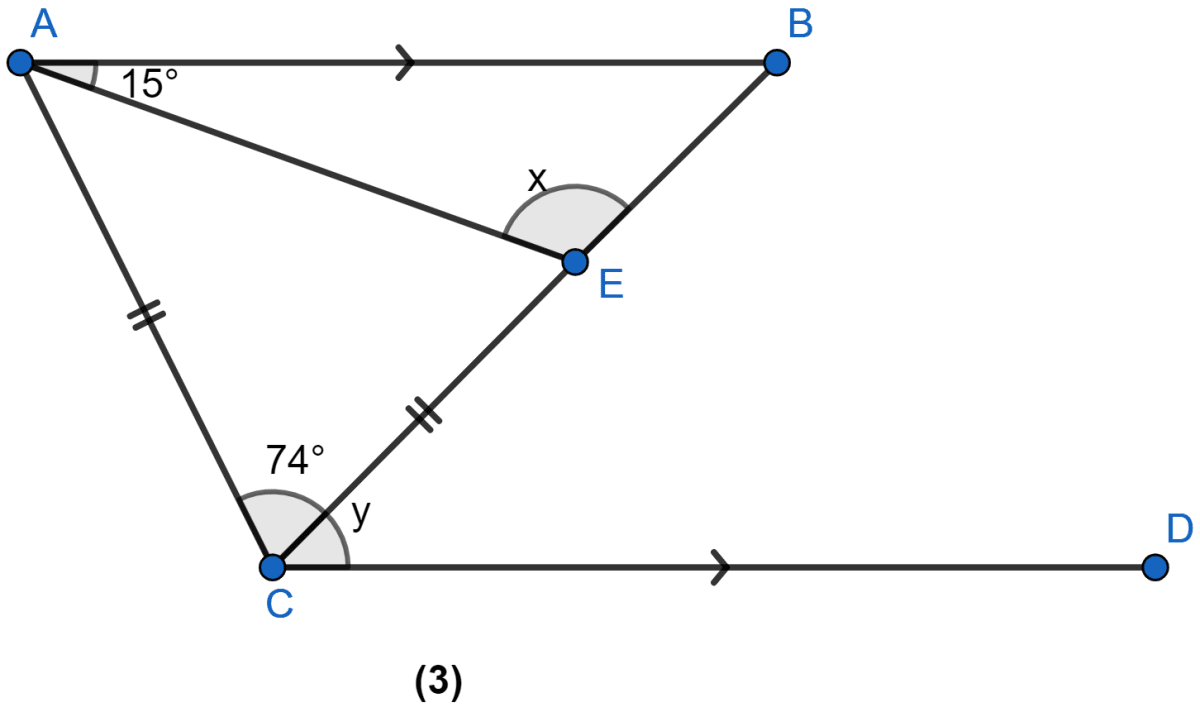
In the figure (3) given below, AB || CD and CA = CE. If ∠ACE = 74° and ∠BAE = 15°, find the values of x and y.

From figure,

∠CAE = ∠CEA = a (Angles opposite to equal side are equal.)

In △ACE

⇒ ∠CAE + ∠CEA + ∠ACE = 180°

⇒ a + a + 74° = 180°

⇒ 2a = 106°

⇒ a = 53°.

From figure,

∠CEA + ∠AEB = 180°

a + x = 180°

53° + x = 180°

x = 180° - 53° = 127°.

In △AEB,

⇒ ∠EAB + ∠AEB + ∠ABE = 180°

⇒ 15 + 127° + ∠ABE = 180°

⇒ 142° + ∠ABE = 180°

⇒ ∠ABE = 180° - 142° = 38°.

From figure,

y = ∠ABE (Alternate angles)

∴ y = 38°.

Hence, x = 127° and y = 38°.