Mathematics
In the figure (2) given below, CA ∥ BD, the lines AB and CD meet at O.
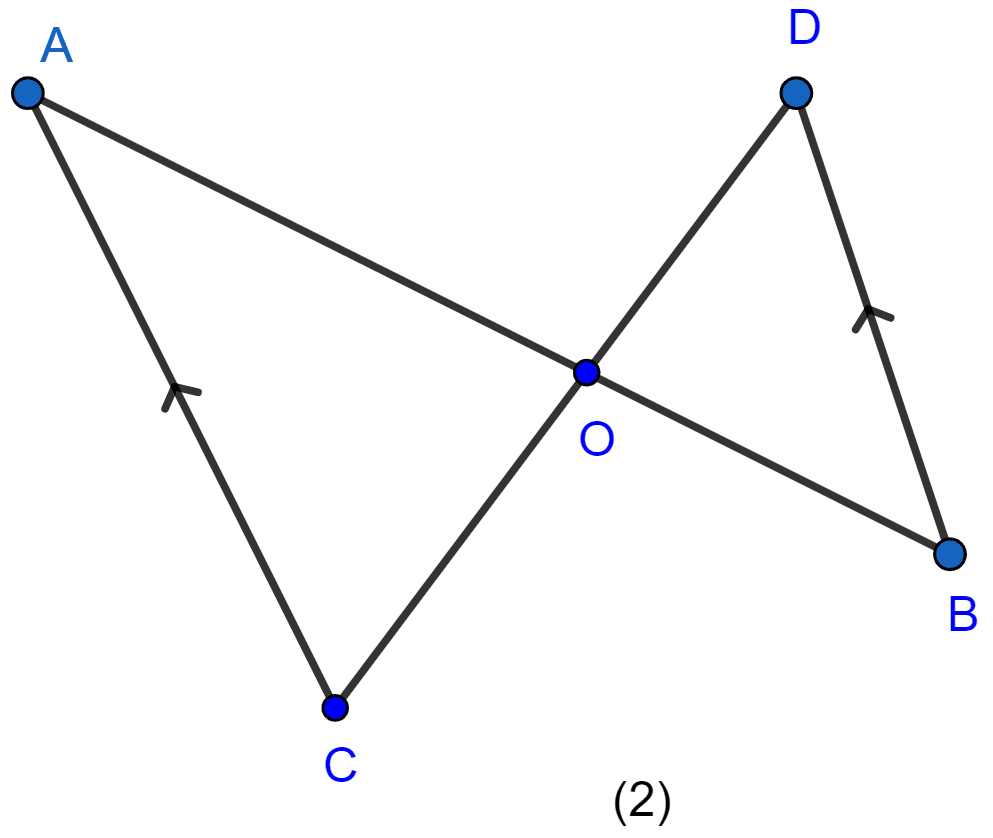
(i) Prove that △ACO ~ △BDO.
(ii) If BD = 2.4 cm, OD = 4 cm, OB = 3.2 cm and AC = 3.6 cm, calculate OA and OC.
Similarity
42 Likes
Answer
Considering △ACO and △BDO,
∠ AOC = ∠ BOD [Vertically opposite angles]
∠ A = ∠ B [Alternate angles]
Then, by AA rule of similarity, △AOC ~ △BOD.
So,
Now, consider
Hence, OA = 4.8 cm and OC = 6 cm.
Answered By
18 Likes
Related Questions
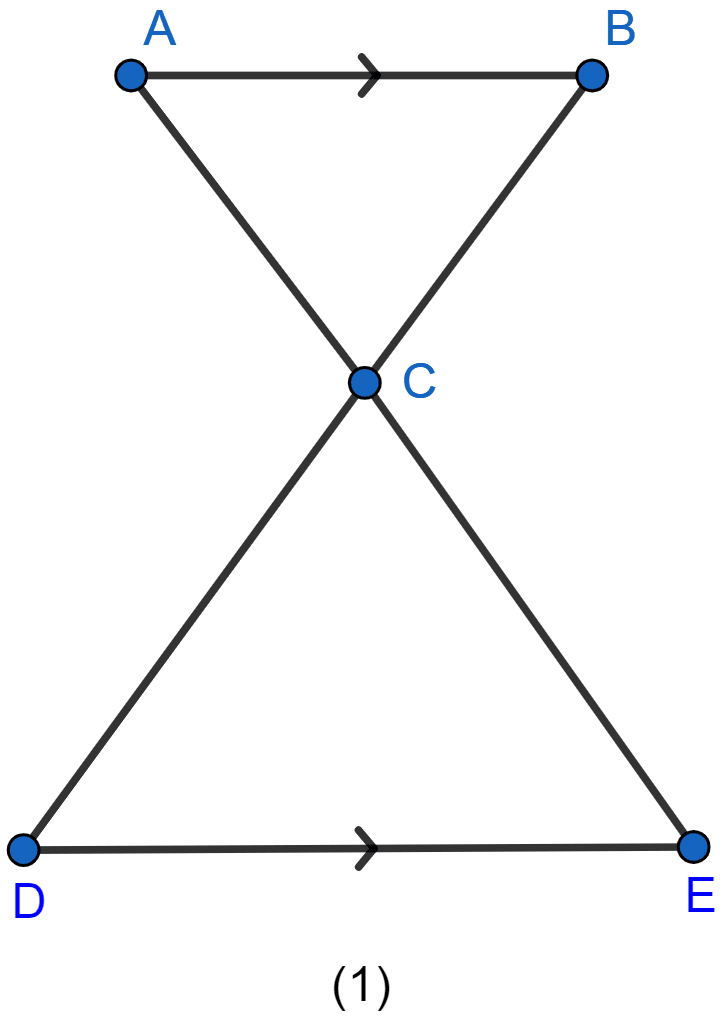
In the figure given below, AB ∥ DE, AC = 3cm, CE = 7.5cm and BD = 14cm. Calculate CB and DC.
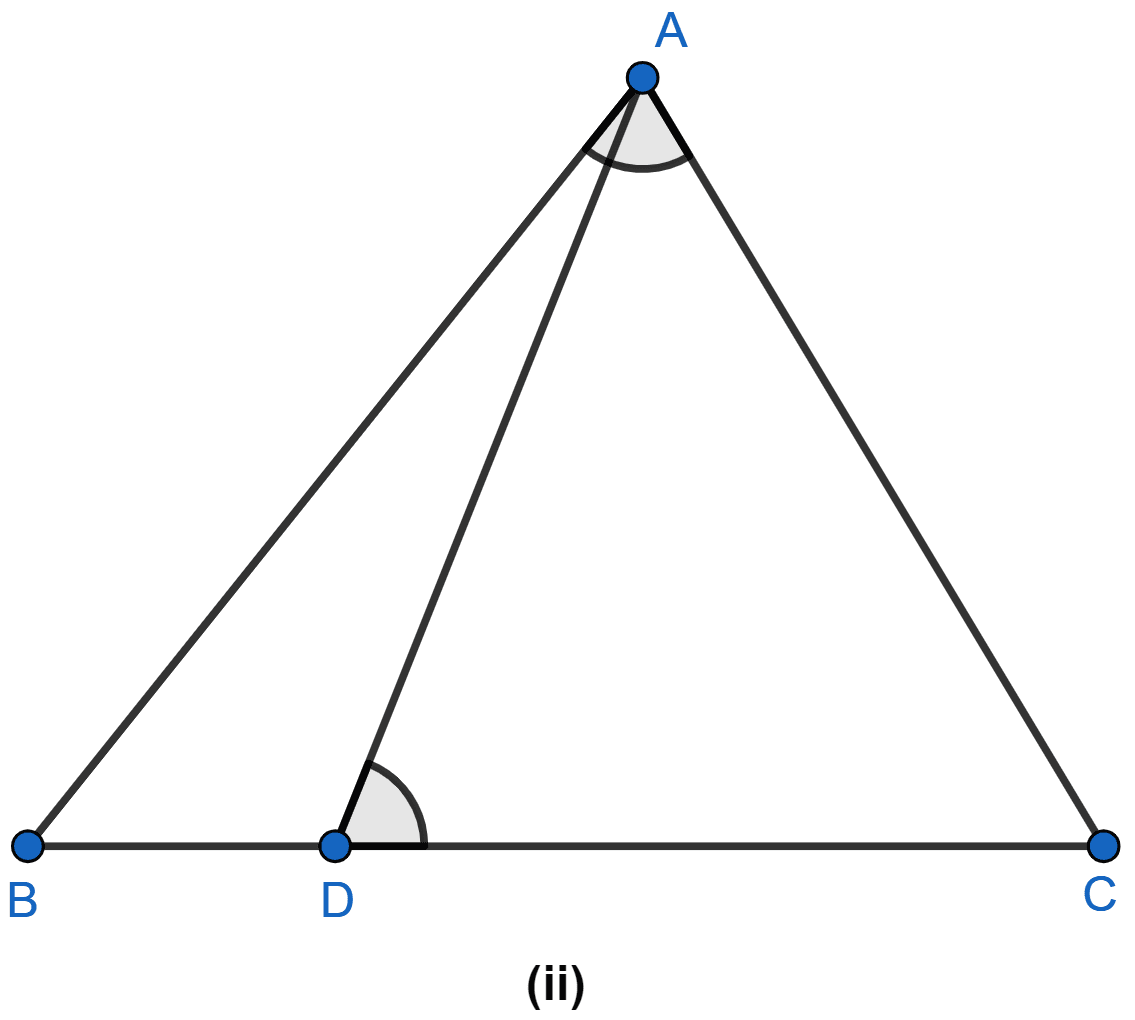
In the figure (ii) given below, ∠ADC = ∠BAC. Prove that CA2 = DC × BC.
Calculate the other sides of a triangle whose shortest side is 6cm and which is similar to a triangle whose sides are 4cm, 7cm and 8cm.
In the figure (i) given below, ∠P = ∠RTS. Prove that △RPQ ~ △RTS.
