Mathematics
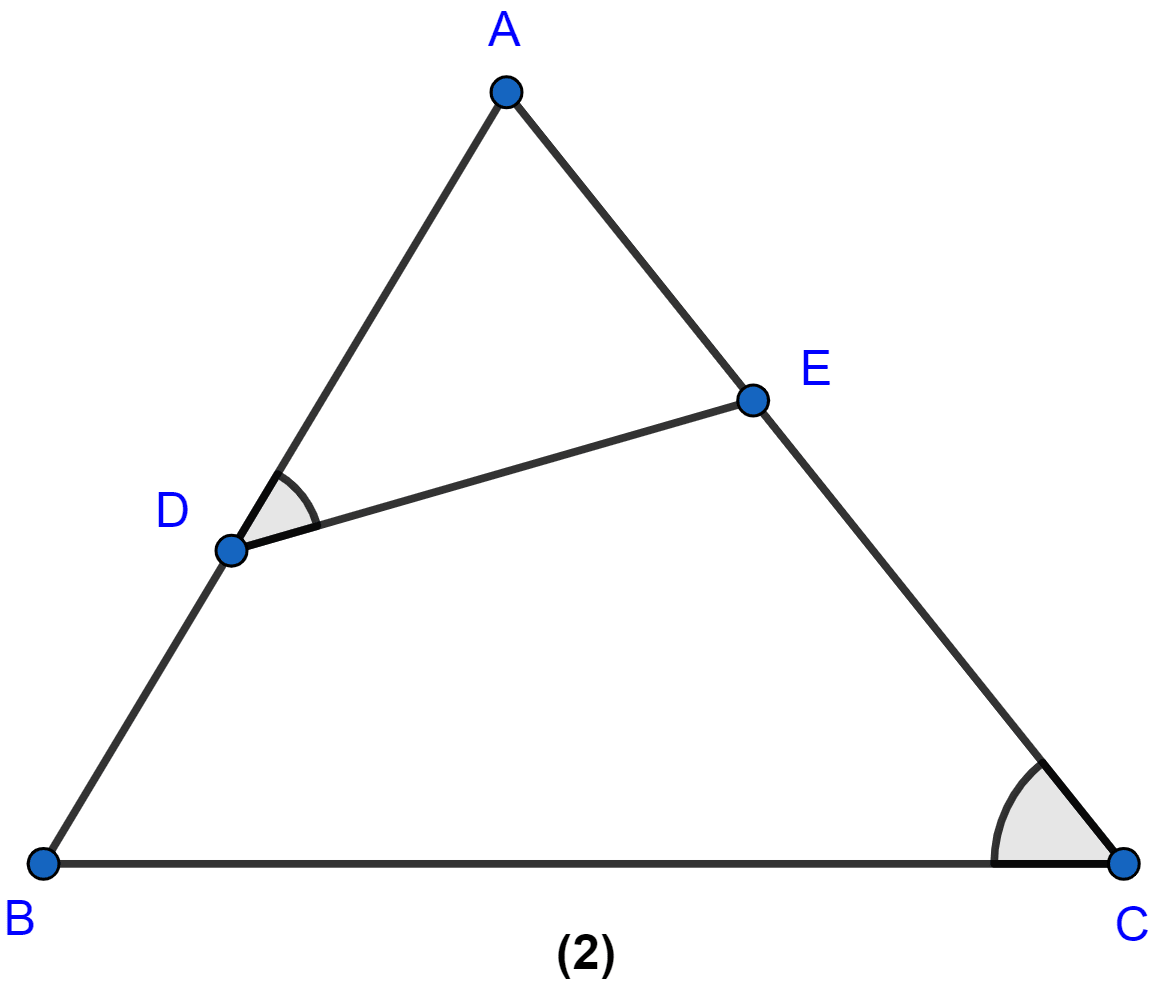
In the figure (2) given below, ∠ ADE = ∠ ACB.
(i) Prove that △s ABC and AED are similar.
(ii) If AE = 3 cm, BD = 1 cm and AB = 6 cm, calculate AC.
Similarity
51 Likes
Answer
(i) From the given figure,
∠ ADE = ∠ ACB (Given)
∠ A = ∠ A. (Common)
Hence, by AA rule of similarity △ABC ~ △AED.
(ii) Since triangles are similar,
From figure:
AD = AB - BD = 6 - 1 = 5 cm.
Consider,
Hence, the length of AC = 10 cm.
Answered By
30 Likes
Related Questions
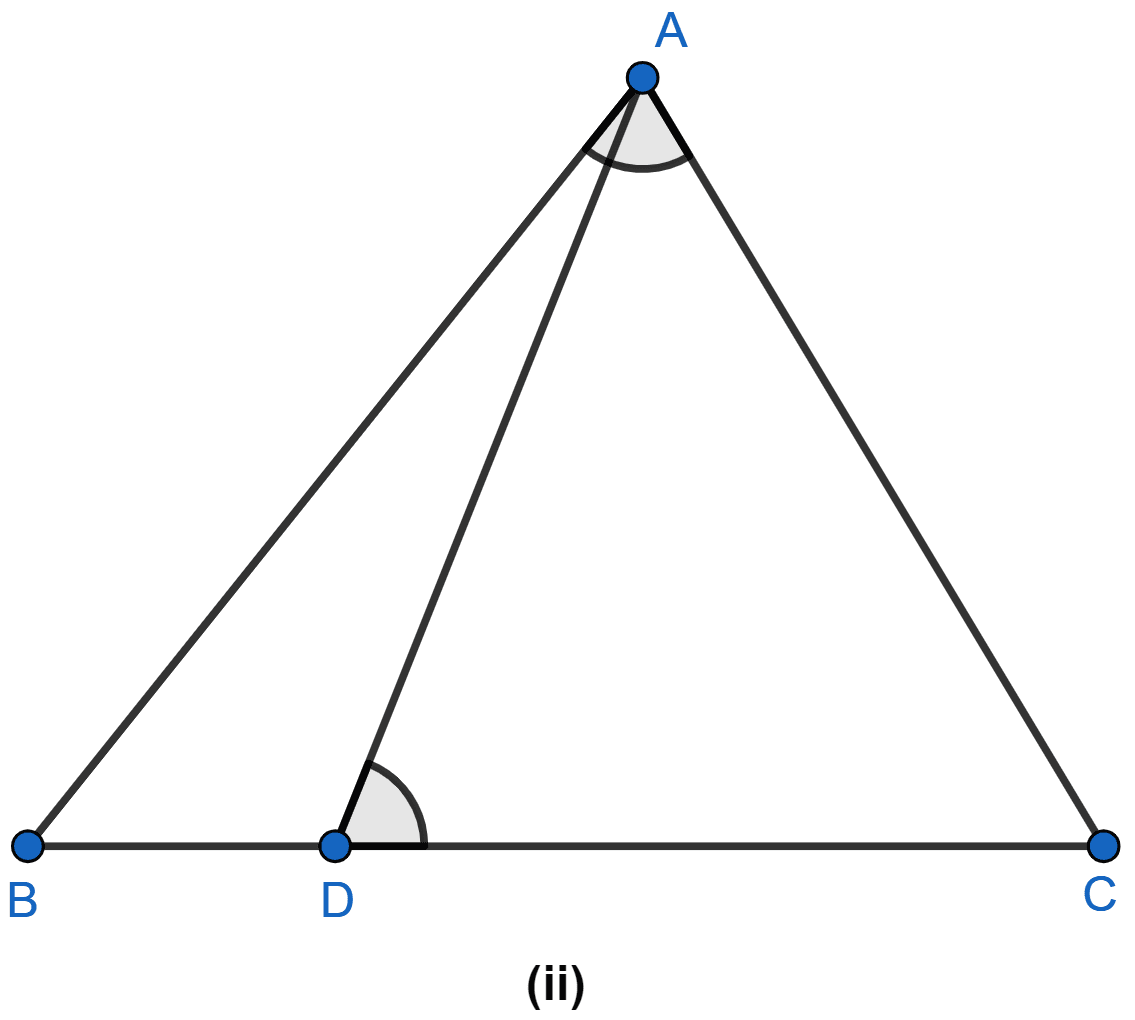
In the figure (ii) given below, ∠ADC = ∠BAC. Prove that CA2 = DC × BC.
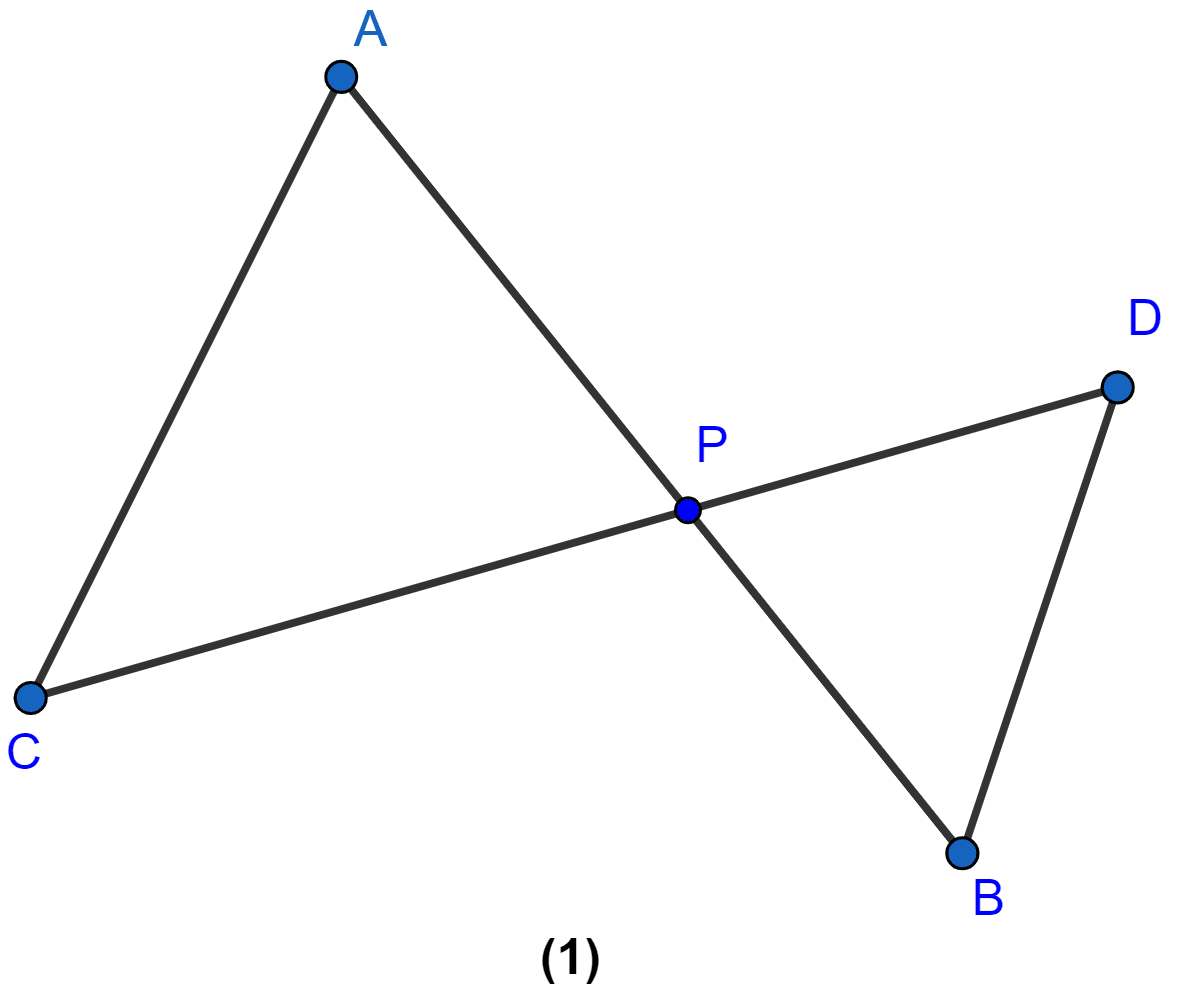
In the figure (1) given below, AP = 2PB and CP = 2PD.
(i) Prove that △ACP is similar to △BDP and AC ∥ BD.
(ii) If AC = 4.5 cm, calculate the length of BD.
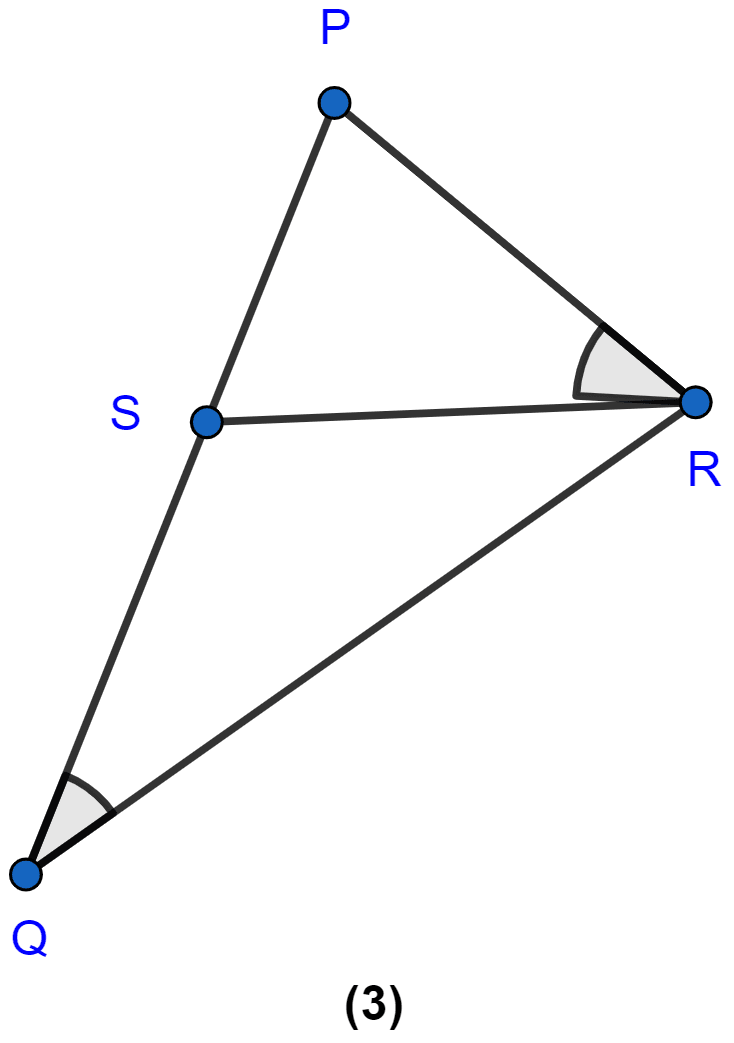
In the figure (3) given below, ∠ PQR = ∠ PRS. Prove that triangles PQR and PRS are similar. If PR = 8 cm, PS = 4 cm, calculate PQ.
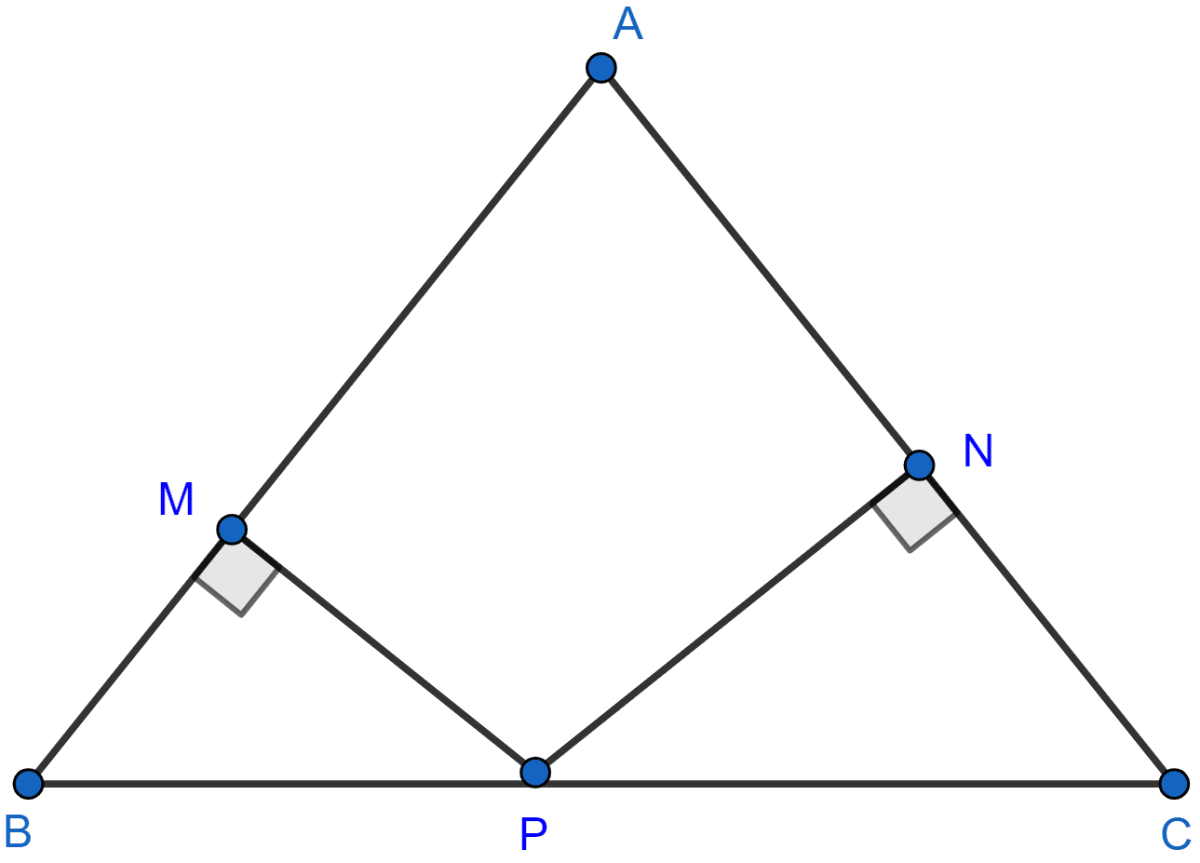
In the adjoining figure, ABC is a triangle in which AB = AC. P is a point on the side BC such that PM ⊥ AB and PN ⊥ AC. Prove that BM × NP = CN × MP.