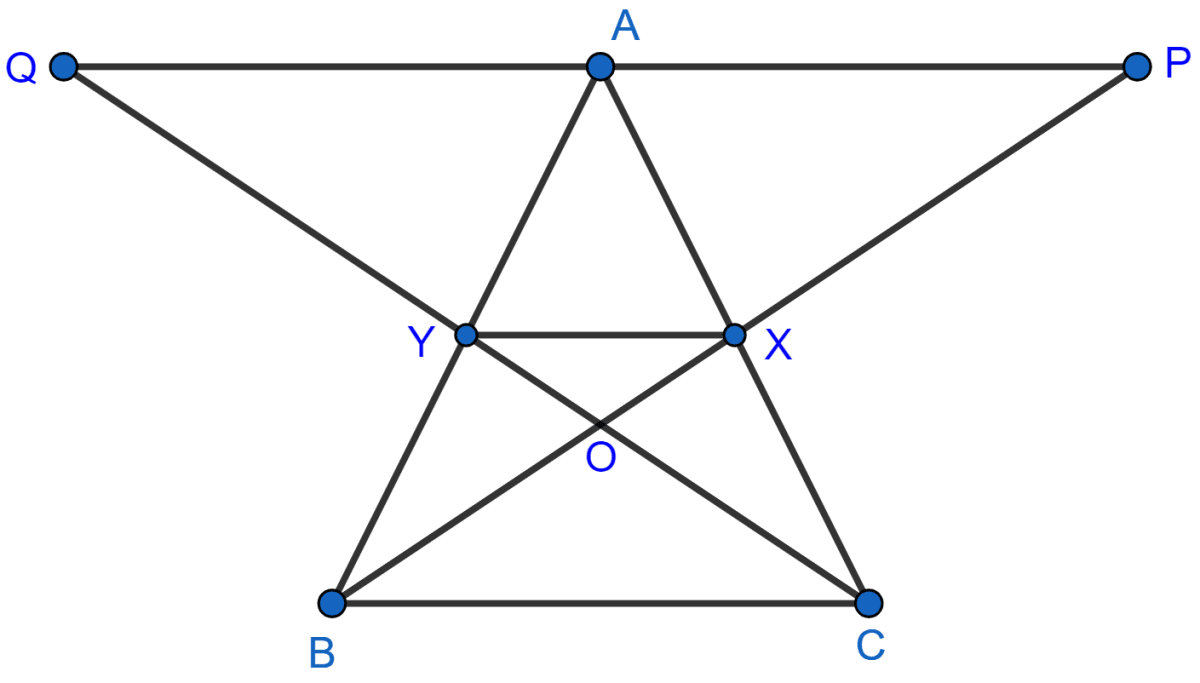
In the adjoining figure, X and Y are mid-points of the sides AC and AB respectively of ∆ABC. QP || BC and CYQ and BXP are straight lines. Prove that area of ∆ABP = area of ∆ACQ.

X and Y are the mid-points of sides AC and AB respectively.

Since, X and Y are midpoints of AC and AB respectively.

In ∆ABC,

XY || BC (By midpoint theorem).

Given, QP || BC

∴ QP || BC || XY

In ∆BAP, Y is mid of AB and XY || AP

∴ X is mid-point of BP (Converse of mid-point theorem)

∴ XY = $\dfrac{1}{2}$AP …….(1)

Similarly we can prove in ∆AQC

X is mid-point of AC and XY is parallel to QA

∴ Y is mid-point of QC (Converse of mid-point theorem)

XY = $\dfrac{1}{2}$ QA …….(2)

From (1) and (2),

⇒ $\dfrac{1}{2}QA = \dfrac{1}{2}AP$

⇒ QA = AP.

Thus, ∆ABP and ∆ACQ are on the equal bases (QA = AP) and between the same parallel lines BC and QP

∴ Area of ∆ABP = Area of ∆ACQ.

Hence, proved that Area of ∆ABP = Area of ∆ACQ.