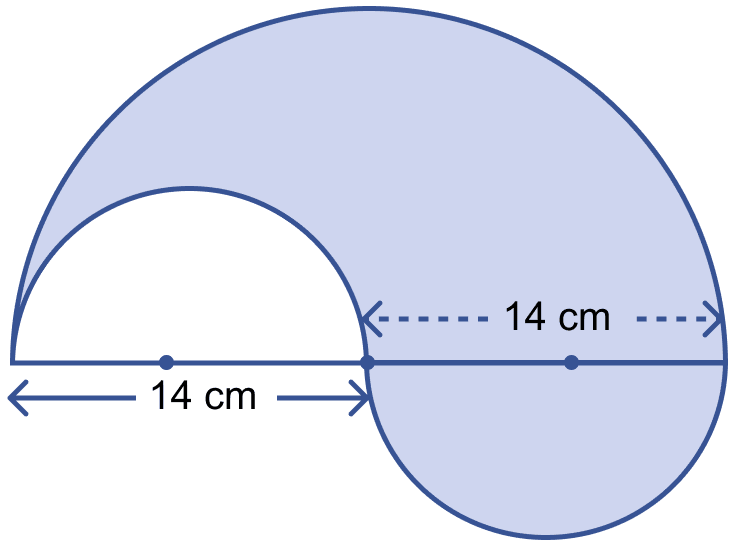
In the adjoining figure, the boundary of the shaded region consists of semicircular arcs. The area of the shaded region is equal to

1. 616 cm²

2. 385 cm²

3. 231 cm²

4. 308 cm²

From figure,

Radius of larger circle (R) = 14 cm.

Area of shaded region = Area of larger circle - Area of 1st smaller circle + Area of 2nd smaller circle …….(1)

From figure,

Diameter of both the smaller semi-circles = 14 cm.

∴ Radius = 7 cm and area of both the circle are equal.

∴ Area of shaded region = Area of larger circle = $\dfrac{πR^2}{2}$

$= \dfrac{1}{2} \times \dfrac{22}{7} \times (14)^2 \\[1em] = \dfrac{11}{7} \times 196 \\[1em] = 11 \times 28 \\[1em] = 308 \text{ cm}^2.$

Hence, Option 4 is the correct option.