Mathematics
In the adjoining figure, find the values of x and y.
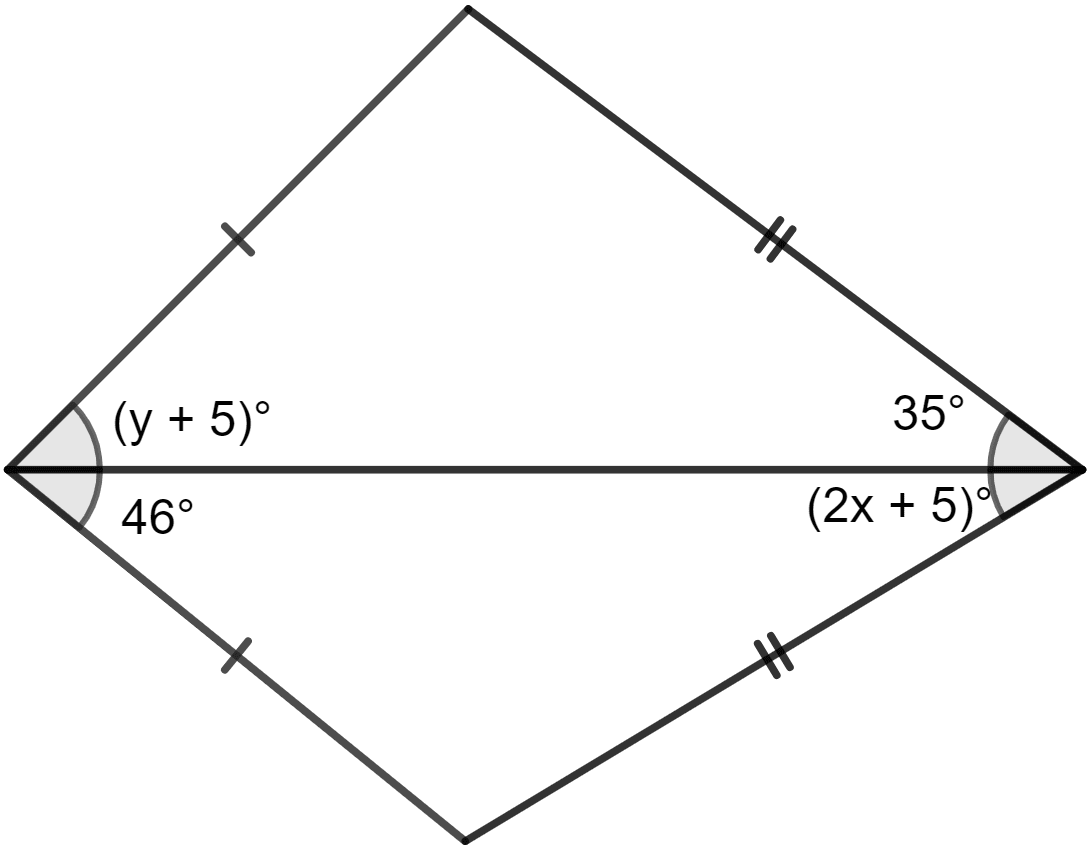
Triangles
100 Likes
Answer
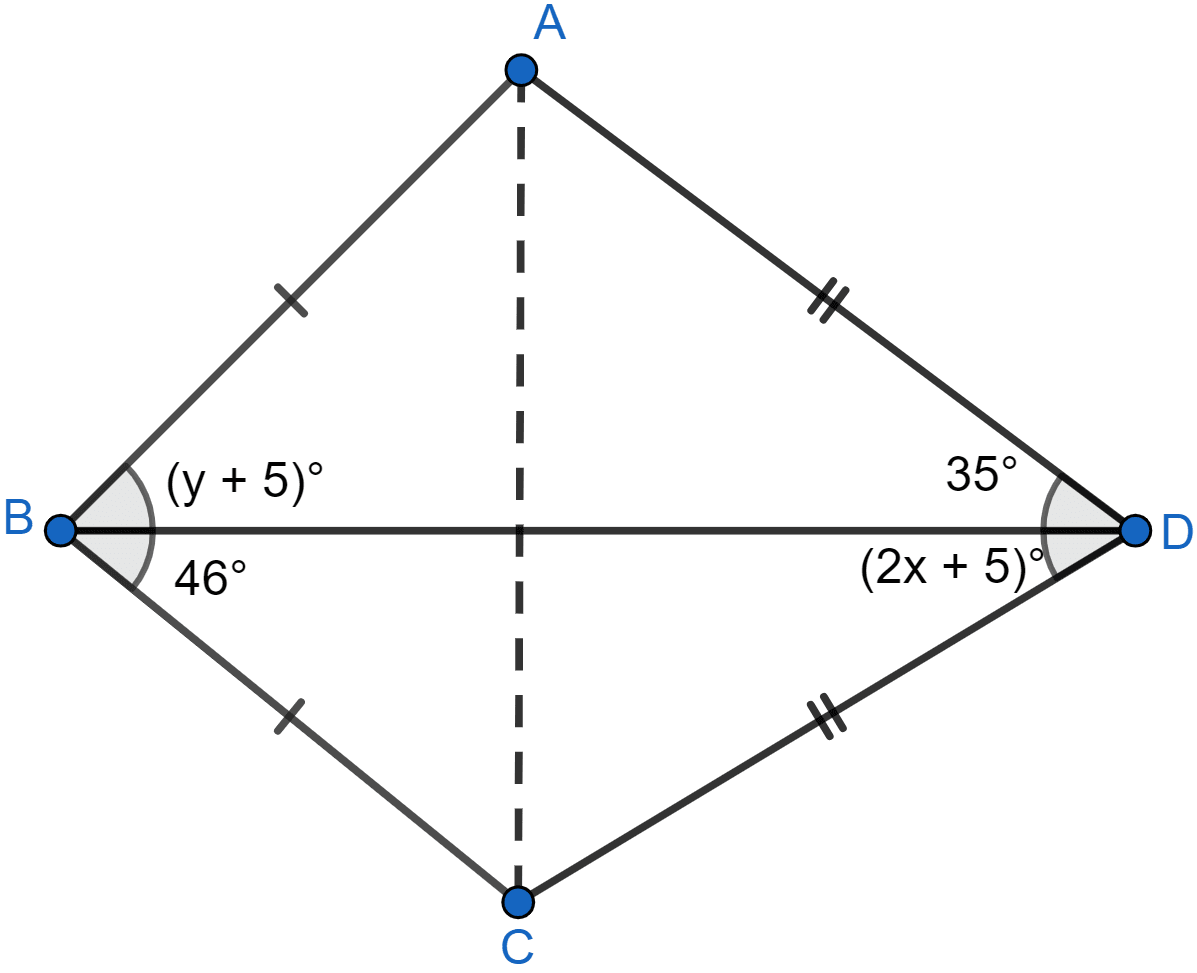
In △ABD and △CBD,
Given,
AB = BC (Given)
AD = CD (Given)
BD = BD (Common)
Hence, by SSS axiom △ABD ≅ △CBD.
We know that corresponding angles of congruent triangles are equal.
∴ (y + 5)° = 46° and (2x + 5)° = 35°
y° = 41° and 2x° = 30°
y = 41 and x = 15.
Hence, x = 15 and y = 41.
Answered By
65 Likes
Related Questions
In the adjoining figure, AB = AC and D is the midpoint of BC. Use SSS rule of congruency to show that
(i) △ABD ≅ △ACD
(ii) AD is bisector of ∠A
(iii) AD is perpendicular to BC.

Two line segments AB and CD bisect each other at O. Prove that
(i) AC = BD
(ii) ∠CAB = ∠ABD
(iii) AD || CB
(iv) AD = CB
In triangles ABC and PQR, ∠A = ∠Q and ∠B = ∠R. Which side of △PQR should be equal to side AB of △ABC so that the two triangles are congruent? Give reason for your answer.
In triangles ABC and PQR, ∠A = ∠Q and ∠B = ∠R. Which side of △PQR should be equal to side BC of △ABC so that the two triangles are congruent? Give reason for your answer.