Mathematics
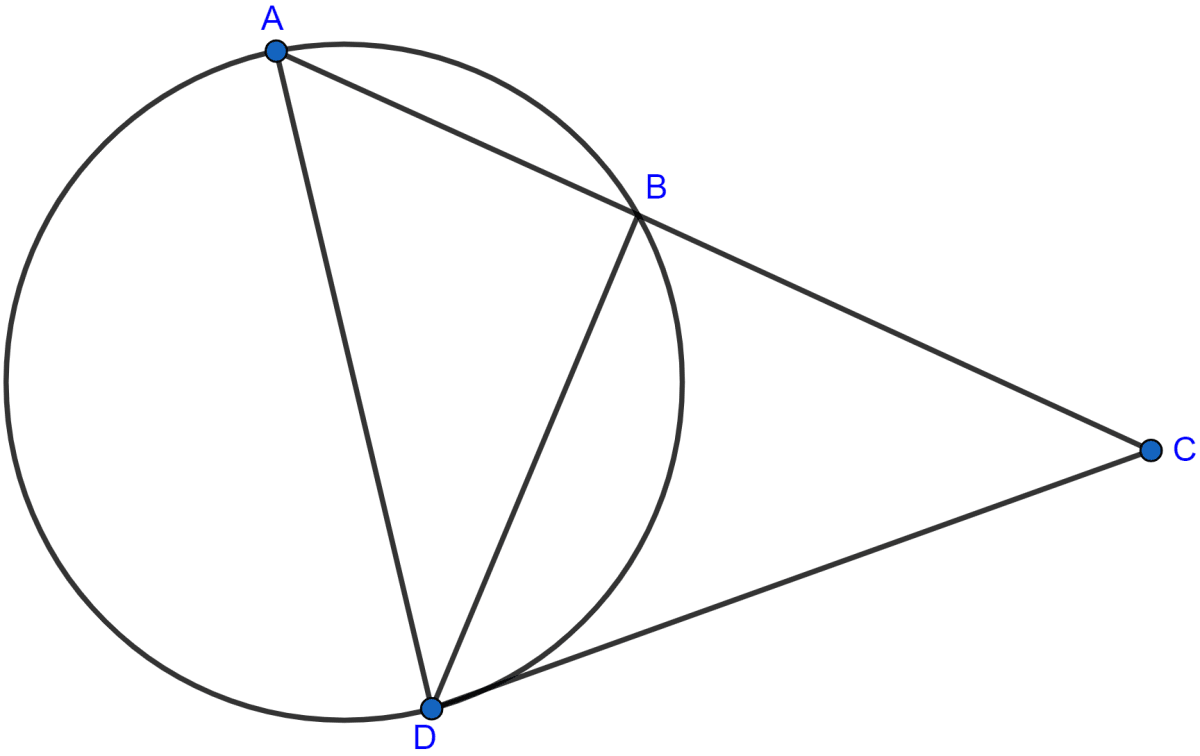
In the adjoining figure, CBA is a secant and CD is tangent to the circle. If AB = 7 cm and BC = 9 cm, then
(i) Prove that △ACD ~ △DCB
(ii) find the length of CD.
Circles
37 Likes
Answer
(i) In △ACD and △DCB
∠C = ∠C (Common angles)
∠CAD = ∠CDB (Angles in alternate segments are equal)
∴ △ACD ~ △DCB (By AA axiom.)
Hence, proved that △ACD ~ △DCB.
(ii) Since triangles are similar hence, the ratio of their corresponding sides are equal.
Hence, the length of DC = 12 cm.
Answered By
21 Likes
Related Questions
In the figure (ii) given below, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find :
(i) AB
(ii) the length of tangent PT.

PAB is a secant and PT is tangent to a circle. If
(i) PT = 8 cm and PA = 5 cm, find the length of AB.
(ii) PA = 4.5 cm and AB = 13.5 cm, find the length of PT.
In the figure (i) given below, PAB is a secant and PT is tangent to a circle. If PA : AB = 1 : 3 and PT = 6 cm, find the length of PB.

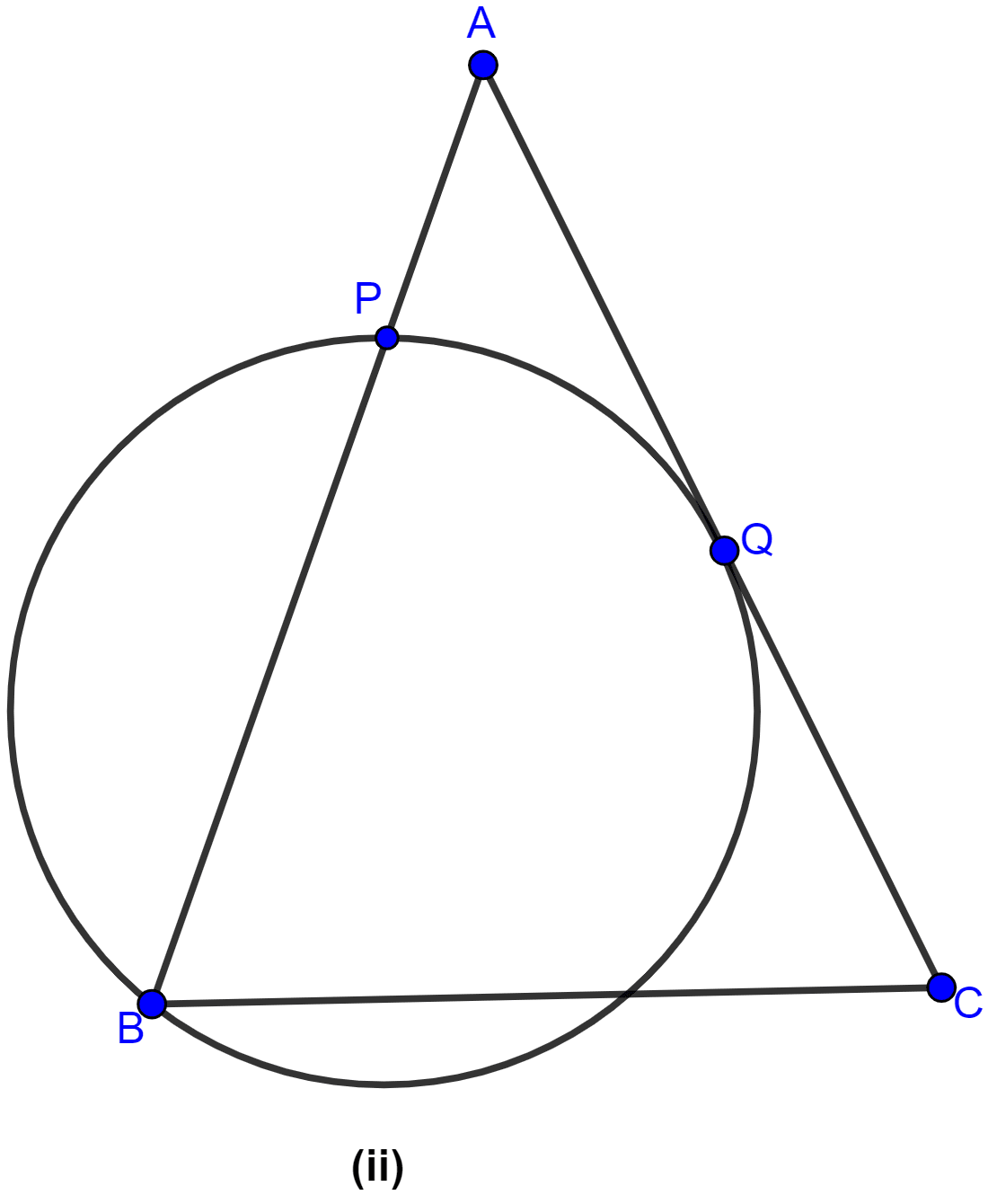
In the figure (ii) given below, ABC is an isosceles triangle in which AB = AC and Q is mid-point of AC. If APB is a secant and AC is tangent to the circle at Q, prove that AB = 4AP.