Mathematics
In △PQR, ∠Q = 90° and QM is perpendicular to PR. Prove that :
(i) PQ2 = PM × PR
(ii) QR2 = PR × MR
(iii) PQ2 + QR2 = PR2
Similarity
23 Likes
Answer
△PQR is shown in the figure below:
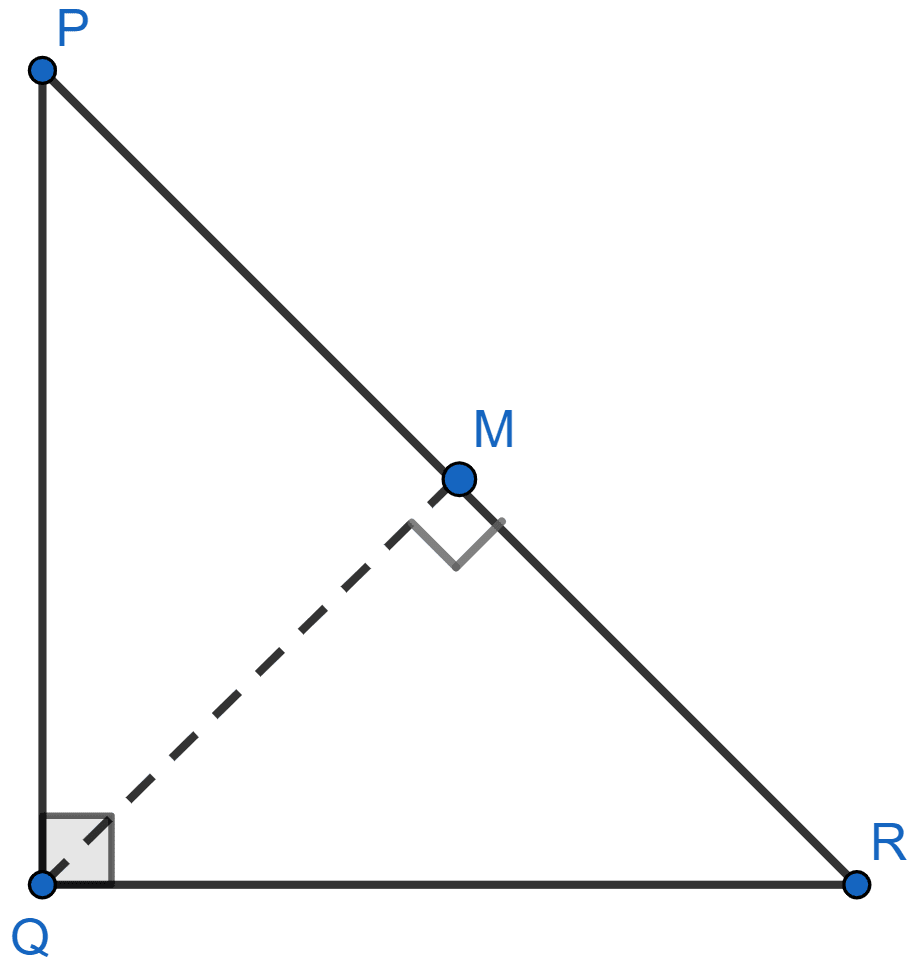
(i) In △PQR and △PMQ,
⇒ ∠PMQ = ∠PQR [Both = 90°]
⇒ ∠QPM = ∠RPQ [Common]
∴ △PQR ~ △PMQ [By AA]
Since, corresponding sides of similar triangles are proportional we have :
⇒
⇒ PQ2 = PM × PR
Hence, proved that PQ2 = PM × PR.
(ii) In △QRM and △PRQ,
⇒ ∠QMR = ∠PQR [Both = 90°]
⇒ ∠QRM = ∠QRP [Common]
∴ △QRM ~ △PRQ [By AA]
Since, corresponding sides of similar triangles are proportional we have :
⇒
⇒ QR2 = PR × MR
Hence, proved that QR2 = PR × MR.
(iii) Adding equations from (i) and (ii) we get,
⇒ PQ2 + QR2 = PM × PR + PR × MR ………(1)
⇒ PQ2 + QR2 = PR(PM + MR)
From figure,
PM + MR = PR
⇒ PQ2 + QR2 = PR2.
Hence, proved that PQ2 + QR2 = PR2.
Answered By
16 Likes
Related Questions
D is a point on the side BC of triangle ABC such that angle ADC is equal to angle BAC. Prove that: CA2 = CB x CD.
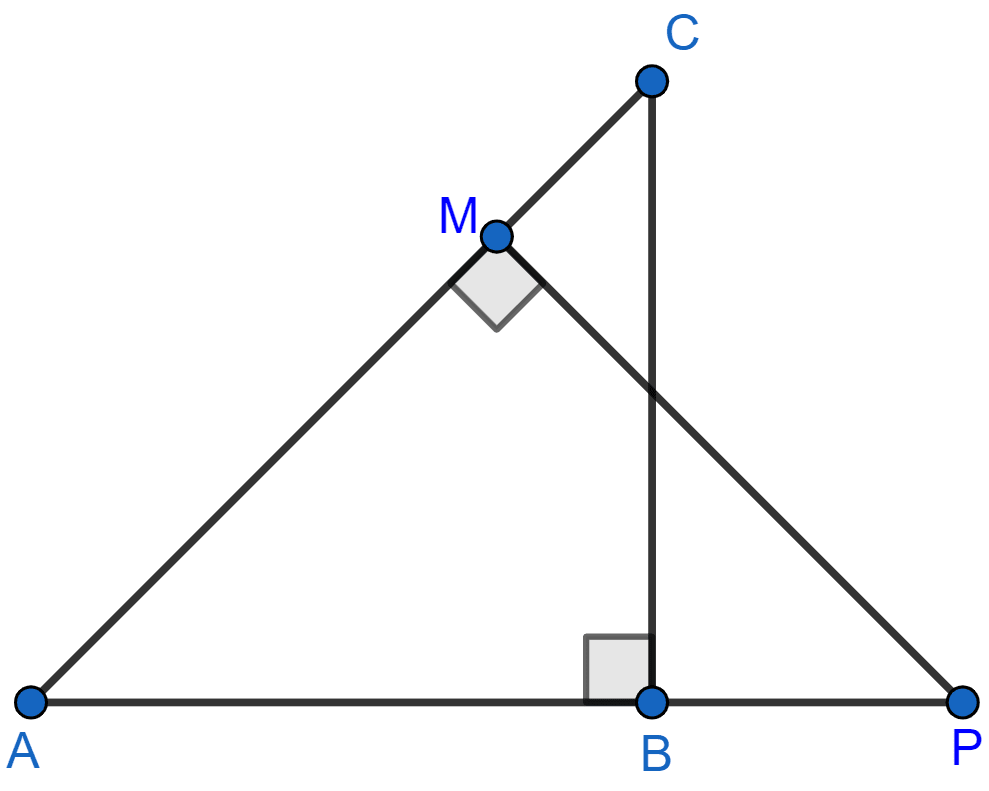
In the given figure, ΔABC and ΔAMP are right angled at B and M respectively.
Given AC = 10 cm, AP = 15 cm and PM = 12 cm.
(i) Prove that : ∆ABC ~ ∆AMP.
(ii) Find AB and BC.
In △ABC, ∠B = 90° and BD ⊥ AC.
(i) If CD = 10 cm and BD = 8 cm; find AD.
(ii) If AC = 18 cm and AD = 6 cm; find BD.
(iii) If AC = 9 cm and AB = 7 cm; find AD.
In the figure, PQRS is a parallelogram with PQ = 16 cm and QR = 10 cm, L is a point on PR such that RL : LP = 2 : 3. QL produced meets RS at M and PS produced at N. Find the lengths of PN and RM.