Mathematics
If the diagonals of a parallelogram are equal, then show that it is a rectangle.
Related Questions
Show that the diagonals of a square are equal and bisect each other at right angles.
Diagonal AC of a parallelogram ABCD bisects ∠A. Show that
(i) it bisects ∠C also,
(ii) ABCD is a rhombus.
(Ex.8.1Q3.png)
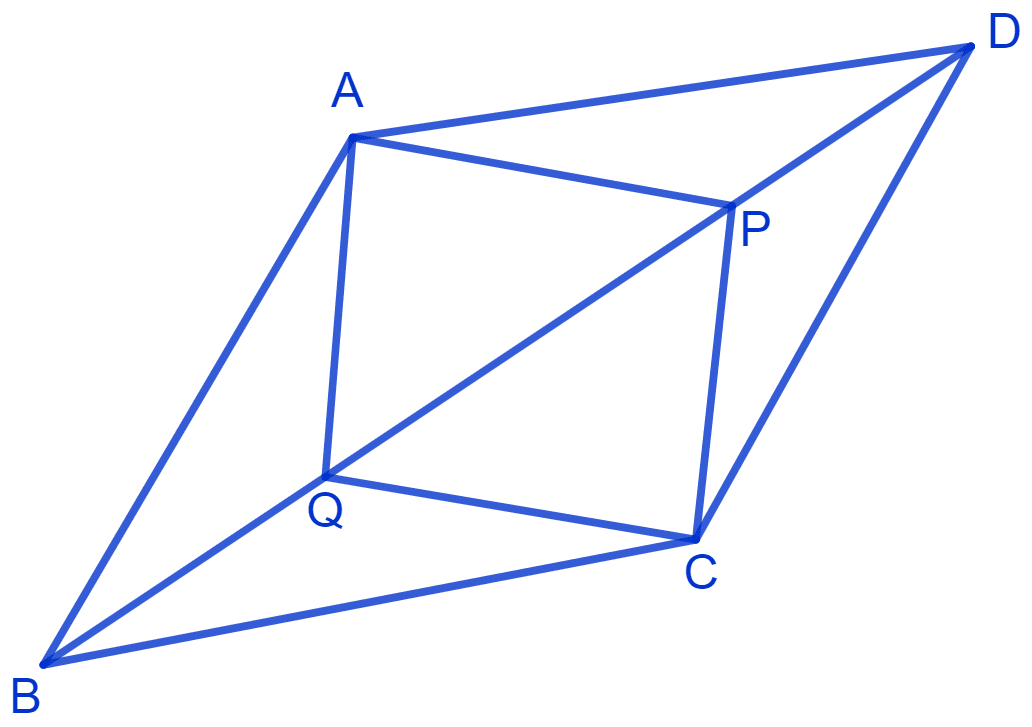
In parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP = BQ see Fig. Show that:
(i) Δ APD ≅ Δ CQB
(ii) AP = CQ
(iii) Δ AQB ≅ Δ CPD
(iv) AQ = CP
(v) APCQ is a parallelogram
ABCD is a rectangle in which diagonal AC bisects ∠A as well as ∠C. Show that :
(i) ABCD is a square
(ii) diagonal BD bisects ∠B as well as ∠D.