Mathematics
If the abscissa of a point P is 2, find the ratio in which it divides the line segment joining the points (-4, 3) and (6, 3). Hence, find the coordinates of P.
Section Formula
25 Likes
Answer
Let coordinates of A be (-4, 3) and of B be (6, 3) and of P be (2, y).
Let the ratio in which the P divides AB be m : n.
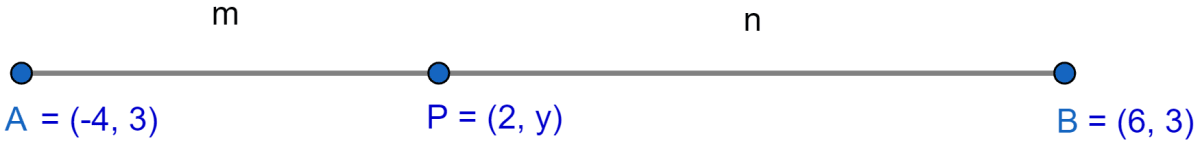
By section formula,
x-coordinate =
Similarly for y-coordinate,
Hence, the coordinates of P is (2, 3) and it divides the line in the ratio 3 : 2.
Answered By
9 Likes
Related Questions
Find the ratio in which the point P(-3, p) divides the line segment joining the points (-5, -4) and (-2, 3). Hence, find the value of p.
In what ratio is the line joining the points (4, 2) and (3, -5) divided by the x-axis? Also find the coordinates of the point of division.
Determine the ratio in which the line 2x + y - 4 = 0 divide the line segment joining the points A(2, -2) and B(3, 7). Also find the coordinates of the point of the division.
ABCD is a parallelogram. If the coordinates of A, B and D are (10, -6), (2, -6) and (4, -2) respectively, find the coordinates of C.