If P(2, -1), Q(3, 4), R(-2, 3) and S(-3, -2) be four points in a plane, show that PQRS is a rhombus but not a square. Find the area of the rhombus.

By distance formula,

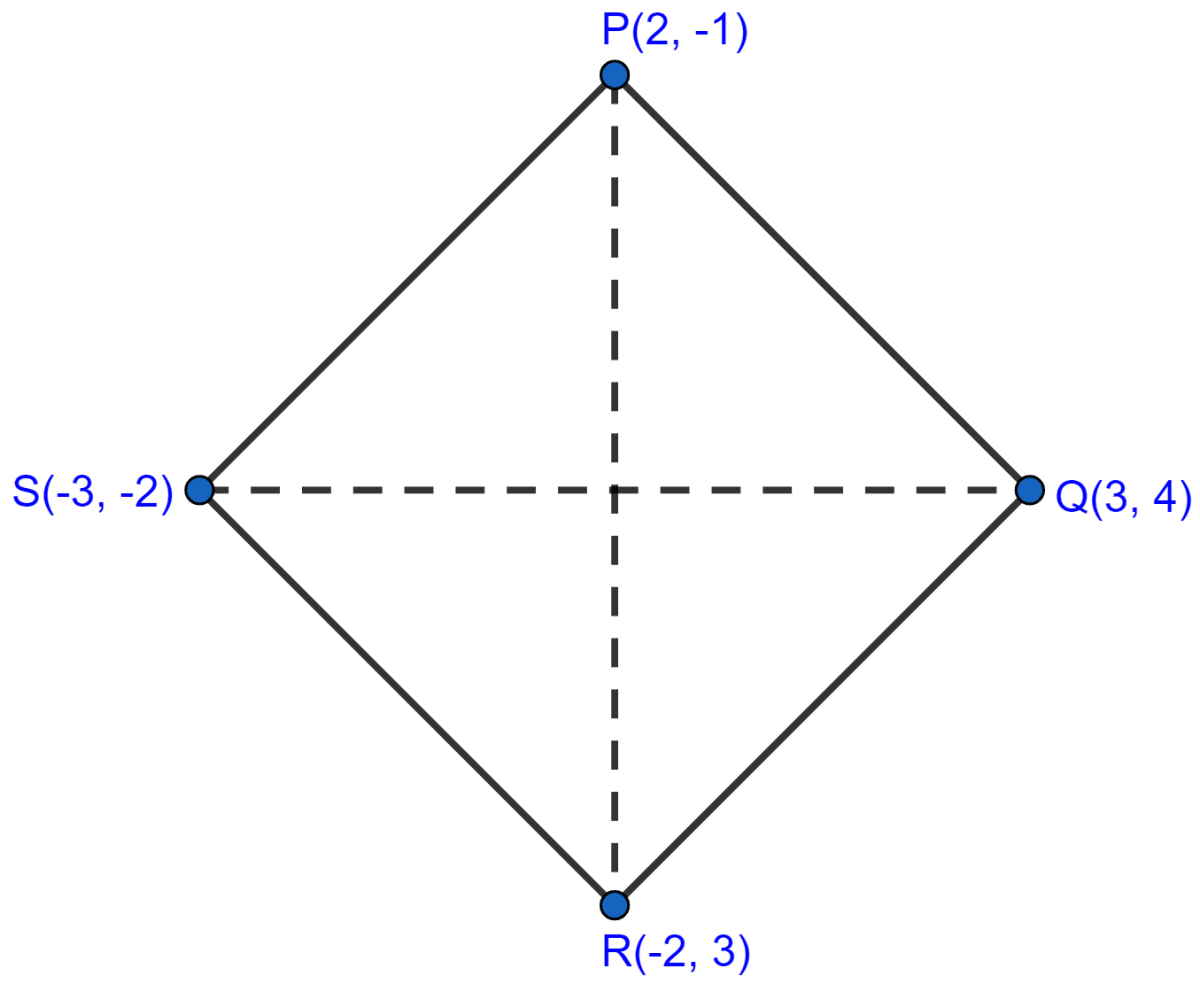
d = $\sqrt{(x$2 - x1)^2 + (y2 - y1)^2}

$PQ = \sqrt{(3 - 2)^2 + [4 - (-1)]^2} \\[1em] = \sqrt{1^2 + [4 + 1]^2} \\[1em] = \sqrt{1 + 5^2} \\[1em] = \sqrt{1 + 25} \\[1em] = \sqrt{26}. \\[1em] QR = \sqrt{(-2 - 3)^2 + (3 - 4)^2} \\[1em] = \sqrt{(-5)^2 + (-1)^2} \\[1em] = \sqrt{25 + 1} \\[1em] = \sqrt{26}. \\[1em] RS = \sqrt{[-3 - (-2)]^2 + [-2 - 3]^2} \\[1em] = \sqrt{[-3 + 2]^2 + [-5]^2} \\[1em] = \sqrt{[-1]^2 + 25} \\[1em] = \sqrt{26}. \\[1em] PS = \sqrt{[-3 - 2]^2 + [-2 - (-1)]^2} \\[1em] = \sqrt{[-5]^2 + [-2 + 1]^2} \\[1em] = \sqrt{25 + [-1]^2} \\[1em] = \sqrt{25 + 1} \\[1em] = \sqrt{26}.$

Here, PQ = QR = RS = PS.

So, PQRS can be a square or rhombus.

Calculating diagonal,

$PR = \sqrt{(-2 - 2)^2 + [3 - (-1)]^2} \\[1em] = \sqrt{(-4)^2 + [3 + 1]^2} \\[1em] = \sqrt{16 + 4^2} \\[1em] = \sqrt{16 + 16} \\[1em] = \sqrt{32} \\[1em] = 4\sqrt{2}. \\[1em] QS = \sqrt{(-3 - 3)^2 + (-2 - 4)^2} \\[1em] = \sqrt{(-6)^2 + (-6)^2} \\[1em] = \sqrt{36 + 36} \\[1em] = \sqrt{72} \\[1em] = 6\sqrt{2}.$

PR ≠ QS.

Since, diagonal of rhombus are not equal,

∴ PQRS is a rhombus

Area of rhombus = $\dfrac{1}{2} \times d$1 \times d2

= $\dfrac{1}{2} \times 4\sqrt{2} \times 6\sqrt{2}$

= $\dfrac{48}{2}$ = 24 sq. units.

Hence, PQRS is a rhombus and area = 24 sq. units.