Physics
(i) When a tuning fork [vibrating] is held close to ear, one hears a faint hum. The same [vibrating tuning fork] is held such that it's stem is in contact with the table surface, then one hears a loud sound. Explain.
(ii) A man standing in front of a vertical cliff fires a gun. He hears the echo after 3.5 seconds. On moving closer to the cliff by 84 m, he hears the echo after 3 seconds. Calculate the distance of the cliff from the initial position of the man.
Sound
ICSE 2020
Answer
(i) When the stem of the tuning fork is placed in contact with a table top, it sets the table surface in small vibrations but the large number of air molecules in contact with the table surface also start vibrating, thereby producing a loud sound.
(ii) Let the man be standing at distance d from the cliff and v be the speed of sound in air.
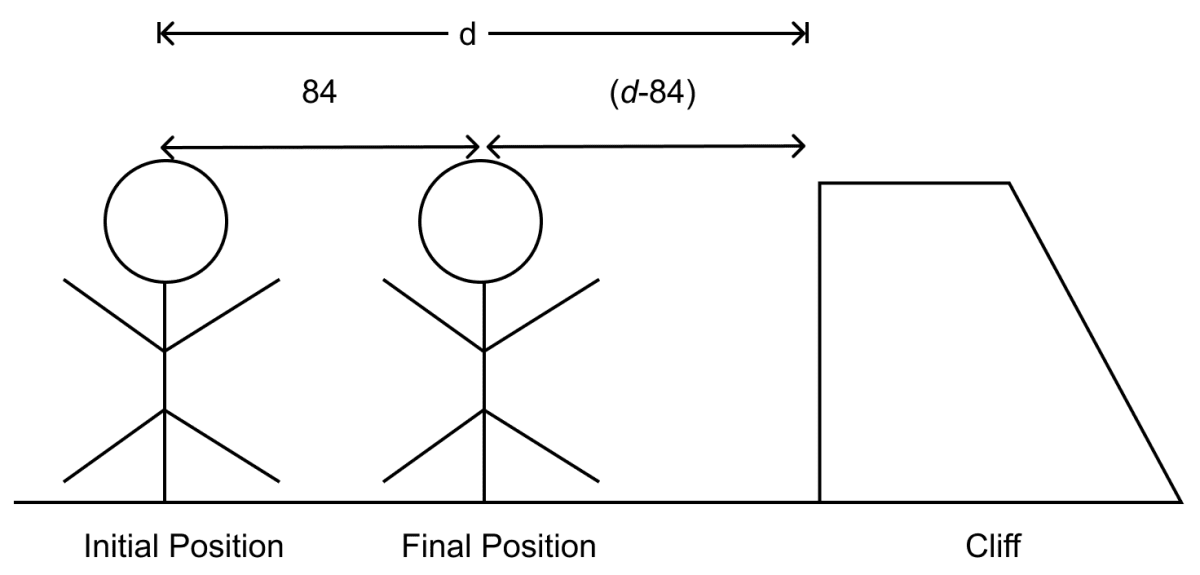
For first echo, t1 = = 3.5 s [Eqn i ]
and for second echo, t2 = = 3 s [Eqn ii]
Dividing eqn (ii) by eqn (i)
Hence, the distance of the cliff from the initial position of the man = 588 m
Answered By
Related Questions
Give reasons for the following:
During the day:
(i) Clouds appear white.
(ii) Sky appears blue.
(i) Name the system which enables us to locate underwater objects by transmitting ultrasonic waves and detecting the reflecting impulse.
(ii) What are acoustically measurable quantities related to pitch and loudness?
The diagram below shows the core of a transformer and it's input and output connections

(i) State the material used for the core.
(ii) Copy and complete the diagram of the transformer by drawing input and output coils.
(i) What are superconductors?
(ii) Calculate the current drawn by an appliance rated 110 W, 220 V when connected across 220 V supply.
(iii) Name a substance whose resistance decreases with the increase in temperature.