Given sin θ = $\dfrac{p}{q}$, find cos θ + sin θ in terms of p and q.

Let ABC be a right angle triangle with ∠C = θ and ∠B = 90°.

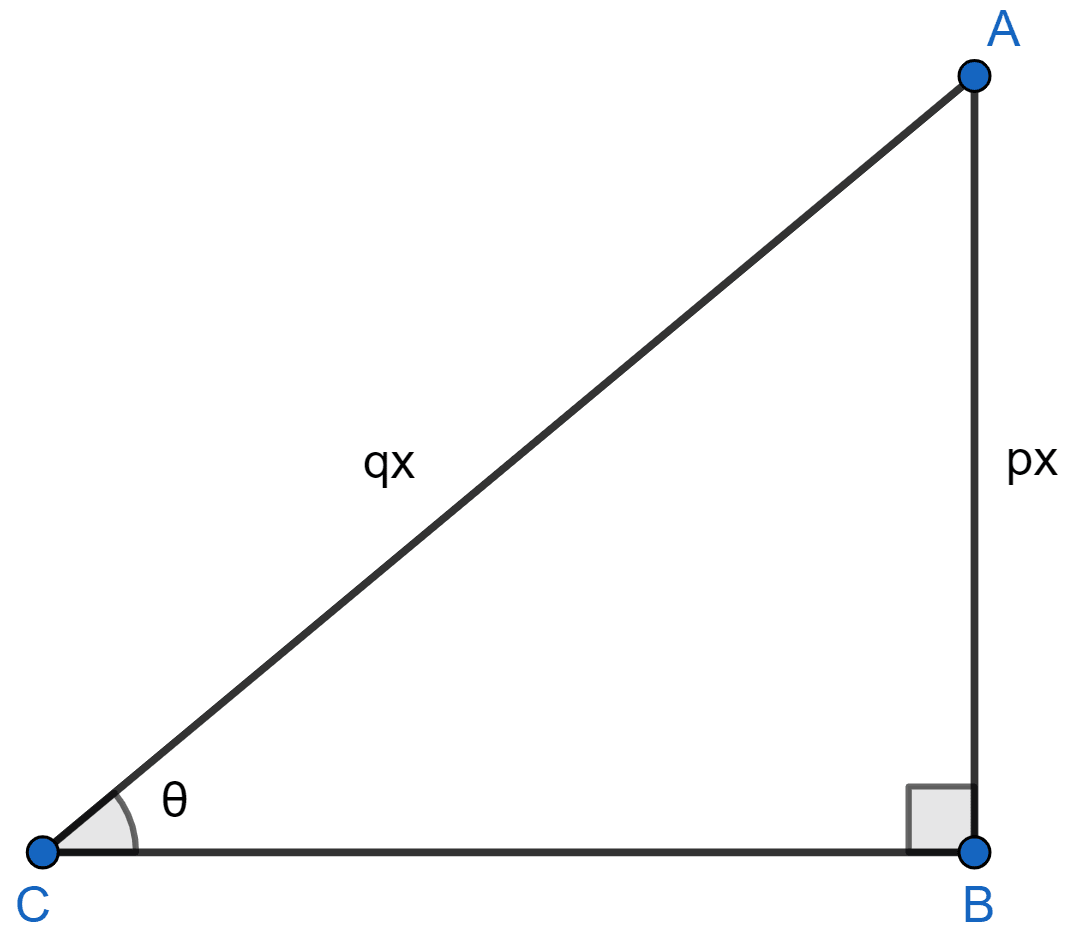
By formula,

sin θ = $\dfrac{\text{Perpendicular}}{\text{Hypotenuse}} = \dfrac{AB}{AC}$ ……..(1)

Given,

sin θ = $\dfrac{p}{q}$ ………(2)

From (1) and (2) we get,

$\dfrac{AB}{AC} = \dfrac{p}{q}$

Let AB = px and AC = qx.

In right angled triangle ABC,

By pythagoras theorem,

⇒ AC² = AB² + BC²

⇒ BC² = AC² - AB²

⇒ BC² = (qx)² - (px)²

⇒ BC² = q²x² - p²x²

⇒ BC² = x²(q² - p²)

⇒ BC = $\sqrt{x^2(q^2 - p^2)}$

⇒ BC = $x\sqrt{(q^2 - p^2)}$

In right angled triangle ABC,

By formula,

cos θ = $\dfrac{\text{Base}}{\text{Hypotenuse}}$

= $\dfrac{BC}{AC} = \dfrac{x\sqrt{q^2 - p^2}}{qx} = \dfrac{\sqrt{q^2 - p^2}}{q}$.

Substituting values in cos θ + sin θ we get,

$\Rightarrow \text{cos θ + sin θ} = \dfrac{\sqrt{q^2 - p^2}}{q} + \dfrac{p}{q} \\[1em] = \dfrac{p + \sqrt{q^2 - p^2}}{q}.$

Hence, cos θ + sin θ = $\dfrac{p + \sqrt{q^2 - p^2}}{q}.$