Mathematics
From the top of a cliff, the angles of depression of the top and bottom of a tower are observed to be 45° and 60° respectively. If the height of the tower is 20 m, find :
(i) the height of the cliff.
(ii) the distance between the cliff and the tower.
Heights & Distances
177 Likes
Answer
(i) Let AB be the cliff and CD be the tower.
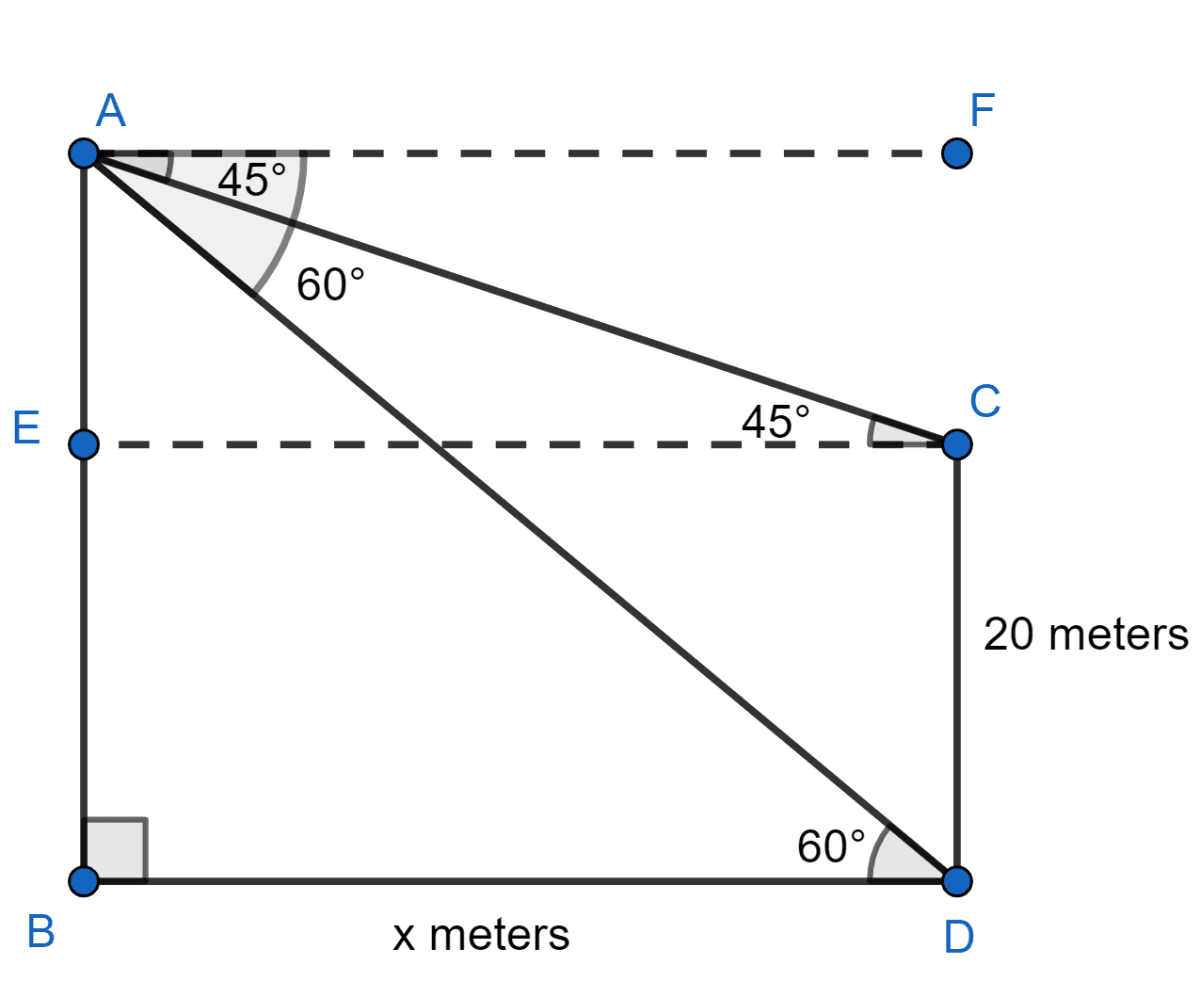
From figure,
∠ACE = ∠FAC = 45° (Alternate angles are equal)
∠ADB = ∠FAD = 60° (Alternate angles are equal)
Let BD = x.
From figure,
EC = BD = x meters.
EB = CD = 20 meters.
In △ AEC,
⇒ tan 45° =
⇒ 1 =
⇒ AE = x meters.
In △ ABD,
From figure,
Height of cliff (AB) = AE + EB
= x + 20
= 27.32 + 20
= 47.32 meters.
Hence, the height of cliff = 47.32 meters.
(ii) From figure,
Distance between cliff and tower (BD) = x meters = 27.32 meters.
Hence, distance between cliff and tower = 27.32 meters.
Answered By
49 Likes
Related Questions
A trader buys x identical articles for ₹ 600. If the cost per article were ₹ 5 more, the number of articles that can be bought for ₹ 600 would be four less. Find the value of x.
The marks obtained by 120 students in a Mathematics test are given below :
Marks No. of students 0-10 5 10-20 9 20-30 16 30-40 22 40-50 26 50-60 18 60-70 11 70-80 6 80-90 4 90-100 3 Using the informations, given above draw an ogive on a graph sheet. Take a suitable scale for your ogive. Use the ogive to drawn to estimate :
(i) the median marks.
(ii) the number of students who obtained more than 75% marks in the test.
(iii) the number of students who did not pass in the test if the pass percentage was 40.
If x = , prove that : x2 - 4ax + 1 = 0.
Construct a cyclic quadrilateral ABCD in which AC = 4.5 cm, ∠ABC = 45°, AB = 3 cm and AD = 2.3 cm.