Mathematics
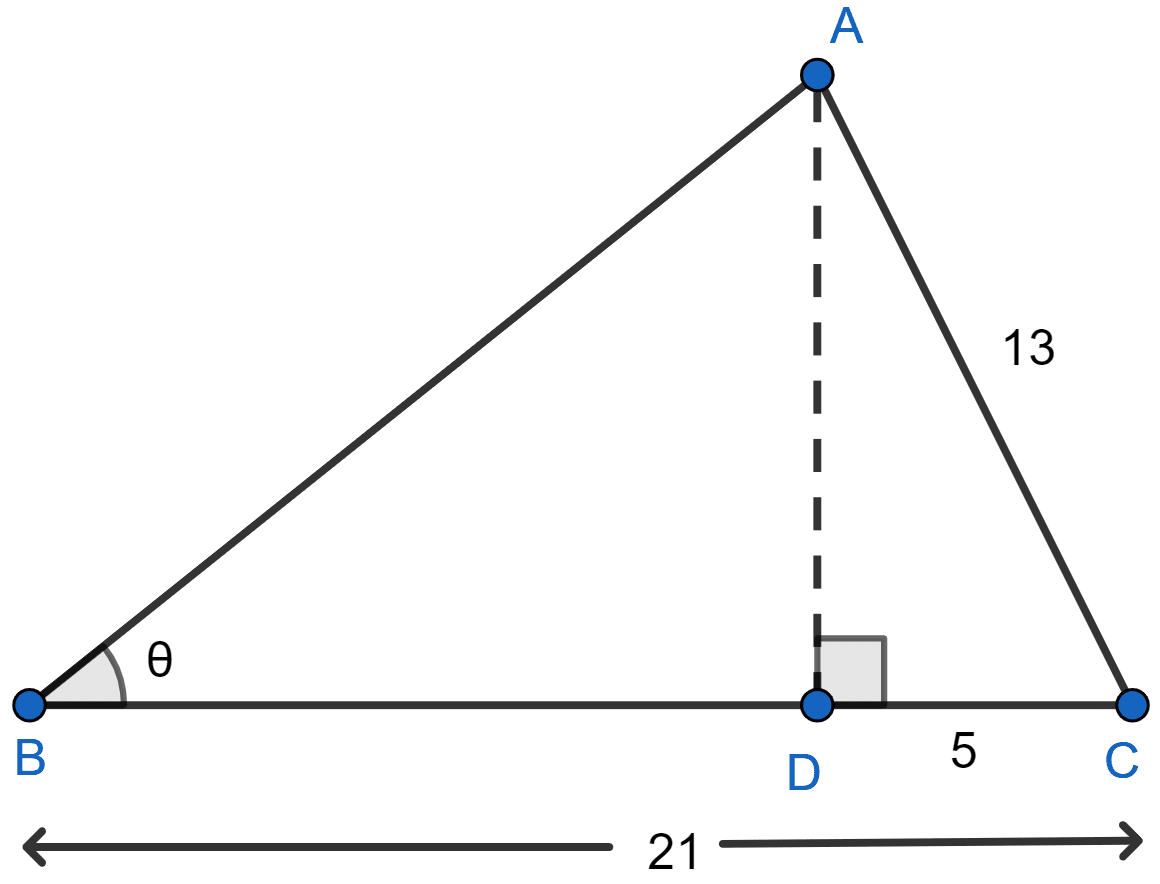
From the figure (1) given below, find the values of:
(i) sin θ
(ii) cos θ
(iii) tan θ
(iv) cot θ
(v) sec θ
(vi) cosec θ
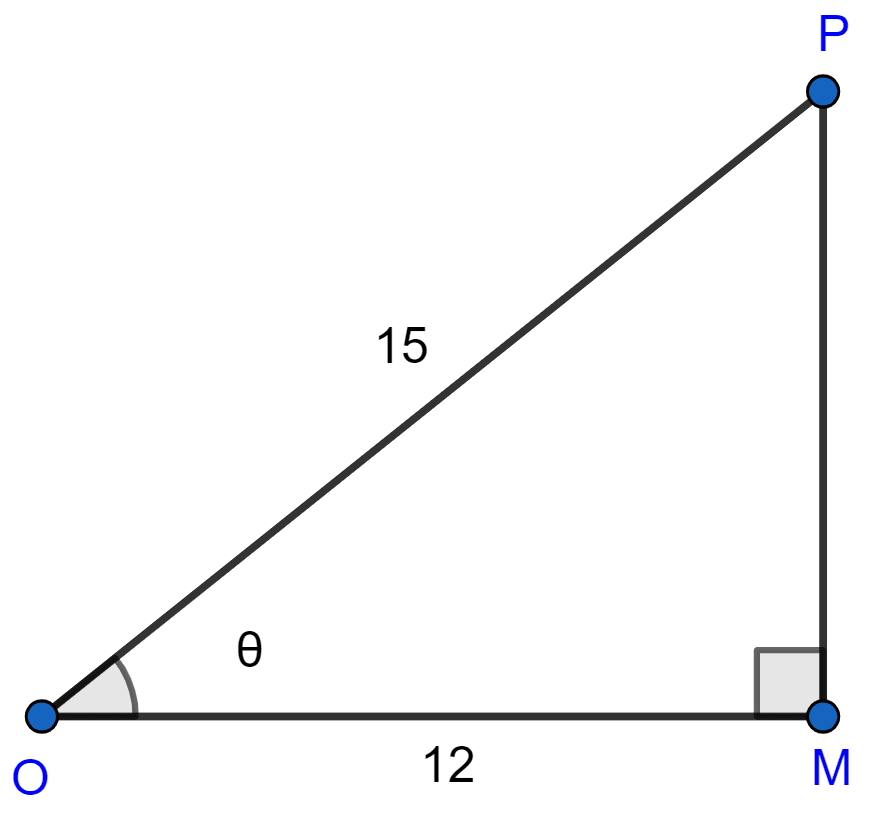
Trigonometrical Ratios
69 Likes
Answer
In right-angled triangle OMP,
By Pythagoras theorem, we get
⇒ OP2 = OM2 + MP2
⇒ MP2 = OP2 - OM2
⇒ MP2 = (15)2 - (12)2
⇒ MP2 = 225 - 144
⇒ MP2 = 81
⇒ MP = = 9.
(i) sin θ =
= .
Hence, sin θ = .
(ii) cos θ =
= .
Hence, cos θ = .
(iii) tan θ =
= .
Hence, tan θ = .
(iv) cot θ =
= .
Hence, cot θ = .
(v) sec θ =
= .
Hence, sec θ = .
(vi) cosec θ =
= .
Hence, cosec θ = .
Answered By
38 Likes
Related Questions
From the figure (2) given below, find the values of :
(i) sin A
(ii) cos A
(iii) sin2 A + cos2 A
(iv) sec2 A - tan2 A

From the figure (1) given below, find the values of:
(i) sin B
(ii) cos C
(iii) sin B + sin C
(iv) sin B cos C + sin C cos B.

From the figure (2) given below, find the values of :
(i) tan x
(ii) cos y
(iii) cosec2 y - cot2 y
(iv) .
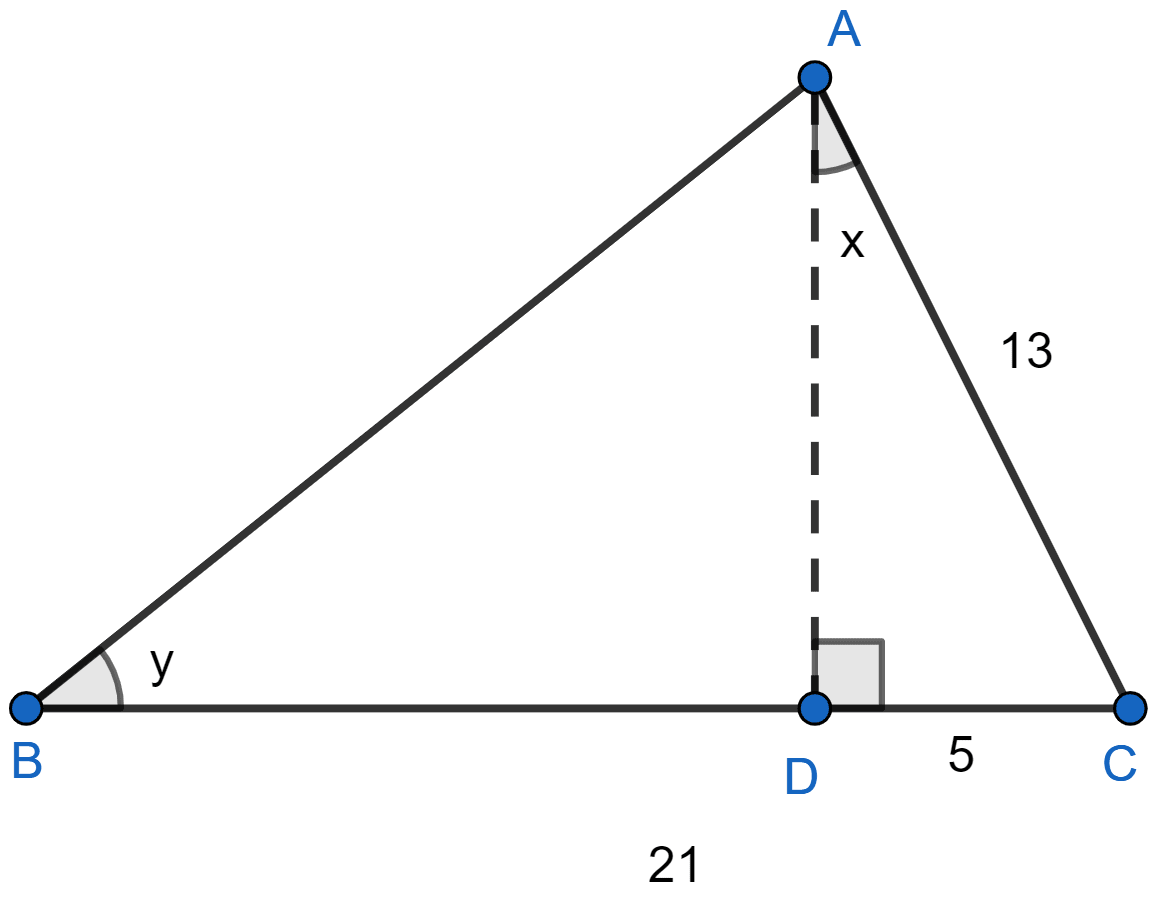
From the figure (1) given below, find the value of sec θ.