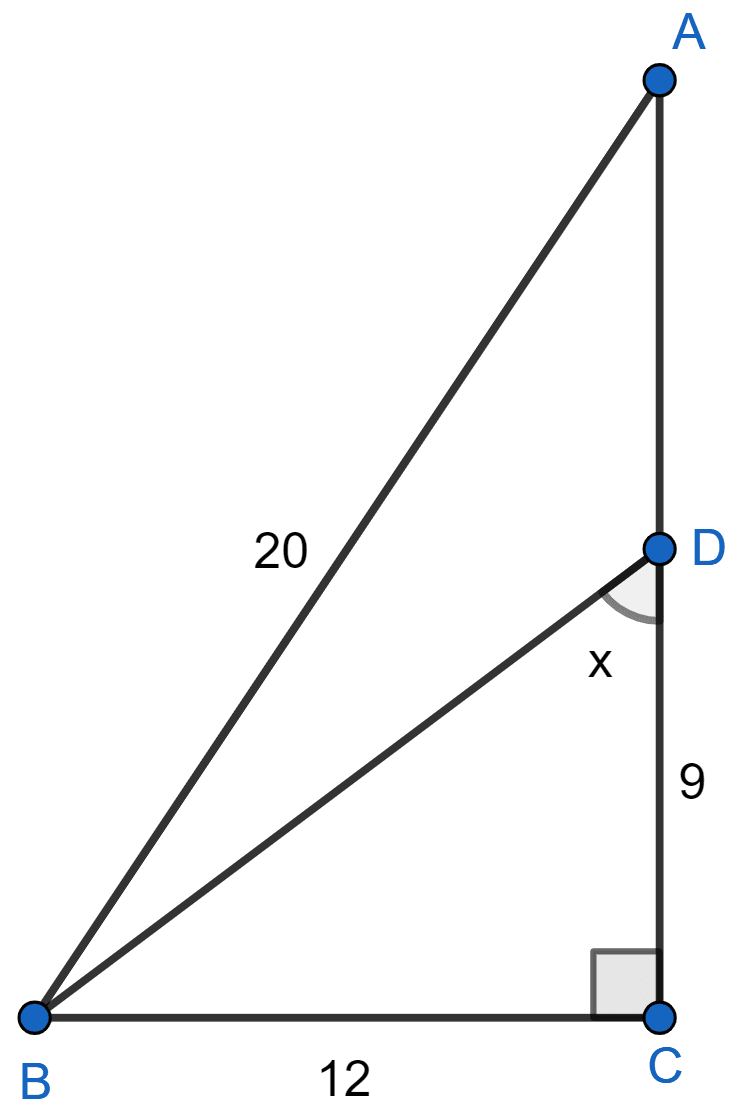
From the figure (1) given below, find the values of :

(i) sin ∠ABC

(ii) tan x - cos x + 3 sin x

(i) In right angle triangle ABC,

By pythagoras theorem we get :

⇒ AB² = AC² + BC²

⇒ 20² = AC² + 12²

⇒ 400 = AC² + 144

⇒ AC² = 400 - 144

⇒ AC² = 256

⇒ AC = $\sqrt{256}$ = 16.

$\text{sin ∠ABC} = \dfrac{\text{Perpendicular}}{\text{\text{Hypotenuse}}} \\[1em] = \dfrac{AC}{AB} \\[1em] = \dfrac{16}{20} = \dfrac{4}{5}.$

Hence, sin ∠ABC = $\dfrac{4}{5}$.

(ii) In right angle triangle BCD,

By pythagoras theorem we get :

⇒ BD² = BC² + CD²

⇒ BD² = 12² + 9²

⇒ BD² = 144 + 81

⇒ BD² = 225

⇒ BD = $\sqrt{225}$

⇒ BD = 15.

By formula,

$\text{tan x } = \dfrac{\text{Perpendicular}}{\text{Base}} \\[1em] = \dfrac{BC}{CD} = \dfrac{12}{9} = \dfrac{4}{3}. \\[1em] \text{cos x } = \dfrac{\text{Base}}{\text{Hypotenuse}} \\[1em] = \dfrac{CD}{BD} = \dfrac{9}{15} = \dfrac{3}{5}. \\[1em] \text{sin x } = \dfrac{\text{Perpendicular}}{\text{Hypotenuse}} \\[1em] = \dfrac{BC}{BD} = \dfrac{12}{15} = \dfrac{4}{5}. \\[1em]$

Substituting values in tan x - cos x + 3 sin x we get :

$\Rightarrow \dfrac{4}{3} - \dfrac{3}{5} + 3 \times \dfrac{4}{5} \\[1em] \Rightarrow \dfrac{4}{3} - \dfrac{3}{5} + \dfrac{12}{5} \\[1em] \Rightarrow \dfrac{20 - 9 + 36}{15} \\[1em] \Rightarrow \dfrac{47}{15} \\[1em] \Rightarrow 3\dfrac{2}{15}.$

Hence, tan x - cos x + 3 sin x = $3\dfrac{2}{15}.$