Mathematics
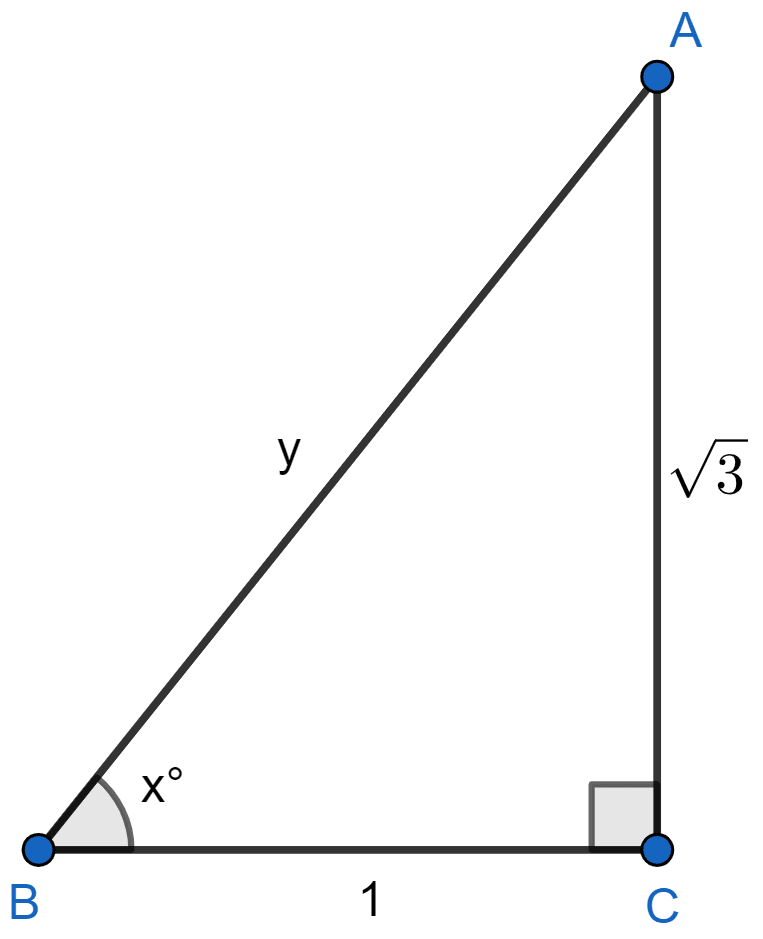
From the adjoining figure, find
(i) tan x°
(ii) x
(iii) cos x°
(iv) use sin x° to find y.
Trigonometrical Ratios
46 Likes
Answer
(i) By formula,
tan x° = .
Hence, tan x° = .
(ii) tan x° =
⇒ tan x° = tan 60°
⇒ x = 60.
Hence, x = 60.
(iii) Substituting value of x in cos x°, we get :
⇒ cos x° = cos 60°
⇒ cos x° = .
Hence, cos x° = .
(iv) Substituting value of x in sin x°, we get :
⇒ sin x° = sin 60° = .
By formula,
Hence, y = 2.
Answered By
31 Likes
Related Questions
If 3 sin2 θ = and θ is less than 90°, find the value of θ.
If θ is an acute angle and sin θ = cos θ, find the value of θ and hence, find the value of 2 tan2 θ + sin2 θ - 1.
If 3θ is an acute angle, solve the following equations for θ :
(i) 2 sin 3θ =
(ii) tan 3θ = 1.
If tan 3x = sin 45° cos 45° + sin 30°, find the value of x.