Mathematics
For a regular hexagon inscribed in a circle, the radius of the circle and the length of a side of the hexagon are :
equal
not equal
equal, if hexagon is regular
not equal, if hexagon is regular.
Constructions
3 Likes
Answer
We know that,
A regular hexagon comprises 6 equilateral triangles.
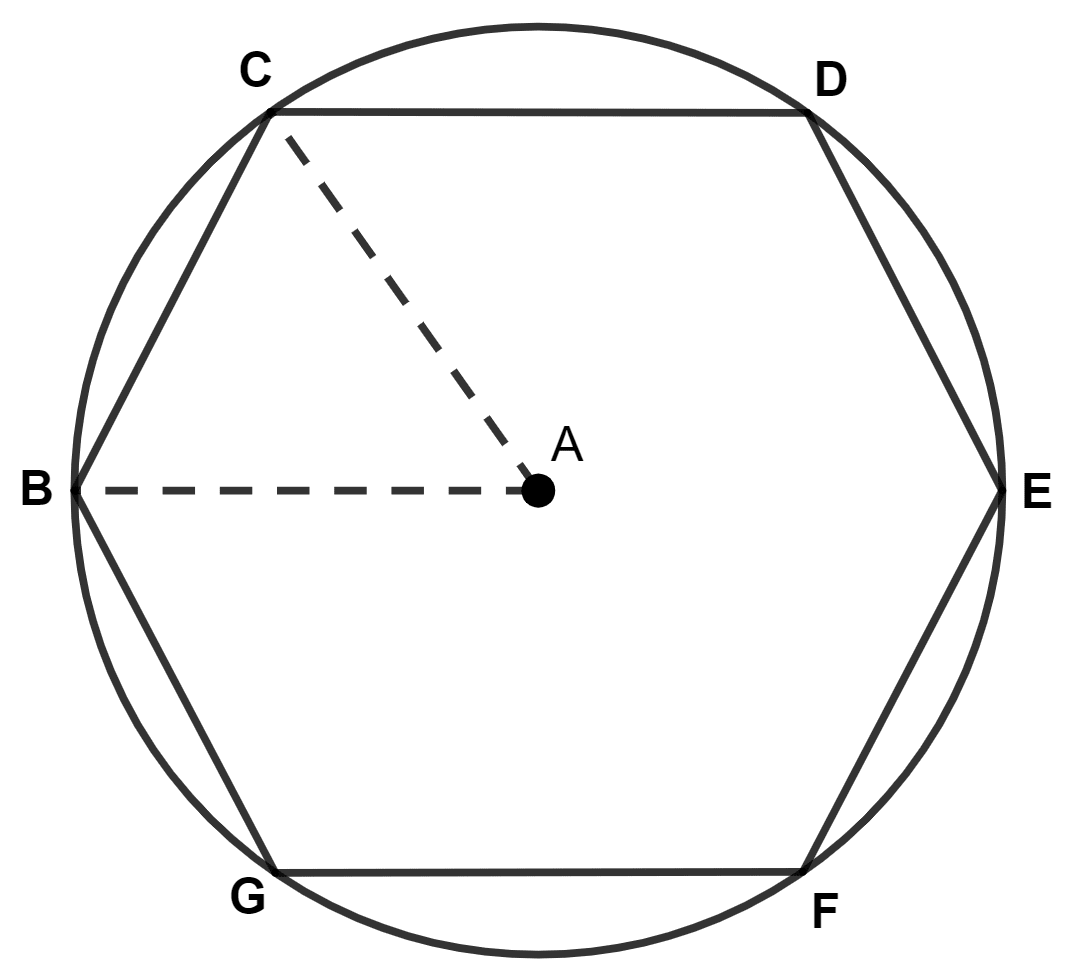
∴ BAC is an equilateral triangle.
∴ AB = BC = CA.
From figure,
AB and AC are radius of the circle.
⇒ AB = BC.
⇒ Radius = Side of hexagon
Hence, Option 1 is the correct option.
Answered By
3 Likes
Related Questions
Incenter of a triangle is the point of intersection of the :
perpendicular bisector of its sides
bisectors of its angles
one perpendicular of its side and bisector of any one angle of it
none of these.
For a regular hexagon, inscribing a circle, the length of the side of the hexagon and the radius of the circle are :
equal
not equal
side of hexagon is bigger than the radius of the circle
side of hexagon is smaller than the radius of the circle.
A regular octagon is circumscribing a circle, the angle subtended by each side of the regular octagon at the center of the circle is :
60°
30°
45°
(2 × 8 - 4) × 90°
O is center of the the circle and ∠AOB = 60°. The length of chord AB is :
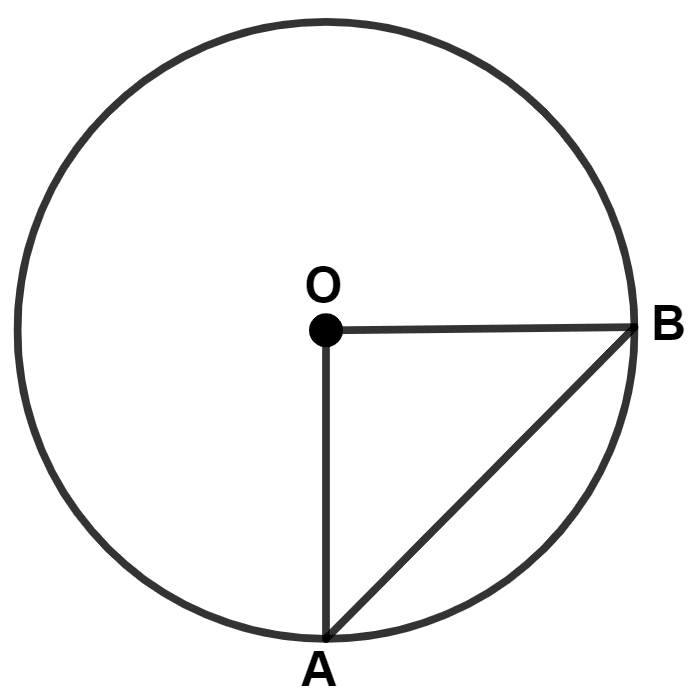
equal to radius of the circle
equal to the side of a regular pentagon
bigger than the radius of the circle
smaller than the radius of the circle.