Mathematics
Find the value of x which satisfies the inequation :
-2 ≤ , x ∈ W.
Also, graph the solution on a number line.
Linear Inequations
1 Like
Answer
Solving L.H.S. of the above inequation :
Solving R.H.S. of the above inequation :
From (1) and (2), we get :
-2 ≤ x ≤
Since, x ∈ W.
x = {0, 1, 2, 3}
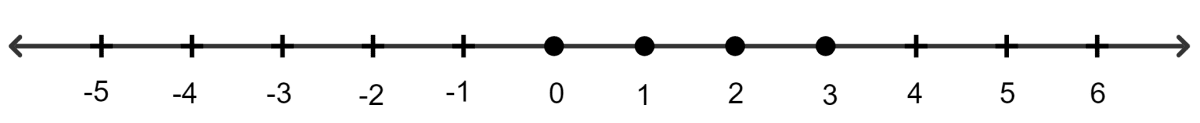
Hence, x = {0, 1, 2, 3}.
Answered By
2 Likes
Related Questions
Rajat invested ₹ 24,000 in 7% hundred rupee shares at 20% discount. After one year, he sold these shares at ₹ 75 each and invested the proceeds (including dividend of first year) in 18% twenty five rupee shares at 64% premium. Find :
(i) his gain or loss after one year.
(ii) his annual income from the second investment.
(iii) the percentage of increase in return on his original investment.
Solve (using formula) the equation :
.
By selling an article for ₹ 24, a man gains as much percent as its cost price. Find the cost price of the article.
A man sold some ₹ 20 shares, paying 8% dividend, at 10% discount and invested the proceeds in ₹ 10 shares, paying 12% dividend, at 50% premium. If the change in his annual income is ₹ 600, find the number of shares sold by the man.