Mathematics
Find the radius of a circle if a 90° arc has a length of 3.5π cm. Hence, find the area of the sector formed by this arc.
Mensuration
8 Likes
Answer
From figure,
AOB is a quadrant, with ∠AOB = 90°.
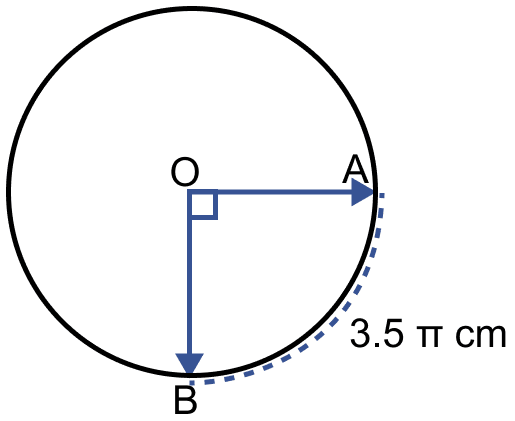
Let radius of circle be r cm,
Circumference of quadrant = .
Given,
Circumference of quadrant = 3.5π
Area of sector =
Hence, radius = 7 cm and area of sector = 38.5 cm2.
Answered By
6 Likes
Related Questions
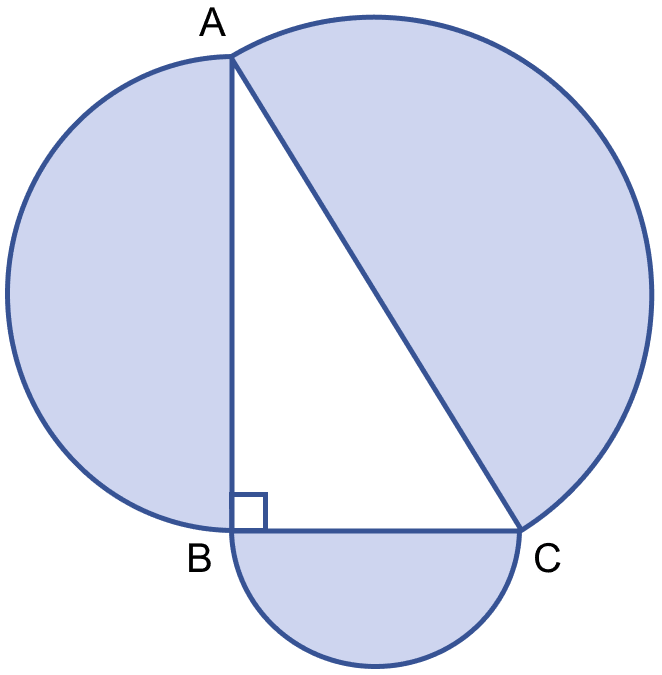
In the adjoining figure, ABC is a right angled triangle right angled at B. Semicircles are drawn on AB, BC and CA as diameter. Show that the sum of areas of semicircles drawn on AB and BC as diameter is equal to the area of the semicircle drawn on CA as diameter.
The length of minute hand of a clock is 14 cm. Find the area swept by the minute hand in 15 minutes.
A cube whose each edge is 28 cm long has a circle of maximum radius on each of its face painted red. Find the total area of the unpainted surface of the cube.
Can a pole 6.5 m long fit into the body of a truck with internal dimensions of 3.5 m, 3 m and 4 m?