Find the image of the point (1, 2) in the line x - 2y - 7 = 0.

The given line is x - 2y - 7 = 0 …..(i)

⇒ 2y = x - 7

⇒ y = $\dfrac{1}{2}x - \dfrac{7}{2}$.

The slope of the line (i) = m₁ = $\dfrac{1}{2}.$

Let the point (1, 2) be P.

From P draw a perpendicular to the line (i) and produce it to point P' such that P'M = MP, then P' is the image of P in line (i) and line (i) is the right bisector of the segment PP'.

Let P' be (a, b).

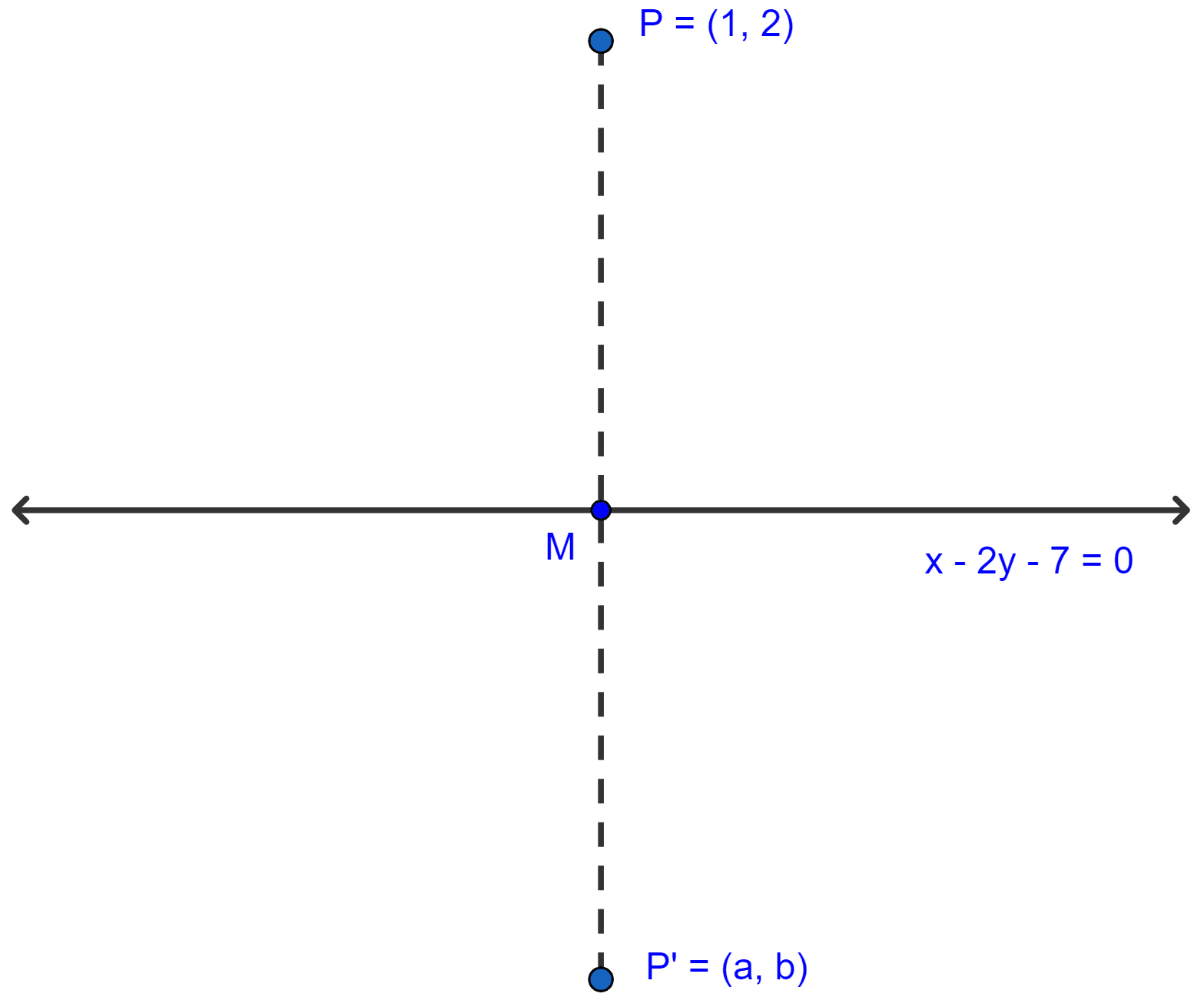
Then slope of PP' = m₂ = $\dfrac{b - 2}{a - 1}$.

Since, line (i) is perpendicular to PP' so,

$\Rightarrow m$1 \times m2 = -1 \\[1em] \Rightarrow \dfrac{1}{2} \times \dfrac{b - 2}{a - 1} = -1 \\[1em] \Rightarrow \dfrac{b - 2}{2a - 2} = -1 \\[1em] \Rightarrow b - 2 = -2a + 2 \\[1em] \Rightarrow 2a + b = 4 \space …..(\text{iii})

Also mid-point of PP' is M$\Big(\dfrac{a + 1}{2}, \dfrac{b + 2}{2}\Big)$.

Since, (i) is the right bisector of the segment PP', M lies on (i)

$\Rightarrow \dfrac{a + 1}{2} - 2\Big(\dfrac{b + 2}{2}\Big) - 7 = 0 \\[1em] \Rightarrow \dfrac{a + 1}{2} - b - 2 - 7 = 0 \\[1em] \Rightarrow \dfrac{a + 1}{2} - b = 9 \\[1em] \Rightarrow \dfrac{a + 1 - 2b}{2} = 9 \\[1em] \Rightarrow a + 1 - 2b = 18 \\[1em] \Rightarrow a - 2b = 17 \space ….(\text{iv})$

Multiplying equation (iv) by 2 and subtracting from (iii) we get,

$\Rightarrow 2a + b - 2(a - 2b) = 4 - 34 \\[1em] \Rightarrow 2a + b - 2a + 4b = -30 \\[1em] \Rightarrow 5b = -30 \\[1em] \Rightarrow b = -6.$

Putting value of b in Eq (iii),

⇒ 2a - 6 = 4
⇒ 2a = 10
⇒ a = 5.

P' = (a, b) = (5, -6).

Hence, the coordinates of image are (5, -6).