E and F are points on the sides PQ and PR respectively of a △PQR. For each of the following cases, state whether EF || QR :

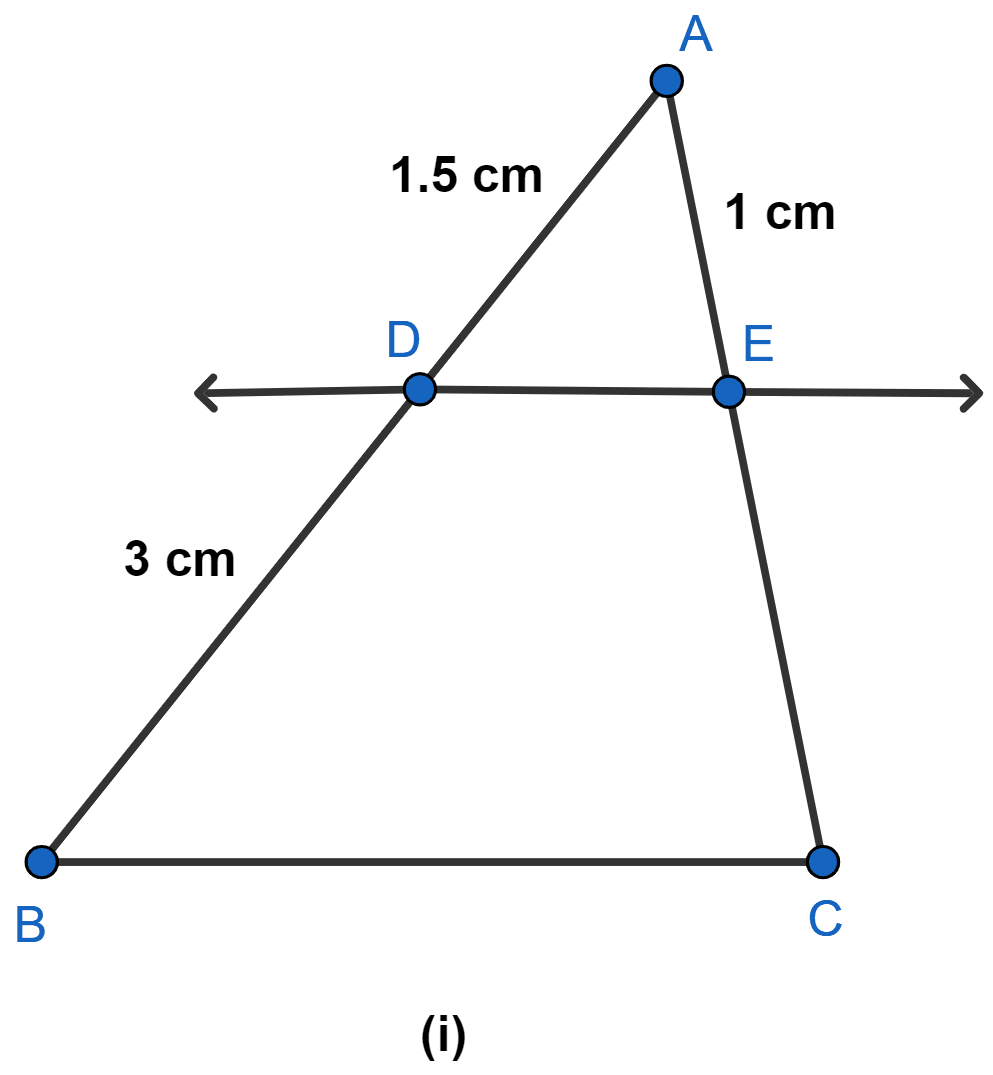
(i) PE = 3.9 cm, EQ = 3 cm, PF = 3.6 cm and FR = 2.4 cm

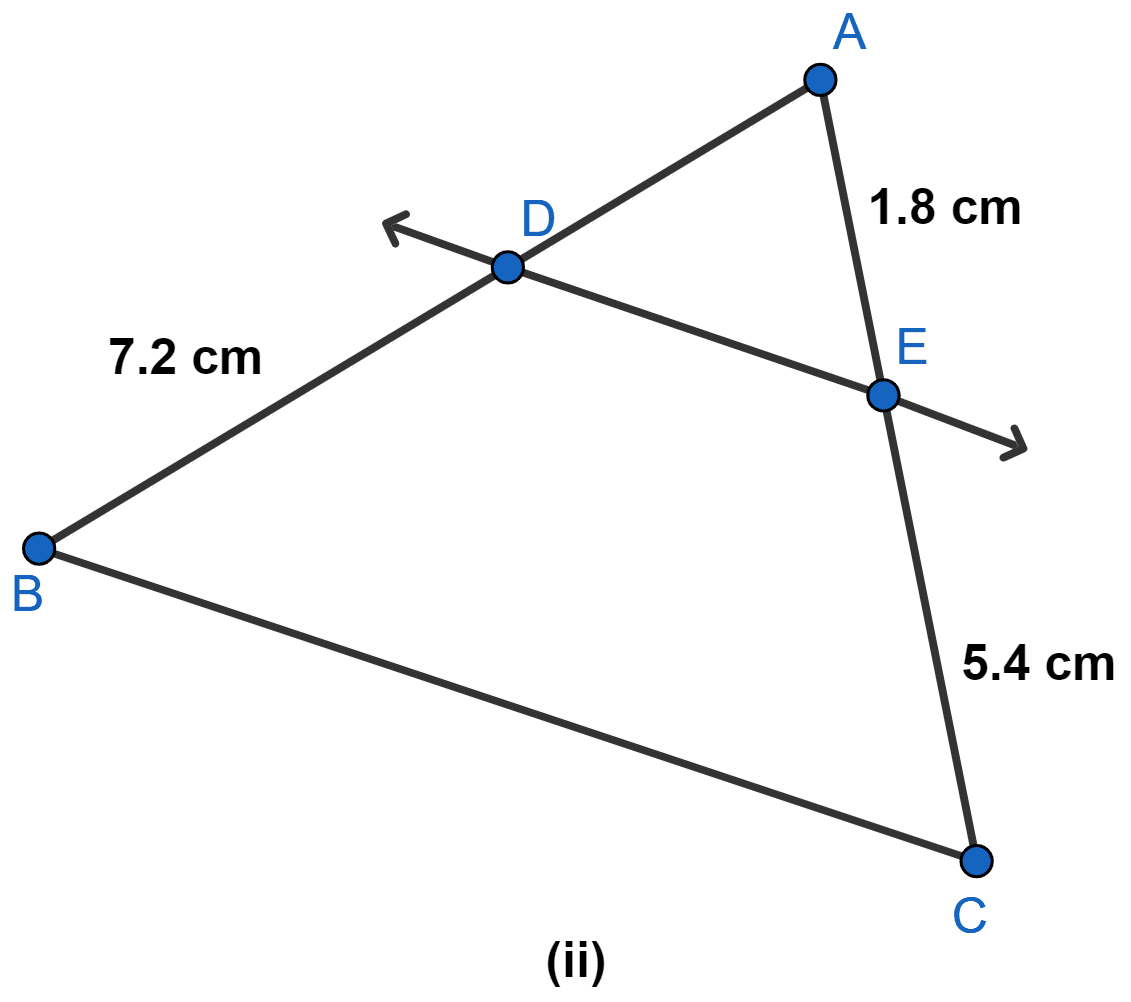
(ii) PE = 4 cm, QE = 4.5 cm, PF = 8 cm and RF = 9 cm

(iii) PQ = 1.28 cm, PR = 2.56 cm, PE = 0.18 cm and PF = 0.36 cm

We know that,

If a line divides any two sides of a triangle in the same ratio, then the line is parallel to the third side.

∴ EF || QR if

$\dfrac{PE}{EQ} = \dfrac{PF}{FR}$ ………..(1)

(i) Given,

PE = 3.9 cm, EQ = 3 cm, PF = 3.6 cm and FR = 2.4 cm.

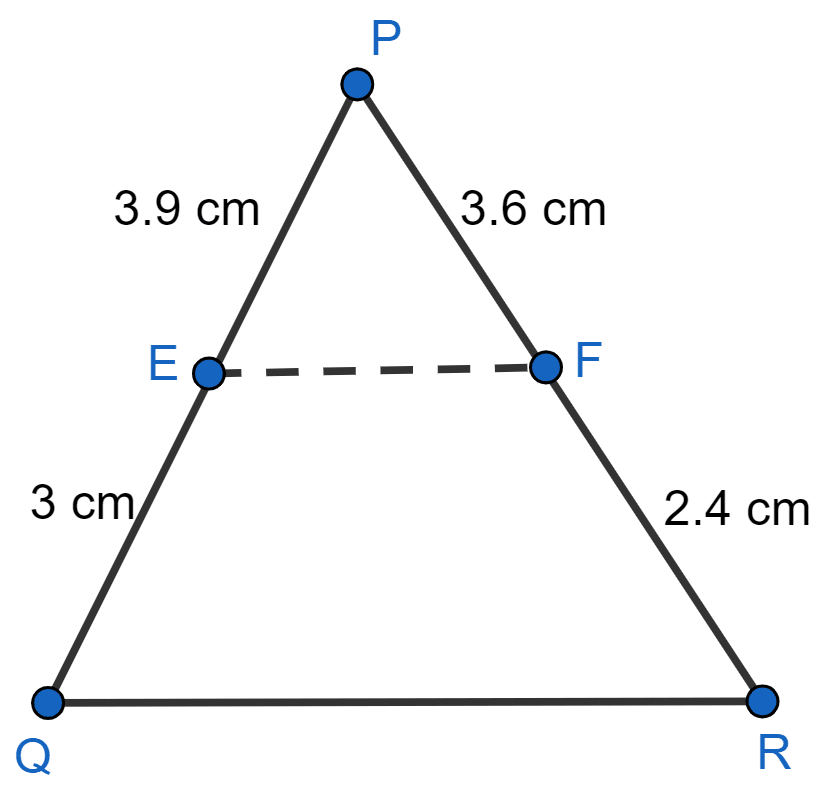
Substituting values in L.H.S. of equation (1), we get :

$\Rightarrow \dfrac{PE}{EQ} = \dfrac{3.9}{3} \\[1em] = \dfrac{1.3}{1} \\[1em] = \dfrac{13}{10}.$

Substituting values in R.H.S. of equation (1), we get :

$\Rightarrow \dfrac{PF}{FR} = \dfrac{3.6}{2.4} \\[1em] = \dfrac{36}{24} \\[1em] = \dfrac{3}{2}.$

Since, $\dfrac{PE}{EQ} \ne \dfrac{PF}{FR}$.

Hence, in this case EF is not parallel to QR.

(ii) Given,

PE = 4 cm, QE = 4.5 cm, PF = 8 cm and RF = 9 cm.

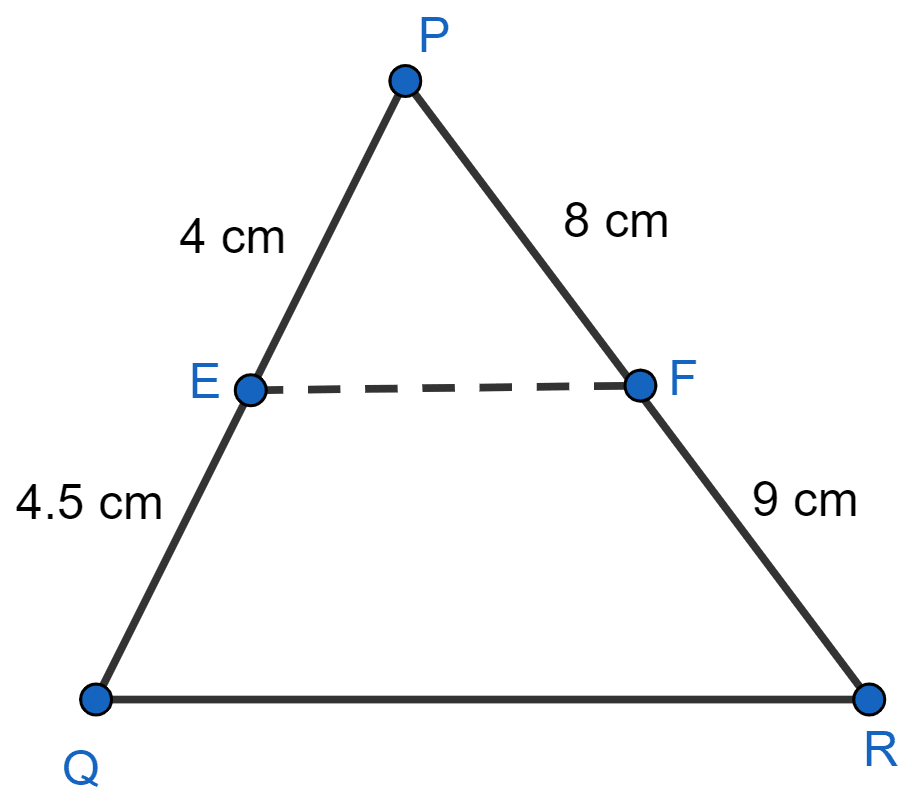
Substituting values in L.H.S. of equation (1), we get :

$\Rightarrow \dfrac{PE}{EQ} = \dfrac{4}{4.5} \\[1em] = \dfrac{40}{45} \\[1em] = \dfrac{8}{9}.$

Substituting values in R.H.S. of equation (1), we get :

$\Rightarrow \dfrac{PF}{FR} = \dfrac{8}{9}$

Since, $\dfrac{PE}{EQ} = \dfrac{PF}{FR}$.

Hence, in this case EF is parallel to QR.

(iii) Given,

PQ = 1.28 cm, PR = 2.56 cm, PE = 0.18 cm and PF = 0.36 cm.

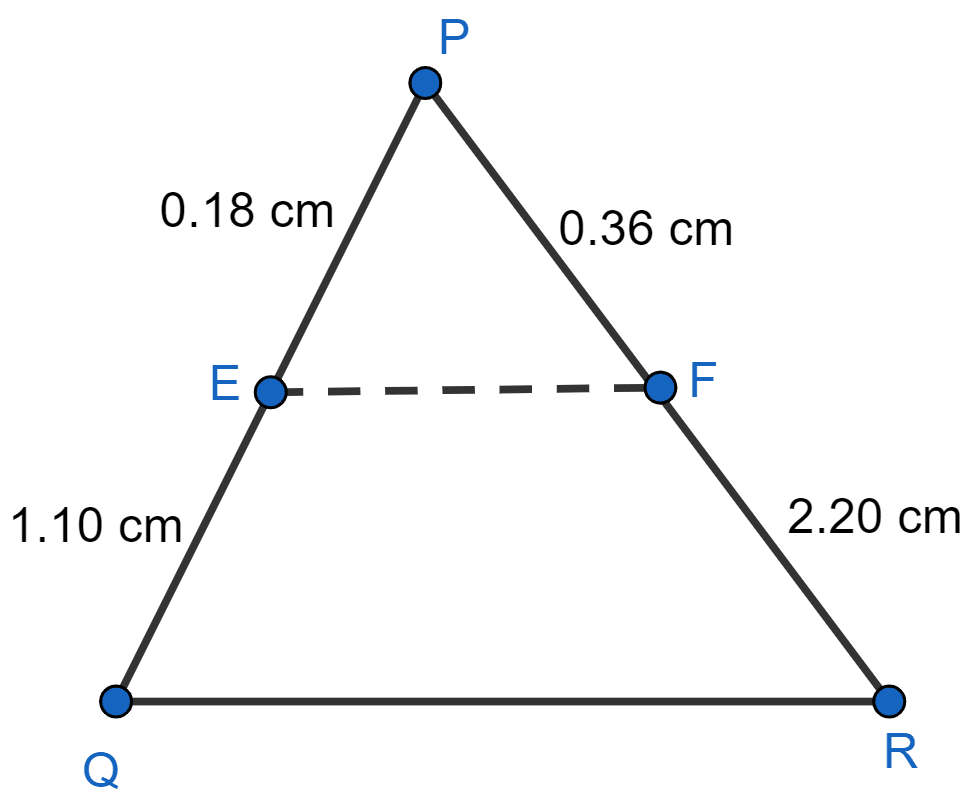
From figure,

⇒ EQ = PQ - PE = 1.28 - 0.18 = 1.10 cm

⇒ FR = PR - PF = 2.56 - 0.36 = 2.20 cm

Substituting values in L.H.S. of equation (1), we get :

$\Rightarrow \dfrac{PE}{EQ} = \dfrac{0.18}{1.10} \\[1em] = \dfrac{18}{110} \\[1em] = \dfrac{9}{55}.$

Substituting values in R.H.S. of equation (1), we get :

$\Rightarrow \dfrac{PF}{FR} = \dfrac{0.36}{2.20} \\[1em] = \dfrac{36}{220} \\[1em] = \dfrac{9}{55}.$

Since, $\dfrac{PE}{EQ} = \dfrac{PF}{FR}$.

Hence, in this case EF is parallel to QR.