Mathematics
Draw and describe the locus of a point in rhombus ABCD which is equidistant from AB and AD.
Locus
7 Likes
Answer
Let ABCD be a rhombus.
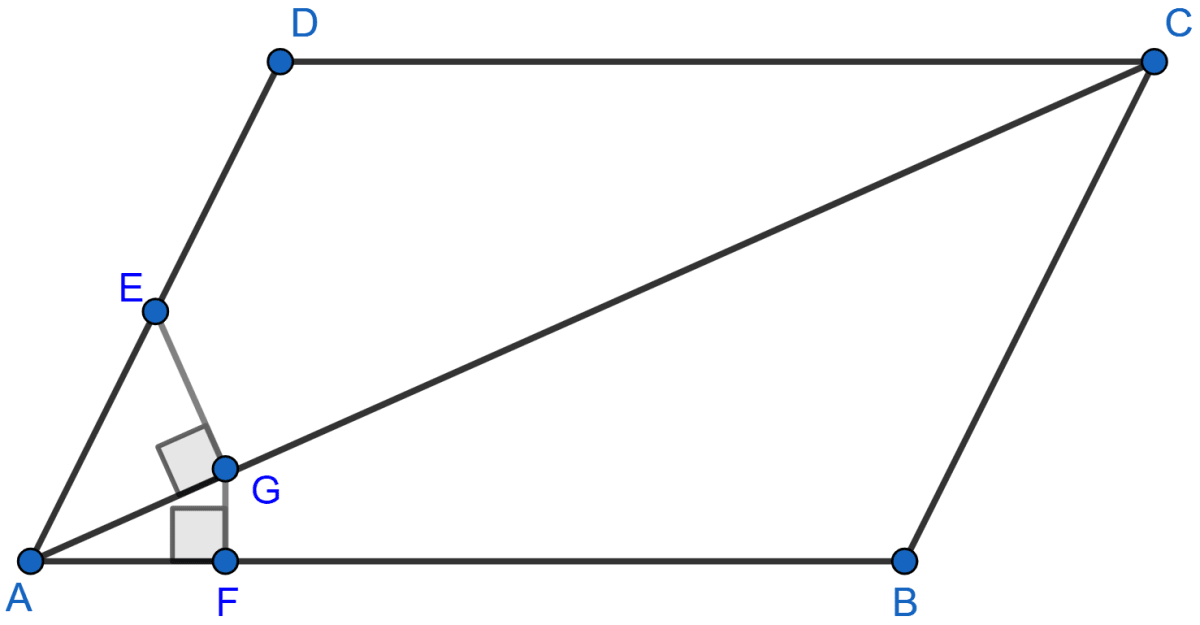
AC is diagonal of rhombus which will bisect angle A. As rhombus bisects the vertices angle.
∴ AC bisects ∠A
Let there be a point E and F on the sides AD and AB respectively. Draw a line perpendicular to AC from both the points.
Considering △AEG and △AFG
∠AGE = ∠AFG (Both are equal to 90°)
∠EAG = ∠FAG (Both are equal to half of ∠DAB.)
Hence, by AA axiom △AEG ~ △AFG.
Since triangles are similar so the ratio of their corresponding sides will be similar.
Since, distance from AD and AB is similar,
Hence, the locus of point is the diagonal AC of the rhombus ABCD.
Answered By
4 Likes
Related Questions
Draw and describe the locus of points inside a circle and equidistant from two fixed points on the circle.
Draw and describe the locus of centres of all circles passing through two fixed points.
Draw and describe the locus of a point in the rhombus ABCD which is equidistant from the points A and C.
Describe completely the locus of mid-point of radii of a circle.