Mathematics
Draw a line segment AB of length 7 cm. Construct the locus of a point P such that area of triangle PAB is 14 cm2.
Locus
11 Likes
Answer
Let the height of triangle PAB = h cm.
So, area of triangle = × base × height
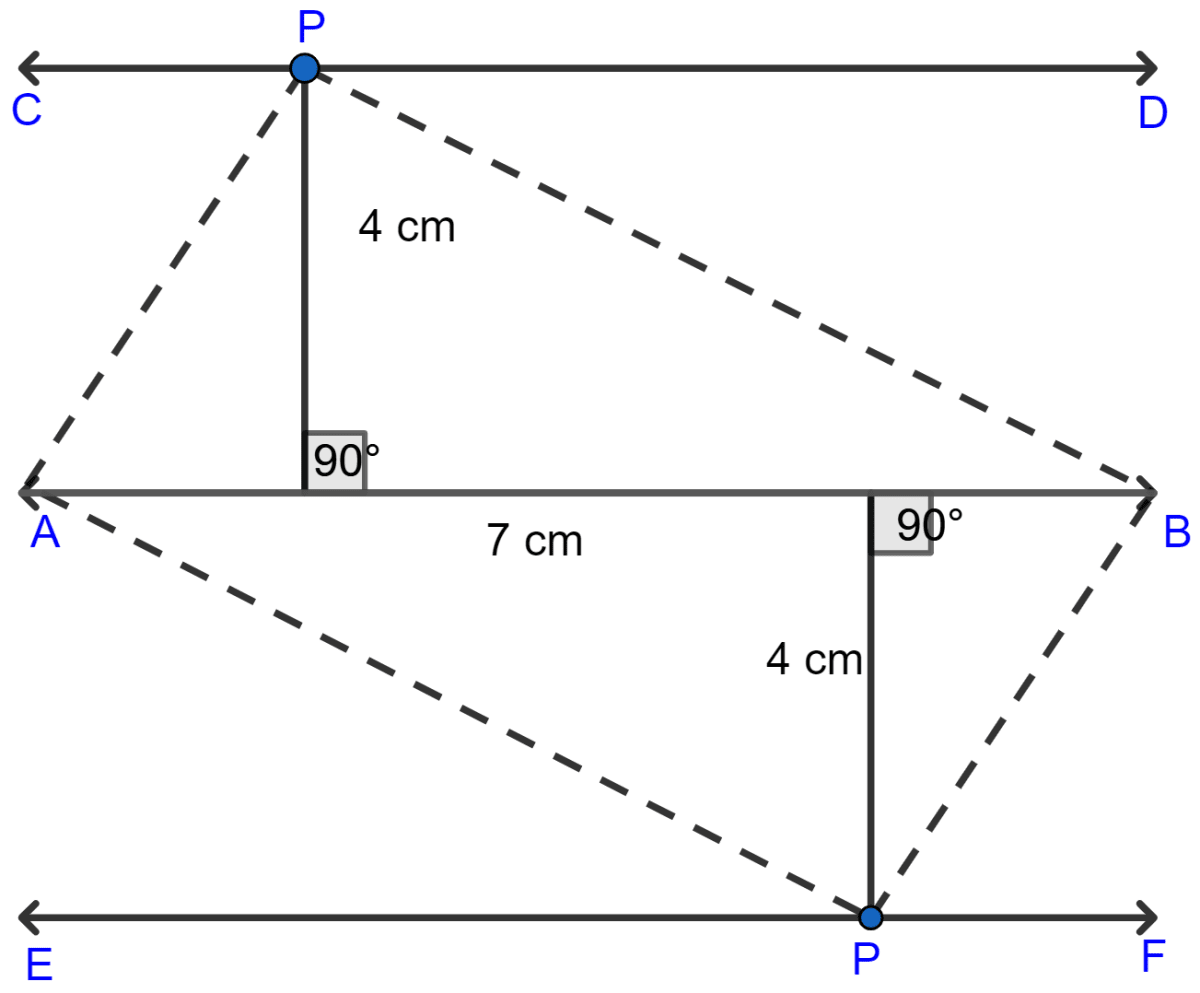
Hence, the locus of point P will be a pair of straight lines (CD and EF in figure) at a distance of 4 cm from AB.
Answered By
3 Likes
Related Questions
Draw a straight line AB of length 8cm. Draw the locus of all points which are equidistant from A and B. Prove your statement.
A point P is allowed to travel in space. State the locus of P so that it always remains at a constant distance from a fixed point C.
AB and CD are two intersecting lines. Find the position of a point which is at a distance of 2 cm from AB and 1.6 cm from CD.
Draw a line segment AB of length 12 cm. Mark M, the mid-point of AB. Draw and describe the locus of a point which is
(i) at a distance of 3 cm from AB.
(ii) at a distance of 5 cm from the point M.
Mark the points P, Q, R, S which satisfy both the above conditions. What kind of quadrilateral is PQRS ? Compute the area of quadrilateral PQRS.