Mathematics
Draw a histogram for the following distribution :
| Wt. (in kg) | No. of students |
|---|---|
| 40 - 44 | 2 |
| 45 - 49 | 8 |
| 50 - 54 | 12 |
| 55 - 59 | 10 |
| 60 - 64 | 6 |
| 65 - 69 | 4 |
Hence, estimate the modal weight.
Measures of Central Tendency
70 Likes
Answer
Steps :
- The given frequency distribution is discontinuous, to convert it into continuous distribution,
Adjustment factor = = 0.5
We construct the continuous frequency table for the given data :
| Classes before adjustment | Classes after adjustment | No. of students |
|---|---|---|
| 40 - 44 | 39.5 - 44.5 | 2 |
| 45 - 49 | 44.5 - 49.5 | 8 |
| 50 - 54 | 49.5 - 54.5 | 12 |
| 55 - 59 | 54.5 - 59.5 | 10 |
| 60 - 64 | 59.5 - 64.5 | 6 |
| 65 - 69 | 64.5 - 69.5 | 4 |
Take 2 cm along x-axis = 5 kg and 1 cm along y-axis = 2 (students).
Since, the scale on x-axis starts at 39.5, a break (zig-zag curve) is shown near the origin along x-axis to indicate that the graph is drawn to scale beginning at 39.5 and not at origin itself.
Construct rectangles corresponding to the given data.
In highest rectangle, draw two st. lines AC and BD from corners of the rectangles on either side of the highest rectangle to the opposite corners of the highest rectangle. Let P be the point of intersection of AC and BD.
Through P, draw a vertical line to meet the x-axis at M. The abscissa of the point M represents 52.75 kg.
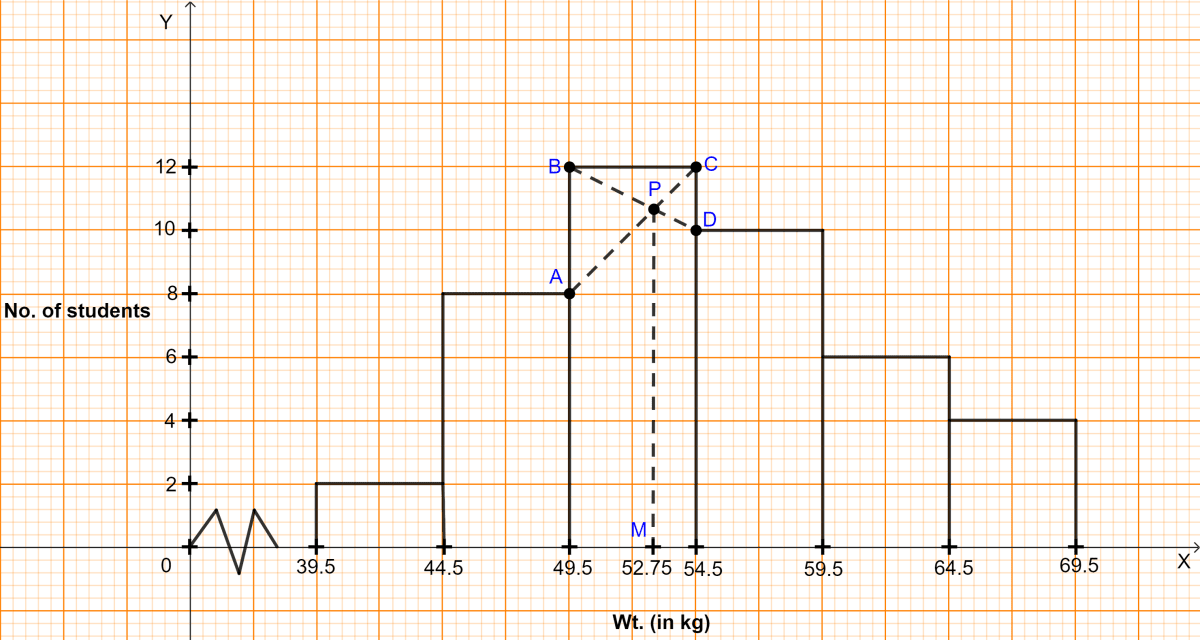
Hence, the required mode = 52.75 kg.
Answered By
19 Likes
Related Questions
Using a graph paper, draw a histogram for the given distribution showing the number of runs scored by 50 batsmen. Estimate the mode of the data :
Runs scored No. of batsmen 3000 - 4000 4 4000 - 5000 18 5000 - 6000 9 6000 - 7000 6 7000 - 8000 7 8000 - 9000 2 9000 - 10000 4 Use a graph paper for this question. The daily pocket expenses of 200 students in a school are given below :
Pocket expenses
(in ₹)No. of students
(frequency)0 - 5 10 5 - 10 14 10 - 15 28 15 - 20 42 20 - 25 50 25 - 30 30 30 - 35 14 35 - 40 12 Draw a histogram representing the above distribution and estimate the mode from the graph.
Find the mode of the following distribution by drawing a histogram.
Mid value Frequency 12 20 18 12 24 8 30 24 36 16 42 8 48 12 Also state the modal class.
Draw an ogive for the following frequency distribution :
Height (in cm) No. of students 150 - 160 8 160 - 170 3 170 - 180 4 180 - 190 10 190 - 200 2